The solution of |4x + 9| = 3 is?
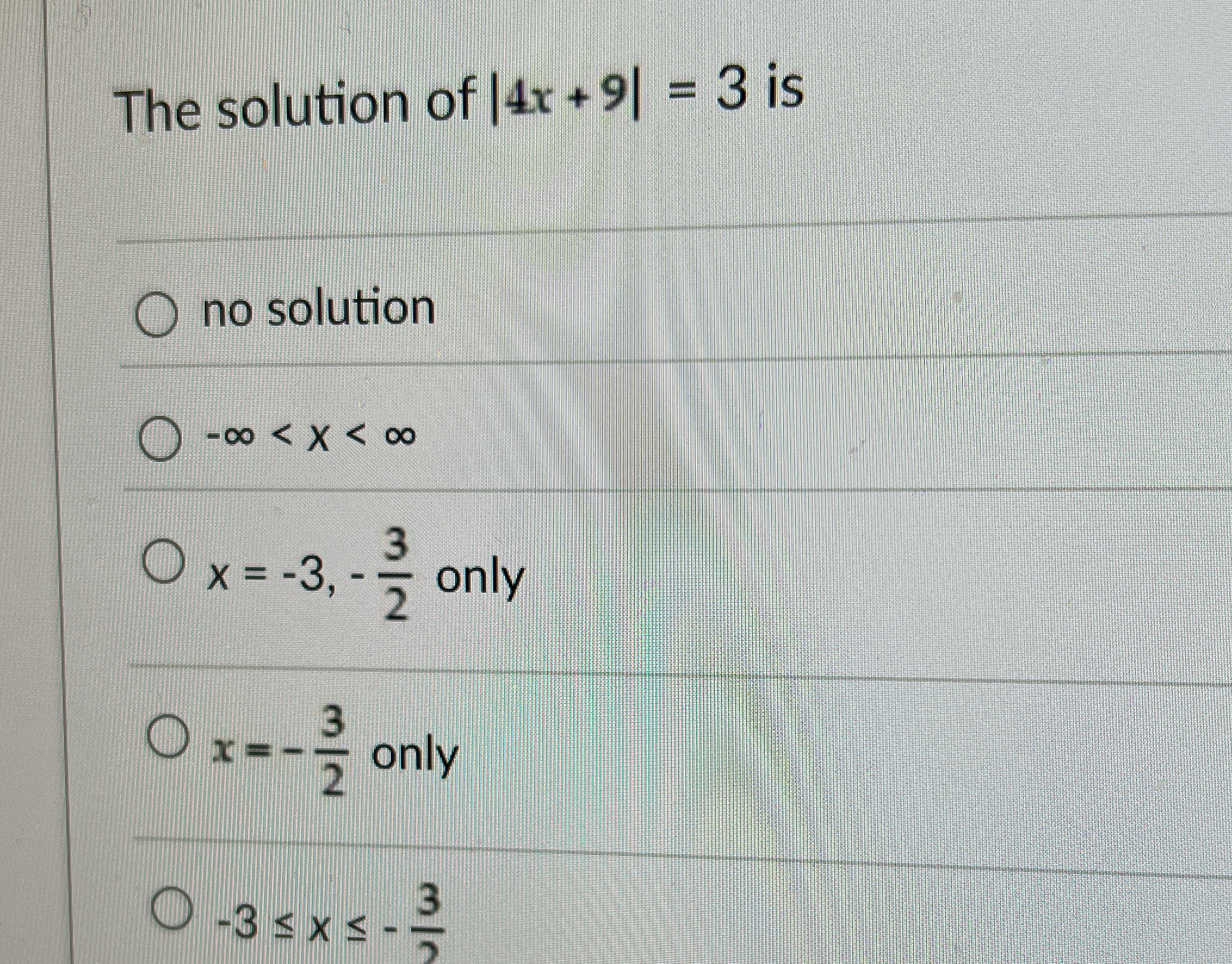
Understand the Problem
The question is asking to find the solution for the equation involving an absolute value, |4x + 9| = 3, and to identify the possible values of x that satisfy this equation.
Answer
The solutions are $x = -3$ and $x = -\frac{3}{2}$.
Answer for screen readers
The solutions are $x = -3$ and $x = -\frac{3}{2}$.
Steps to Solve
- Set up the absolute value equations
To solve the equation $|4x + 9| = 3$, we need to set up two separate equations based on the definition of absolute value.
The two cases to consider are: $$4x + 9 = 3$$ $$4x + 9 = -3$$
- Solve the first equation
Now, solve the first equation: $$4x + 9 = 3$$
Subtract 9 from both sides: $$4x = 3 - 9$$ $$4x = -6$$
Divide by 4: $$x = -\frac{6}{4} = -\frac{3}{2}$$
- Solve the second equation
Next, solve the second equation: $$4x + 9 = -3$$
Subtract 9 from both sides: $$4x = -3 - 9$$ $$4x = -12$$
Divide by 4: $$x = -\frac{12}{4} = -3$$
- List the solutions
The solutions from both equations are: $$x = -\frac{3}{2}$$ and $$x = -3$$.
Therefore, the final answer includes both solutions.
The solutions are $x = -3$ and $x = -\frac{3}{2}$.
More Information
In absolute value equations, the expression can equal either the positive or negative value, leading to two potential solutions.
Tips
- Forgetting to consider both cases of the absolute value.
- Failing to simplify fractions correctly.
AI-generated content may contain errors. Please verify critical information