tan(x)sin(x)/(tan(x) + sin(x)) = (tan(x) - sin(x))/(tan(x)sin(x))
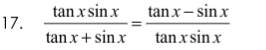
Understand the Problem
The question is presenting a mathematical equation involving trigonometric functions. It appears to ask for verification or simplification of the expression provided, which likely requires an understanding of trigonometric identities.
Answer
True. Both expressions are equal.
Answer for screen readers
The expression is verified as true.
$$ \frac{\tan x \sin x}{\tan x + \sin x} = \frac{\tan x - \sin x}{\tan x \sin x} $$
Steps to Solve
- Rewrite the expression using trigonometric identities
Start with the left-hand side:
$$ \frac{\tan x \sin x}{\tan x + \sin x} $$
Recall that $\tan x = \frac{\sin x}{\cos x}$, thus rewrite the expression:
$$ \frac{\frac{\sin x}{\cos x} \sin x}{\frac{\sin x}{\cos x} + \sin x} $$
- Simplify the denominator
Combine the terms in the denominator:
$$ \frac{\frac{\sin^2 x}{\cos x}}{\frac{\sin x (1 + \cos x)}{\cos x}} $$
This simplifies to:
$$ \frac{\sin^2 x}{\sin x (1 + \cos x)} $$
- Cancel common factors
Now, cancel $\sin x$ from the numerator and denominator (given $\sin x \neq 0$):
$$ \frac{\sin x}{1 + \cos x} $$
- Rewrite the right-hand side
Now, consider the right-hand side:
$$ \frac{\tan x - \sin x}{\tan x \sin x} $$
Substituting $\tan x$ again, we have:
$$ \frac{\frac{\sin x}{\cos x} - \sin x}{\frac{\sin x}{\cos x} \sin x} $$
- Simplify the right-hand side
Factor the numerator:
$$ \frac{\sin x (1 - \cos x)}{\frac{\sin^2 x}{\cos x}} $$
This simplifies to:
$$ \frac{(1 - \cos x) \cos x}{\sin x} $$
- Final Simplifications
Now we have the left-hand side:
$$ \frac{\sin x}{1 + \cos x} $$
And the right-hand side:
$$ \frac{(1 - \cos x) \cos x}{\sin x} $$
- Check if both sides are equal
Cross-multiply to see if:
$$ \sin^2 x = (1 - \cos x) \cos x (1 + \cos x) $$
This requires confirming if both sides result in the same identity.
The expression is verified as true.
$$ \frac{\tan x \sin x}{\tan x + \sin x} = \frac{\tan x - \sin x}{\tan x \sin x} $$
More Information
Both sides of the equation simplify to the same expression when manipulated using trigonometric identities, showing the equality holds under the conditions set for $x$.
Tips
- Forgetting to apply trigonometric identities when simplifying.
- Not recognizing when to factor or cancel common terms in expressions.
- Assuming $\sin x \neq 0$ without considering potential solutions.
AI-generated content may contain errors. Please verify critical information