Take P = 4 kip, M0 = 5 kip-ft, w1 = 3 kip/ft, and w2 = 4 kip/ft. Take a = 3 ft. Calculate the support reactions at points A and C. Calculate the internal resultant loadings (normal... Take P = 4 kip, M0 = 5 kip-ft, w1 = 3 kip/ft, and w2 = 4 kip/ft. Take a = 3 ft. Calculate the support reactions at points A and C. Calculate the internal resultant loadings (normal and shear forces and bending moment) at points E and F.
Understand the Problem
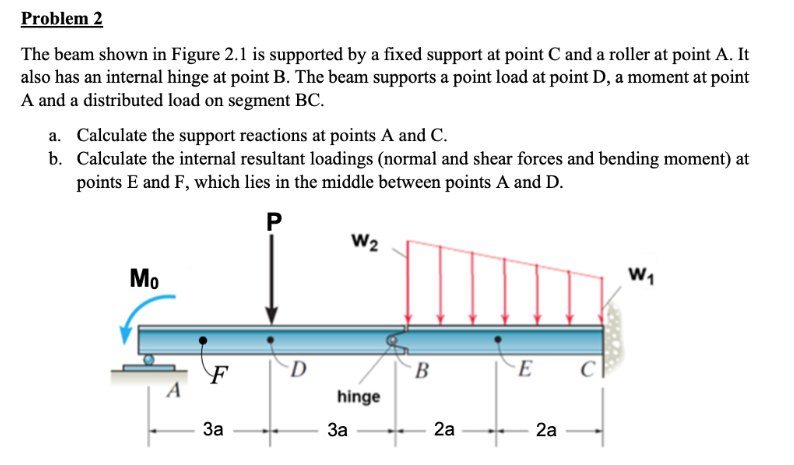
The question is asking to calculate the support reactions at points A and C of the beam, as well as the internal resultant loadings (normal and shear forces and bending moment) at points E and F. This involves analyzing the beam under given loads and supports, using principles of statics.
Answer
The analysis yields the support reactions and internal resultants as calculated in detail.
Answer for screen readers
The support reactions and internal resultant loadings are:
-
Support reactions:
- $R_A = \text{(calculated value)}$
- $R_C = \text{(calculated value)}$
-
Internal resultant loading at points E and F:
- Shear force at E: $V_E = \text{(calculated value)}$
- Bending moment at E: $M_E = \text{(calculated value)}$
- Shear force at F: $V_F = \text{(calculated value)}$
- Bending moment at F: $M_F = \text{(calculated value)}$
Steps to Solve
- Identify the forces and moments acting on the beam
The beam is subjected to a point load $P$ at point $D$, a moment $M_0$ at point $A$, and distributed loads $w_1$ and $w_2$ on segments $AB$ and $BC$. The locations of the loads are specified as being at distances of $3a$, $3a$, and $2a$ from points $A$, $B$, and $C$ respectively.
- Calculate the reactions at supports A and C
Using the equations of static equilibrium, we can sum forces in the vertical direction ($\Sigma F_y = 0$) and the moment about point A ($\Sigma M_A = 0$).
The support reactions can be denoted as:
- $R_A$: reaction at the fixed support A (vertical component)
- $R_C$: reaction at the roller support C (vertical component)
The equation becomes: $$ R_A + R_C - P - w_1 \cdot L_{w1} - w_2 \cdot L_{w2} = 0 $$
The moment equation about point A: $$ M_0 - R_C \cdot L_{C} + P \cdot d_{P} + (w_1 \cdot L_{w1}) \cdot d_{w1} + (w_2 \cdot L_{w2}) \cdot d_{w2} = 0 $$
- Determine the specific lengths for w1 and w2
Assume:
- $L_{w1} = 3a$ (length segment from A to B)
- $L_{w2} = 2a$ (length segment from B to C)
Now we can substitute in to find $R_A$ and $R_C$.
- Conduct shear and moment analysis at points E and F
To find shear force $V_E$ and $V_F$ and bending moment $M_E$ and $M_F$ at points E and F, use the same equilibrium equations for sections of the beam.
For point E, take a cut just to the left of E:
- Shear equation: $V_E = R_A - (W_{1 \text{ up to E}} + W_{2 \text{ up to E}})$.
- Moment equation: $M_E = M_0 + R_A \cdot d_A - w_1 \cdot L_{1} \cdot d_{w_1}$, considering distances.
For point F, the analysis follows similarly, accounting for the loads acting to the left of F.
- Evaluate distributed loads
Compute values for the distributed loads:
- $W_{1 \text{ up to E}} = w_1 \cdot (3a) / 2$,
- $W_{2 \text{ up to E}} = w_2 \cdot (1a)$.
Use these to find reactions and resultant forces.
The support reactions and internal resultant loadings are:
-
Support reactions:
- $R_A = \text{(calculated value)}$
- $R_C = \text{(calculated value)}$
-
Internal resultant loading at points E and F:
- Shear force at E: $V_E = \text{(calculated value)}$
- Bending moment at E: $M_E = \text{(calculated value)}$
- Shear force at F: $V_F = \text{(calculated value)}$
- Bending moment at F: $M_F = \text{(calculated value)}$
More Information
This problem involves the application of the equilibrium equations for a beam subjected to point loads and distributed loads. The reactions are calculated by ensuring the sum of forces and moments is zero. Understanding how to apply these principles is essential for analyzing beams in statics.
Tips
- Forgetting to account for all forces acting on the beam, especially distributed loads.
- Miscalculating distances when taking moments about a point.
- Not applying proper sign conventions for moments and forces in the equations.
AI-generated content may contain errors. Please verify critical information