Solve using substitution: -3x + y = 13 and 2x - y = -10.
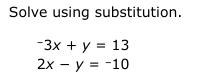
Understand the Problem
The question is asking to solve a system of linear equations using the substitution method. This involves expressing one variable in terms of the other from one equation and substituting that expression into the second equation.
Answer
The solution is $x = -3$ and $y = 4$.
Answer for screen readers
The solution to the system of equations is $x = -3$ and $y = 4$.
Steps to Solve
-
Isolate one variable Start from the first equation: $$ -3x + y = 13 $$ Rearranging the equation to solve for $y$ gives: $$ y = 3x + 13 $$
-
Substitute into the second equation Now substitute the expression for $y$ from the first equation into the second equation: $$ 2x - (3x + 13) = -10 $$
-
Simplify the substituted equation Distributing the negative sign: $$ 2x - 3x - 13 = -10 $$ This simplifies to: $$ -x - 13 = -10 $$
-
Solve for x Add 13 to both sides: $$ -x = 3 $$ Now multiply both sides by -1 to find $x$: $$ x = -3 $$
-
Substitute back to find y Now that we have $x = -3$, substitute it back into the equation for $y$: $$ y = 3(-3) + 13 $$ Calculating this gives: $$ y = -9 + 13 $$ So, $$ y = 4 $$
The solution to the system of equations is $x = -3$ and $y = 4$.
More Information
The solutions $(x, y) = (-3, 4)$ represent the point of intersection for the two lines described by the given equations. This point satisfies both equations simultaneously.
Tips
- Forgetting to distribute the negative sign correctly when substituting. Always double-check that all terms are accounted for.
- Not simplifying the equation completely before solving for the variable. Make sure to combine like terms.
AI-generated content may contain errors. Please verify critical information