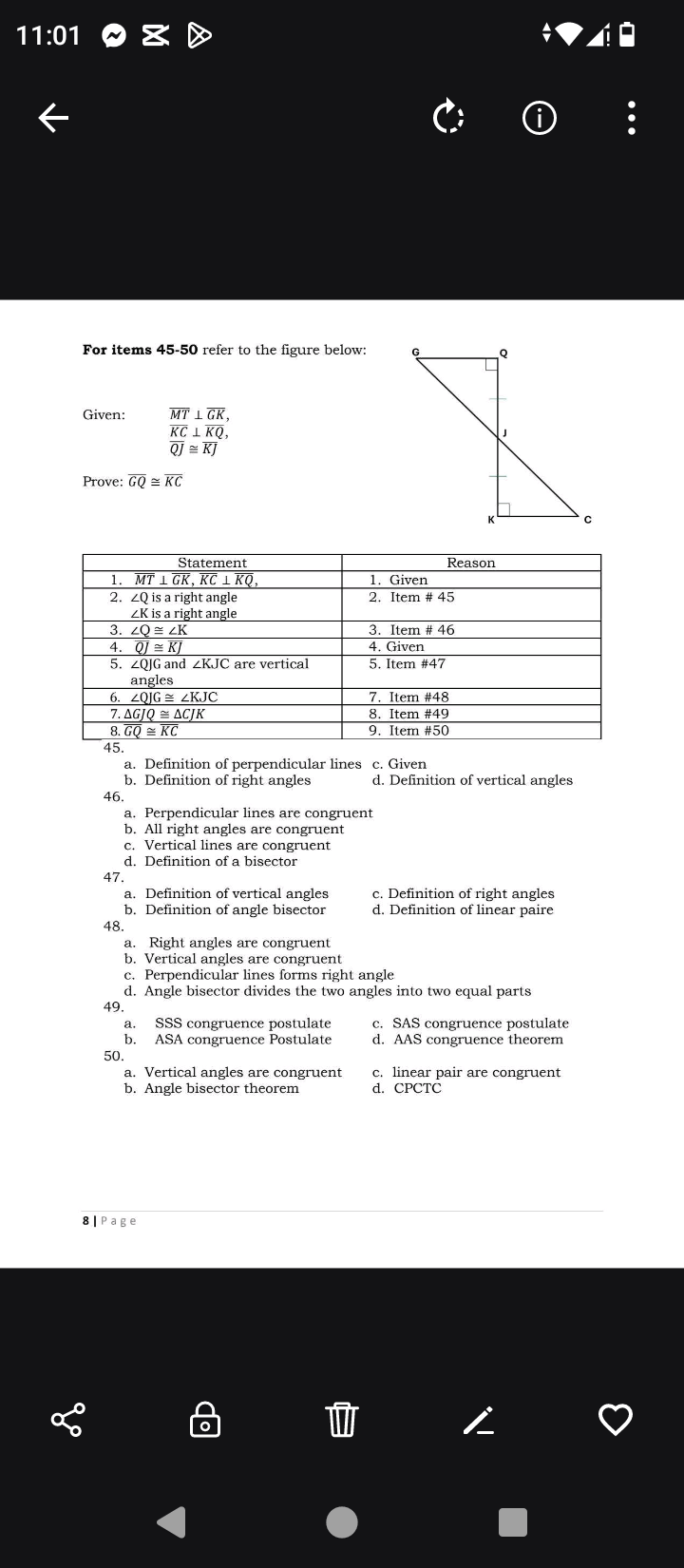
Solve the geometry proof questions 45-50 by choosing the correct definitions, postulates or theorems.

Steps to Solve

1. Identify Right Angles
   The statement mentions that $\overline{MT} \perp \overline{GK}$ and $\overline{KC} \perp \overline{KQ}$. According to the definition of perpendicular lines, this means that both $\angle Q$ and $\angle K$ are right angles.

• Hence, the answer to item 45 is (b) Definition of right angles.

2. State Congruence of Angles
   Since both angles $\angle Q$ and $\angle K$ are right angles, by the property of all right angles, we conclude that $\angle Q \cong \angle K$.

• Therefore, the answer to item 46 is (b) All right angles are congruent.

3. Vertical Angles
   The next step in the proof establishes that $\angle QJG$ and $\angle KJC$ are vertical angles, which can be derived from the intersection of lines.

• Thus, the answer to item 47 is (a) Definition of vertical angles.

4. Congruence of Vertical Angles
   Using the property that vertical angles are always congruent, we conclude that $\angle QJG \cong \angle KJC$.

• Therefore, the answer to item 48 is (b) Vertical angles are congruent.

5. Triangle Congruence
   For triangles $\triangle GJQ$ and $\triangle CJK$, the criteria for congruence can be applied. Here, we use the Side-Angle-Side (SAS) postulate since two sides and the included angle of both triangles are congruent.

• Thus, the answer for item 49 is (c) SAS congruence postulate.

6. Conclude with CPCTC
   Finally, using the Corresponding Parts of Congruent Triangles are Congruent (CPCTC) theorem, we conclude that $\overline{GQ} \cong \overline{KC}$.

• Therefore, the answer for item 50 is (d) CPCTC.