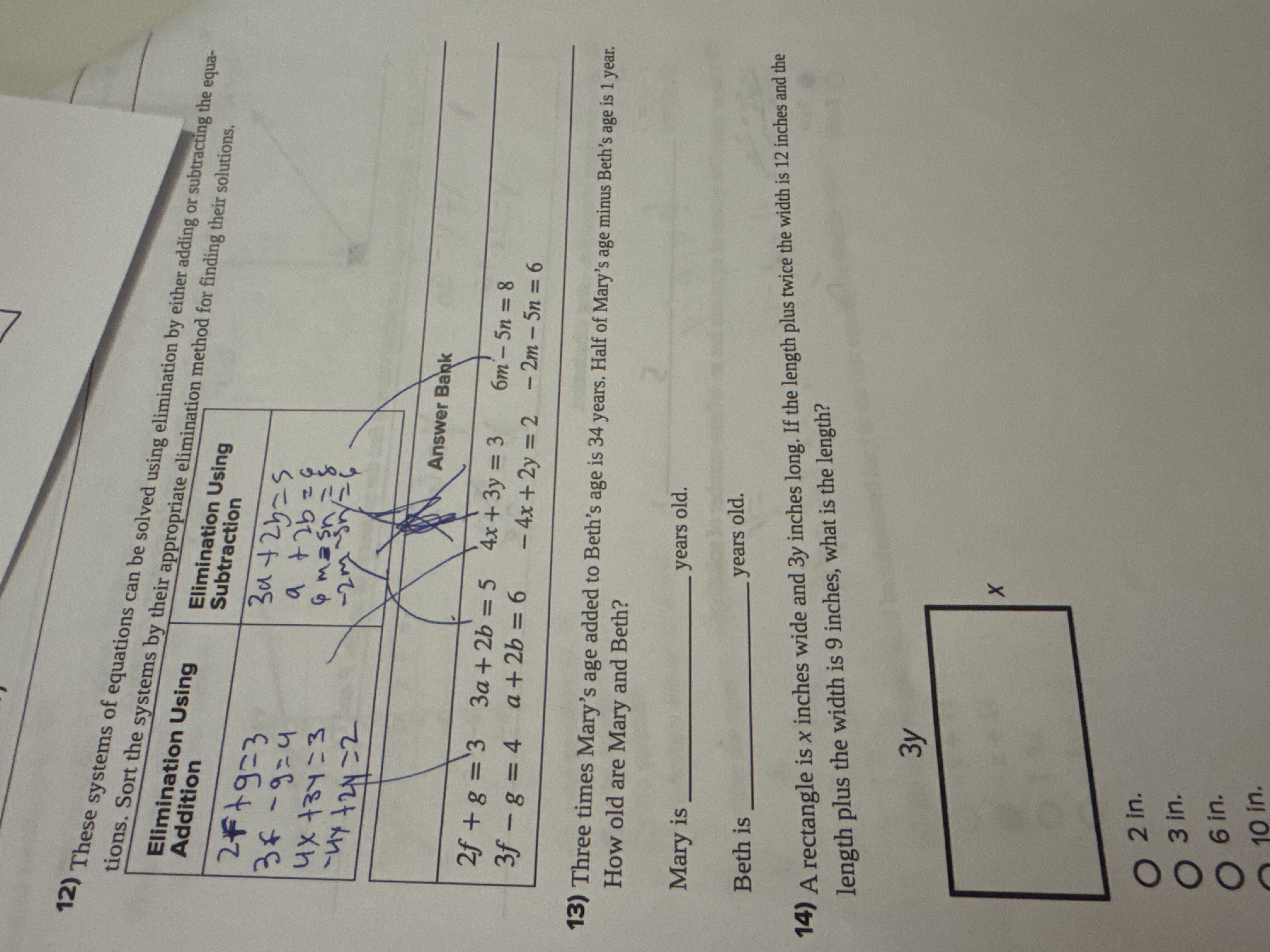
Solve the following math problems: 1) Sort the systems of equations by whether they are solved using addition or subtraction. 2) Three times Mary's age added to Beth's age is 34... Solve the following math problems: 1) Sort the systems of equations by whether they are solved using addition or subtraction. 2) Three times Mary's age added to Beth's age is 34 years. Half of Mary's age minus Beth's age is 1 year. How old are Mary and Beth? 3) A rectangle is x inches wide and 3y inches long. If the length plus twice the width is 12 inches and the length plus the width is 9 inches, what is the length? Read more

Steps to Solve

1. Problem 12: Identify systems for addition

   We need to find systems of equations where adding the equations together will eliminate one of the variables. This happens when the coefficients of one of the variables are opposites (e.g., $g$ and $-g$). The system that fits this is: $2f + g = 3$ $3f - g = 4$

   Because adding these eliminates $g$.

2. Problem 12: Identify systems for subtraction

   We need to find systems of equations where subtracting the equations will eliminate one of the variables. This happens when the coefficients of one of the variables are the same (e.g., $2b$ and $2b$). The system that fits this is: $3a + 2b = 5$ $a + 2b = 6$

   Because subtracting these eliminates $2b$.

   And

   $6m - 5n = 8$ $-2m - 5n = 6$

   Because subtracting these eliminates $5n$.

3. Problem 12: Identify systems for neither addition nor subtraction

   We need to find systems of equations where neither adding nor subtracting the equations will eliminate one of the variables. The system that fits this is: $4x + 3y = 3$ $-4x + 2y = 2$

   This system requires multiplying one or both equations by a constant to make the coefficients match or be opposites. However, adding this system also works since the coefficients of $x$ are opposites, so it can be solved using addition.

   There may have been a typo in the original document.

4. Problem 13: Set up equations for Mary and Beth's ages

   Let $M$ be Mary's age and $B$ be Beth's age. Translate the word problem into two equations: $3M + B = 34$ $\frac{1}{2}M - B = 1$

5. Problem 13: Solve for M

   Add the two equations to eliminate $B$: $(3M + B) + (\frac{1}{2}M - B) = 34 + 1$ $3M + \frac{1}{2}M = 35$ $\frac{6}{2}M + \frac{1}{2}M = 35$ $\frac{7}{2}M = 35$ $M = 35 \cdot \frac{2}{7}$ $M = 5 \cdot 2$ $M = 10$

6. Problem 13: Solve for B

   Substitute $M = 10$ into either equation. Let's use the first equation: $3M + B = 34$ $3(10) + B = 34$ $30 + B = 34$ $B = 34 - 30$ $B = 4$

7. Problem 14: Set up the equations for the rectangle

   Let $x$ be the width and $3y$ be the length. $3y + 2x = 12$ $3y + x = 9$

8. Problem 14: Solve for x

   Subtract the second equation from the first to eliminate $3y$: $(3y + 2x) - (3y + x) = 12 - 9$ $3y + 2x - 3y - x = 3$ $x = 3$

9. Problem 14: The question asks for the length, but solves for the width

   The question asks for the length, the solution we came to calculates the width