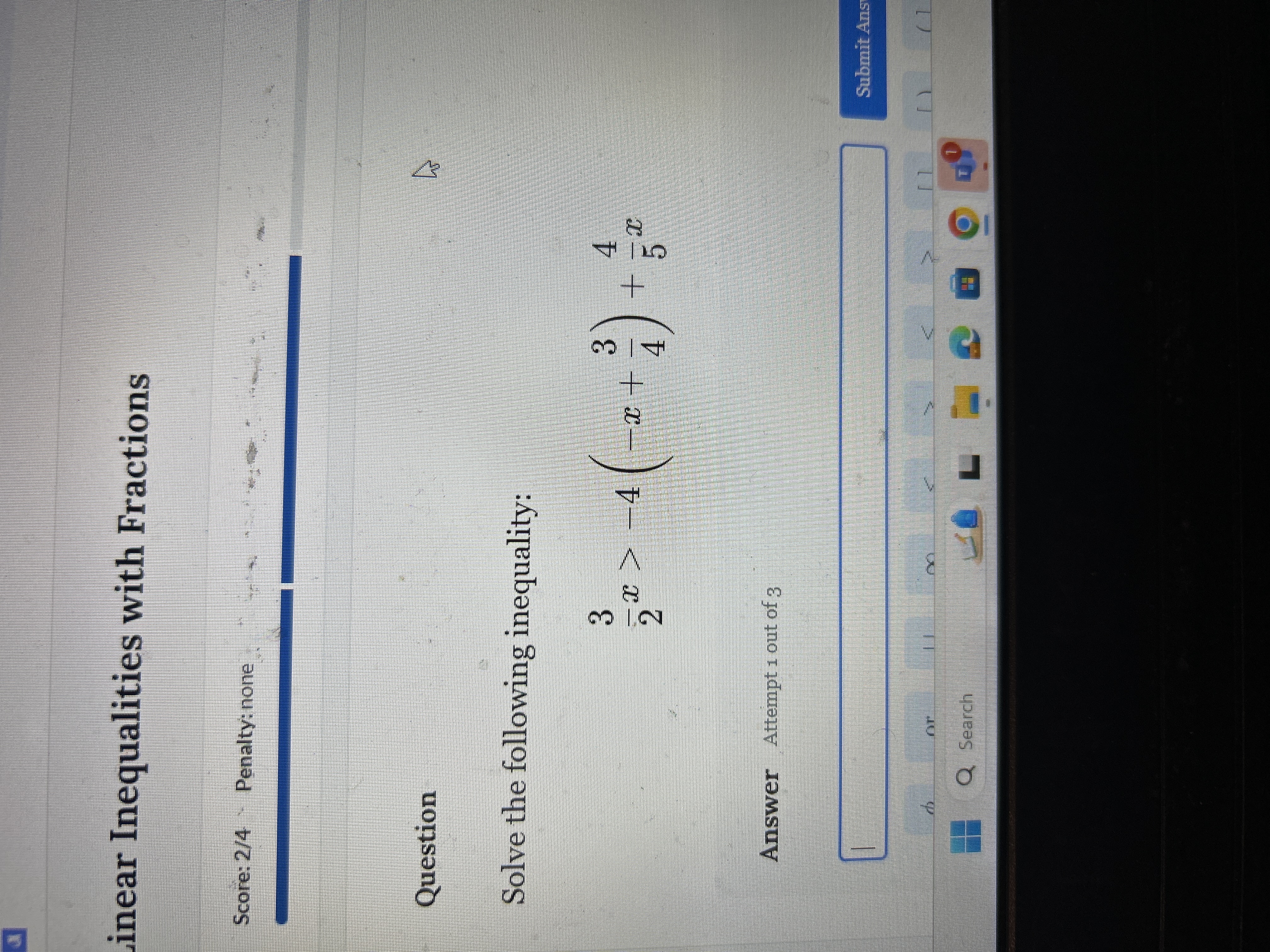
Solve the following inequality: \( \frac{3}{2}x > -4 \left( x + \frac{3}{4} \right) + \frac{4}{5}x \),
Understand the Problem
The question is asking us to solve the given linear inequality involving fractions. We need to find the values of x that satisfy the inequality provided in the question.
Answer
$$ x > \frac{-30}{47} $$
Answer for screen readers
The solution to the inequality is:
$$ x > \frac{-30}{47} $$
Steps to Solve
- Distribute the terms on the right side
Start by distributing (-4) on the right side of the inequality.
[ -4(x + \frac{3}{4}) = -4x - 3 ]
So the inequality becomes:
[ \frac{3}{2}x > -4x - 3 + \frac{4}{5}x ]
- Combine like terms
Next, combine all the (x) terms on the right side:
[ -4x + \frac{4}{5}x = -\frac{20}{5}x + \frac{4}{5}x = -\frac{16}{5}x ]
Substituting this back in, we have:
[ \frac{3}{2}x > -\frac{16}{5}x - 3 ]
- Get all (x) terms on one side
Add (\frac{16}{5}x) to both sides:
[ \frac{3}{2}x + \frac{16}{5}x > -3 ]
- Find a common denominator and simplify
The common denominator of 2 and 5 is 10. Rewrite the (x) terms:
[ \frac{15}{10}x + \frac{32}{10}x > -3 ]
This gives:
[ \frac{47}{10}x > -3 ]
- Isolate (x)
Multiply both sides by (\frac{10}{47}) (since it's positive, the inequality stays the same):
[ x > -3 \cdot \frac{10}{47} ]
- Calculate the right side
Calculating this gives:
[ x > \frac{-30}{47} ]
The solution to the inequality is:
$$ x > \frac{-30}{47} $$
More Information
This result indicates that (x) can take any value greater than (-\frac{30}{47}), which is approximately (-0.6383). It's useful to remember that inequalities can describe a range of values rather than a single solution.
Tips
- Failing to distribute correctly when there are parentheses involved.
- Not combining like terms correctly can lead to errors in solving the inequality.
- Forgetting to reverse the inequality sign when multiplying by a negative number (not applicable here since all multiplications were by positive numbers).
AI-generated content may contain errors. Please verify critical information