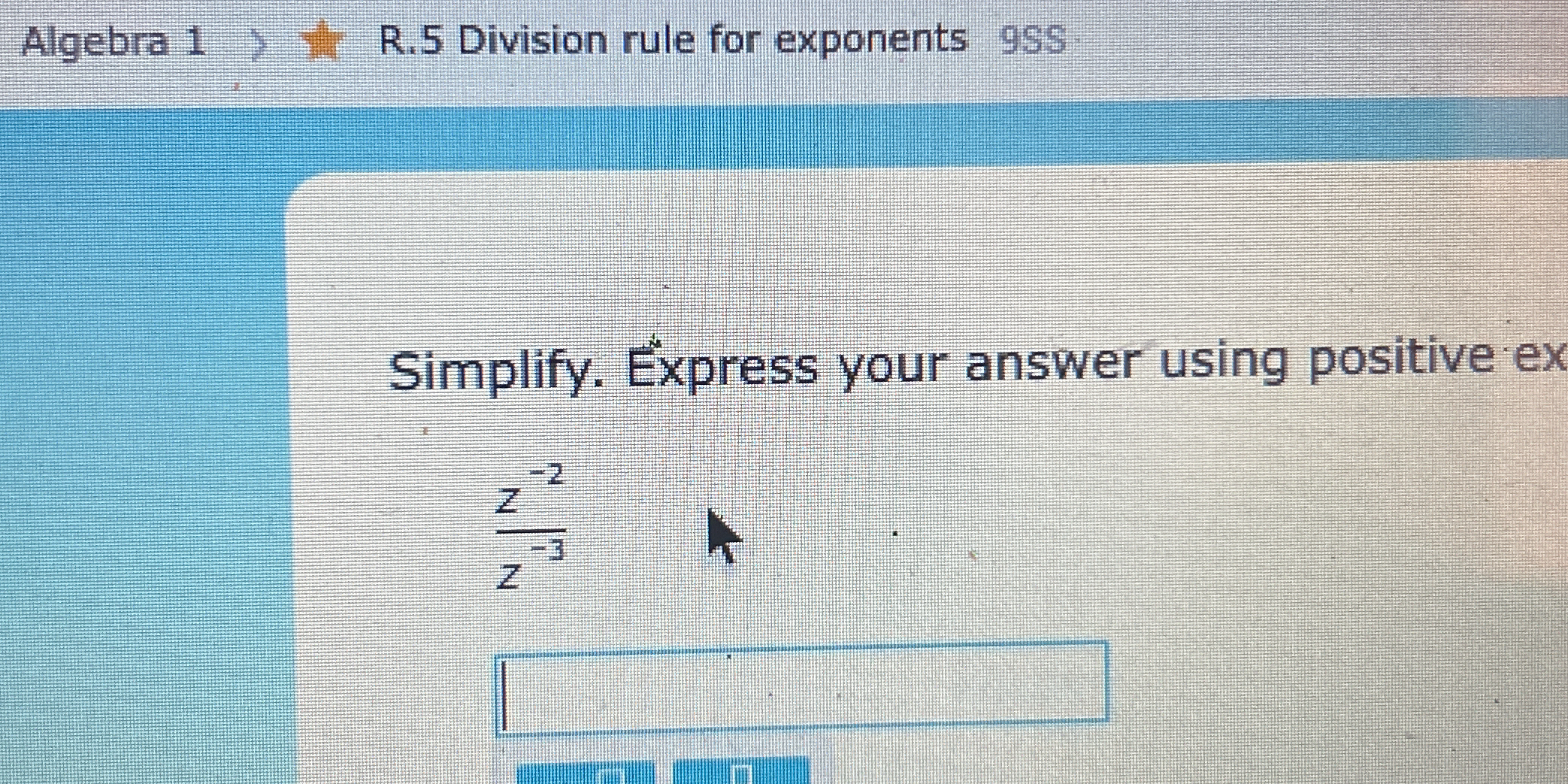
Simplify. Express your answer using positive exponents: z^{-2}/z^{-3}
Understand the Problem
The question is asking to simplify the expression involving exponents, specifically z raised to negative powers. We need to apply the rules of exponents to express the answer in a positive exponent form.
Answer
The simplified expression is \( z \).
Answer for screen readers
The simplified expression is ( z ).
Steps to Solve
- Use the Division Rule for Exponents
When dividing exponential expressions with the same base, subtract the exponent in the denominator from the exponent in the numerator. Here, we have:
$$ \frac{z^{-2}}{z^{-3}} = z^{-2 - (-3)} $$
- Simplify the Exponent
Now, simplify the exponent:
$$ -2 - (-3) = -2 + 3 = 1 $$
- Write as a Positive Exponent
Now rewrite the expression with the simplified exponent:
$$ z^{1} = z $$
The simplified expression is ( z ).
More Information
When working with negative exponents, remember that ( z^{-n} = \frac{1}{z^n} ). Therefore, dividing terms with negative exponents can be simplified by applying the exponent rules before rewriting them in terms of positive exponents.
Tips
- Forgetting to subtract correctly when applying the division rule for exponents.
- Confusing negative exponents with their positive equivalents.
AI-generated content may contain errors. Please verify critical information