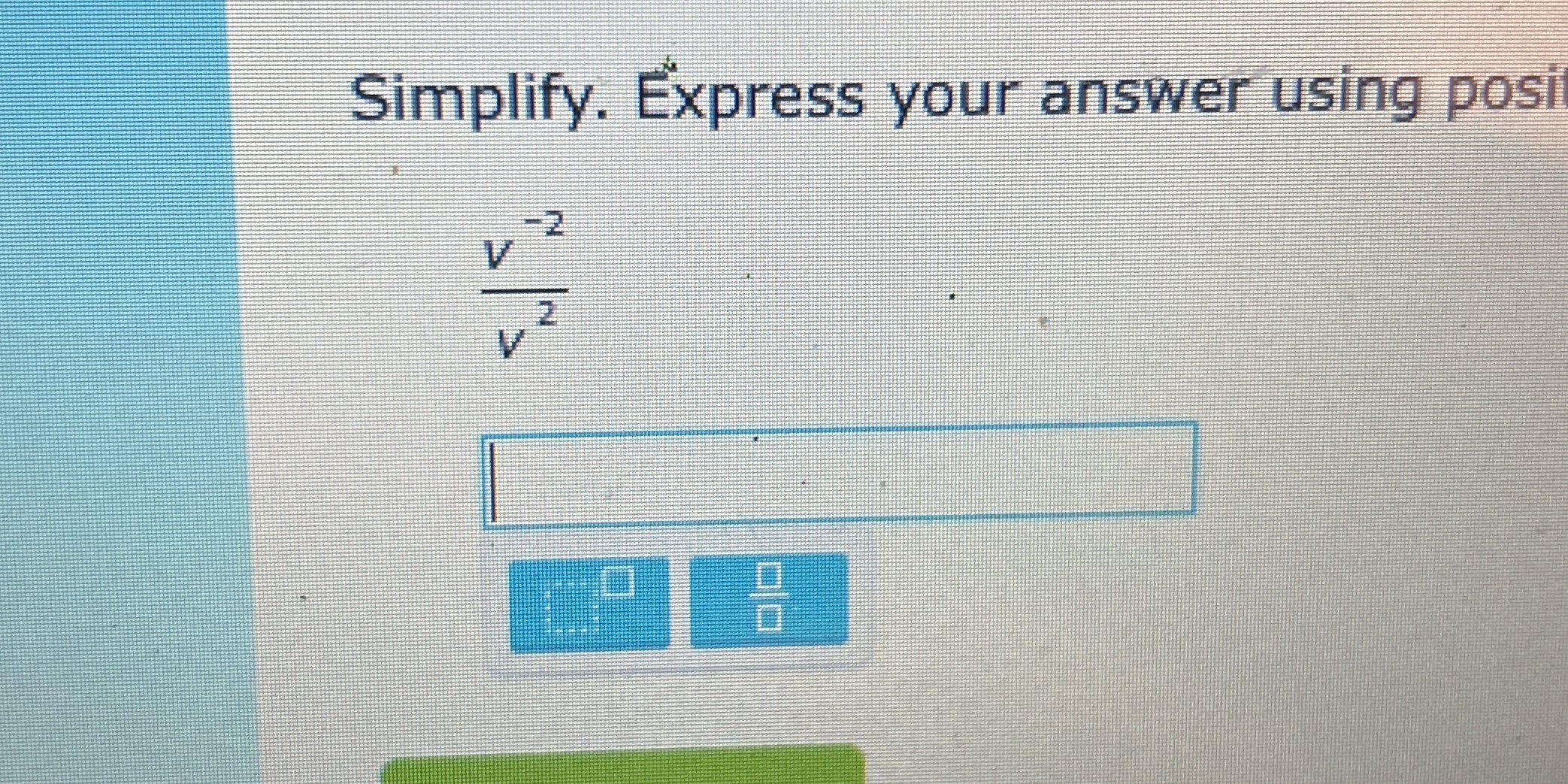
Simplify. Express your answer using positive exponents: v^(-2) / v^2
Understand the Problem
The question is asking to simplify the expression given, which is a fraction with a variable in the numerator and the denominator, specifically involving square roots and negative exponents.
Answer
$$ \frac{1}{v^4} $$
Answer for screen readers
The simplified expression is:
$$ \frac{1}{v^4} $$
Steps to Solve
-
Write Down the Expression
The given expression is:
$$ \frac{v^{-2}}{v^{2}} $$ -
Apply the Quotient Rule
Using the property of exponents that states $\frac{a^m}{a^n} = a^{m-n}$, we can simplify the fraction:
$$ v^{-2 - 2} = v^{-4} $$ -
Convert to Positive Exponents
To express $v^{-4}$ using positive exponents, we can use the rule that $a^{-n} = \frac{1}{a^n}$. Thus, we have:
$$ v^{-4} = \frac{1}{v^4} $$
The simplified expression is:
$$ \frac{1}{v^4} $$
More Information
When simplifying expressions involving exponents, it's important to remember the rules of exponents, especially for dealing with negative and zero exponents.
Tips
- Ignoring the Quotient Rule: Some may forget to subtract the exponents correctly when applying the quotient rule.
- Not Converting: Remembering to express the final answer using positive exponents can sometimes be overlooked.
AI-generated content may contain errors. Please verify critical information