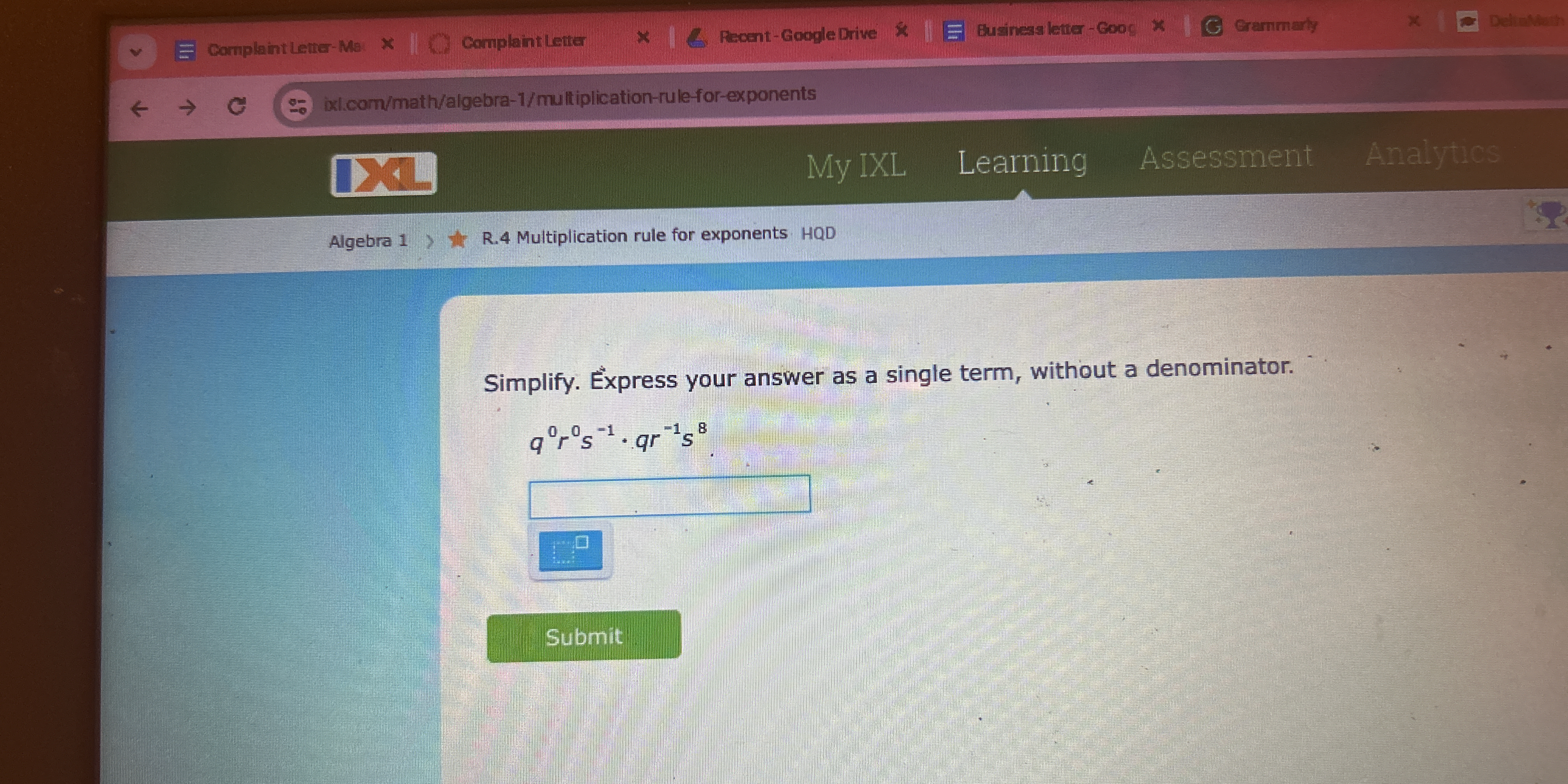
Simplify. Express your answer as a single term, without a denominator: q^0 * r^(-1) * s^(-1) * q^r^(-1) * s^(8)
Understand the Problem
The question is asking to simplify the expression involving exponents and express the answer as a single term without a denominator. The expression includes variables raised to different powers.
Answer
The simplified expression is \( \frac{q}{r^9 s^9} \).
Answer for screen readers
The simplified expression is ( \frac{q}{r^9 s^9} ).
Steps to Solve
-
Identify the Expression
The given expression is (q^0 r^{-1} s^{-1} \cdot q^r s^{-8}).
-
Use the Exponent Rule for Multiplication
According to the exponent multiplication rule, if you multiply two terms with the same base, you add their exponents.
-
For (q): $$ q^0 \cdot q = q^{0 + 1} = q^1 $$
-
For (r): $$ r^{-1} \cdot r^{-8} = r^{-1 + (-8)} = r^{-9} $$
-
For (s): $$ s^{-1} \cdot s^{-8} = s^{-1 + (-8)} = s^{-9} $$
-
-
Combine the Results
Putting it all together, we get: $$ q^1 r^{-9} s^{-9} = q \cdot r^{-9} \cdot s^{-9} $$
-
Express as a Single Term Without Denominator
To rewrite the expression without negative exponents: $$ q \cdot \frac{1}{r^9} \cdot \frac{1}{s^9} = \frac{q}{r^9 s^9} $$
However, this has a denominator. We can express the entire term using the reciprocal: $$ q \cdot r^{-9} \cdot s^{-9} = \frac{q}{r^9 s^9} $$
The simplified expression is ( \frac{q}{r^9 s^9} ).
More Information
The expression originally contained coefficients with both positive and negative exponents. Understanding how to manipulate exponents through addition can simplify complex expressions, making calculations easier.
Tips
- Forgetting the rule for negative exponents; remember that $a^{-n} = \frac{1}{a^n}$.
- Neglecting to combine like terms properly; ensure you add the exponents correctly.
AI-generated content may contain errors. Please verify critical information