Show that the area of R is given by the integral ∫(from 0 to 2) of (1/((t+1)(t+2))) dt.
Understand the Problem
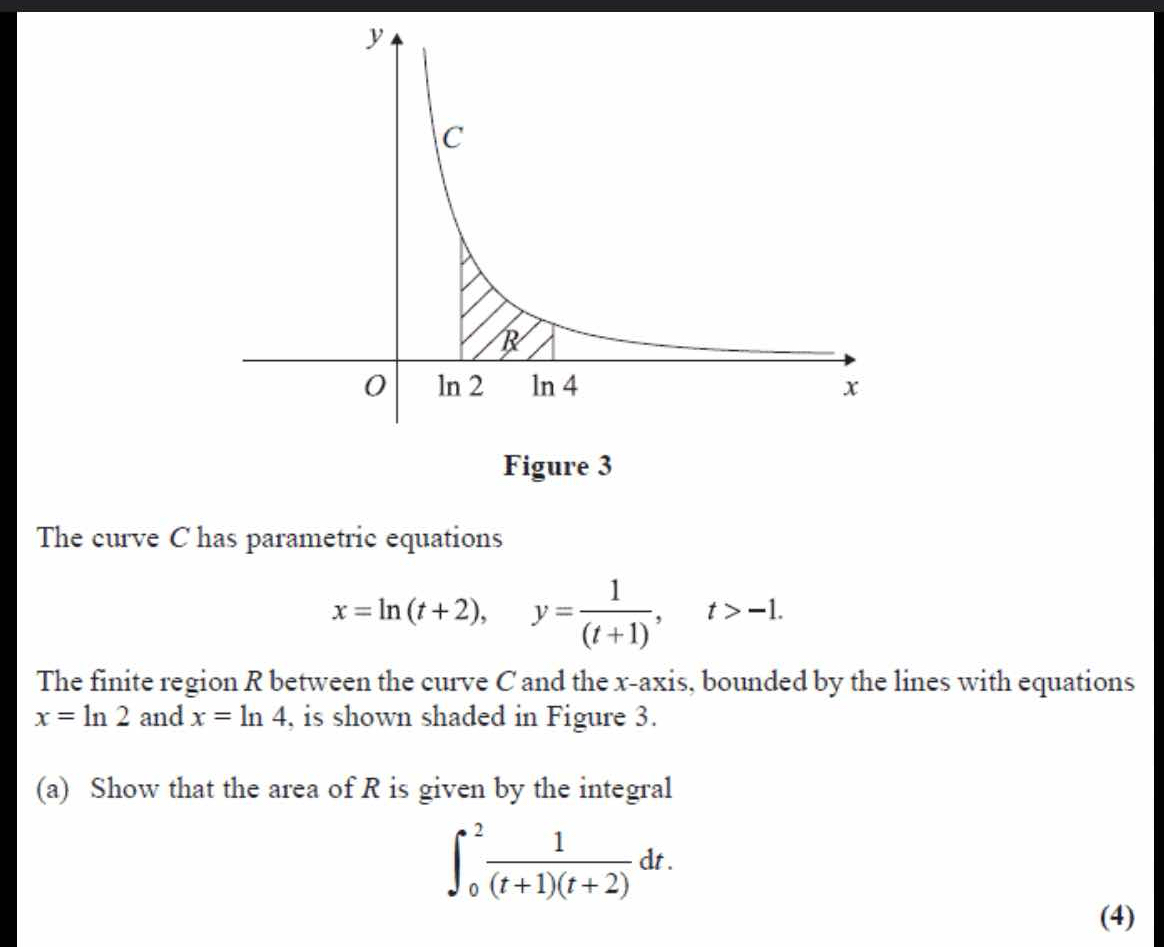
The question is asking to demonstrate that the area of the region R between the curve C and the x-axis, bounded by specific vertical lines, can be expressed using a given integral.
Answer
The area of the region \( R \) is given by the integral \( A = \int_{0}^{2} \frac{1}{(t + 1)(t + 2)} \, dt \).
Answer for screen readers
The area ( A ) of the region ( R ) is given by the integral [ A = \int_{0}^{2} \frac{1}{(t + 1)(t + 2)} , dt. ]
Steps to Solve
-
Identify the Parametric Equations
The given parametric equations for the curve ( C ) are: [ x = \ln(t + 2), \quad y = \frac{1}{(t + 1)}, \quad t > -1. ]
-
Find the Limits of Integration
We need to determine the values of ( t ) corresponding to the vertical lines ( x = \ln(2) ) and ( x = \ln(4) ).
- For ( x = \ln(2) ): [ \ln(t + 2) = \ln(2) \implies t + 2 = 2 \implies t = 0. ]
- For ( x = \ln(4) ): [ \ln(t + 2) = \ln(4) \implies t + 2 = 4 \implies t = 2. ]
-
Express the Area in Terms of ( t )
The area ( A ) under the curve between ( t = 0 ) and ( t = 2 ) can be calculated by integrating the function ( y ) with respect to ( x ): [ A = \int_{0}^{2} y \frac{dx}{dt} , dt. ]
-
Calculate ( \frac{dx}{dt} )
First, find ( \frac{dx}{dt} ): [ \frac{dx}{dt} = \frac{d}{dt}[\ln(t + 2)] = \frac{1}{t + 2}. ]
-
Substitute ( y ) and ( \frac{dx}{dt} ) into the Area Formula
Substitute ( y ) and ( \frac{dx}{dt} ) into the area integral: [ A = \int_{0}^{2} \frac{1}{t + 1} \cdot \frac{1}{t + 2} , dt. ]
-
Final Formulation
Hence, the area ( A ) can be expressed as: [ A = \int_{0}^{2} \frac{1}{(t + 1)(t + 2)} , dt, ] which is the required integral.
The area ( A ) of the region ( R ) is given by the integral [ A = \int_{0}^{2} \frac{1}{(t + 1)(t + 2)} , dt. ]
More Information
The integral calculates the area under the curve ( y = \frac{1}{(t+1)} ) bounded between ( t = 0 ) and ( t = 2 ). This demonstrates the relationship between parametric equations and area calculation in a specific region.
Tips
- Forgetting Limits of Integration: Make sure to compute ( t ) values carefully for the given vertical lines.
- Incorrect Calculation of ( \frac{dx}{dt} ): A miscalculation here affects the area integral.
AI-generated content may contain errors. Please verify critical information