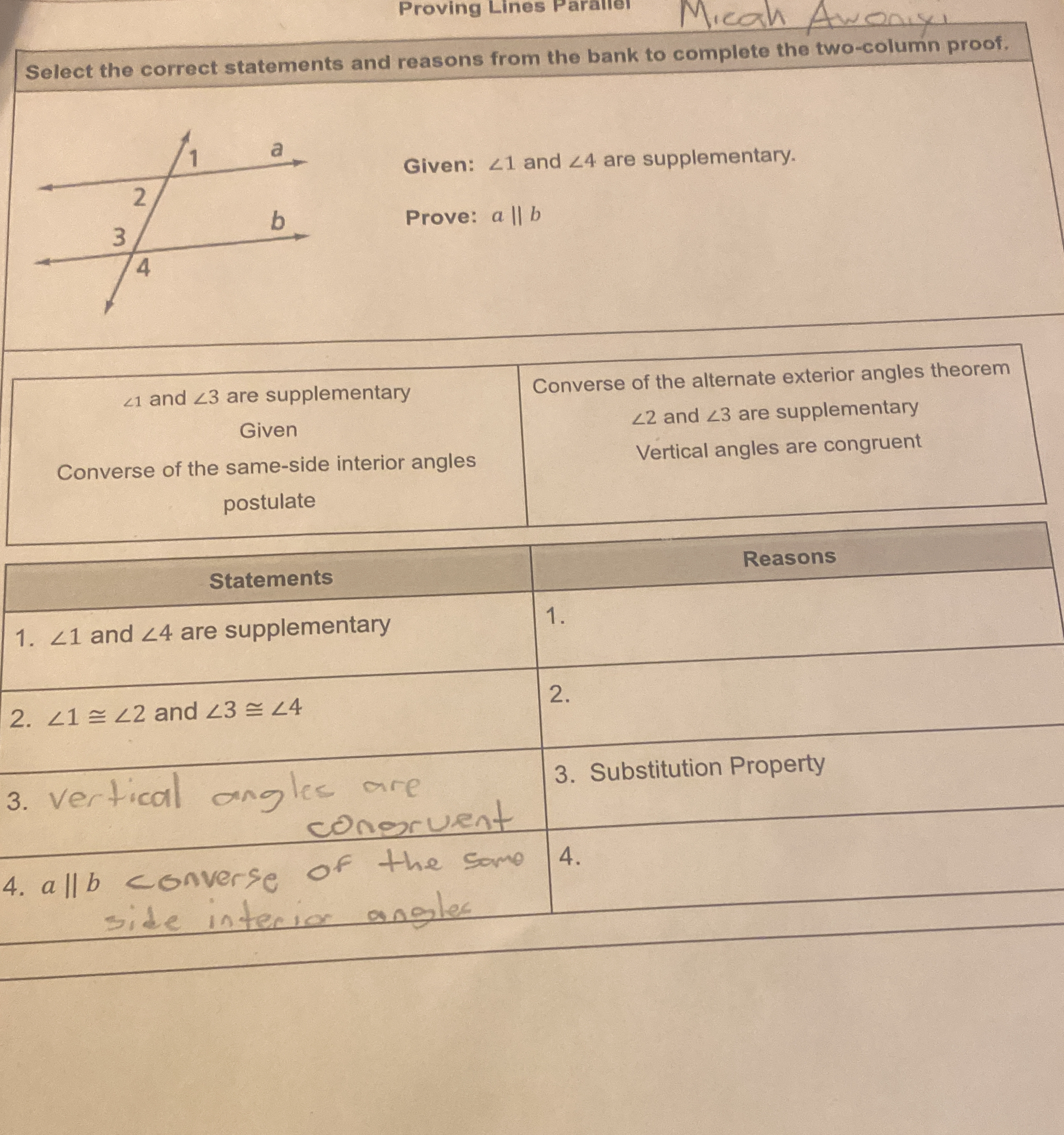
Select the correct statements and reasons from the bank to complete the two-column proof.
Understand the Problem
The question is asking for assistance in completing a two-column proof related to parallel lines based on given angle relationships. It requires selecting appropriate statements and reasons from a provided bank to support the proof that lines a and b are parallel.
Answer
$a \parallel b$
Answer for screen readers
Lines $a$ and $b$ are parallel, proven using the angle relationships.
Steps to Solve
-
State the Given Information The given information is that $\angle 1$ and $\angle 4$ are supplementary. This means: $$ \angle 1 + \angle 4 = 180^\circ $$
-
Identify Vertical Angles Since $\angle 1$ and $\angle 2$ are vertical angles, they are congruent. Therefore: $$ \angle 1 \cong \angle 2 $$
-
Use the Angle Relationship for $\angle 3$ From the given information, we also notice that $\angle 2$ and $\angle 3$ are supplementary: $$ \angle 2 + \angle 3 = 180^\circ $$
-
Apply the Substitute Property Since $\angle 1 \cong \angle 2$, we can substitute $\angle 2$ with $\angle 1$. Then we have: $$ \angle 1 + \angle 3 = 180^\circ $$
-
Conclude with the Converse of Same-Side Interior Angles Postulate From the resultant relationship of $\angle 1$ and $\angle 3$, we conclude that lines $a$ and $b$ are parallel: $$ a \parallel b $$
Lines $a$ and $b$ are parallel, proven using the angle relationships.
More Information
The proof follows logically from the properties of supplementary and vertical angles, showing that the two lines do not intersect and thus are parallel.
Tips
- Confusing vertical angles with corresponding angles can lead to incorrect conclusions about parallel lines. Remember: vertical angles are always congruent, while corresponding angles can help prove lines are parallel through other properties.
- Not clearly labeling the steps in a proof can cause confusion. Always ensure each step logically follows from the previous one.
AI-generated content may contain errors. Please verify critical information