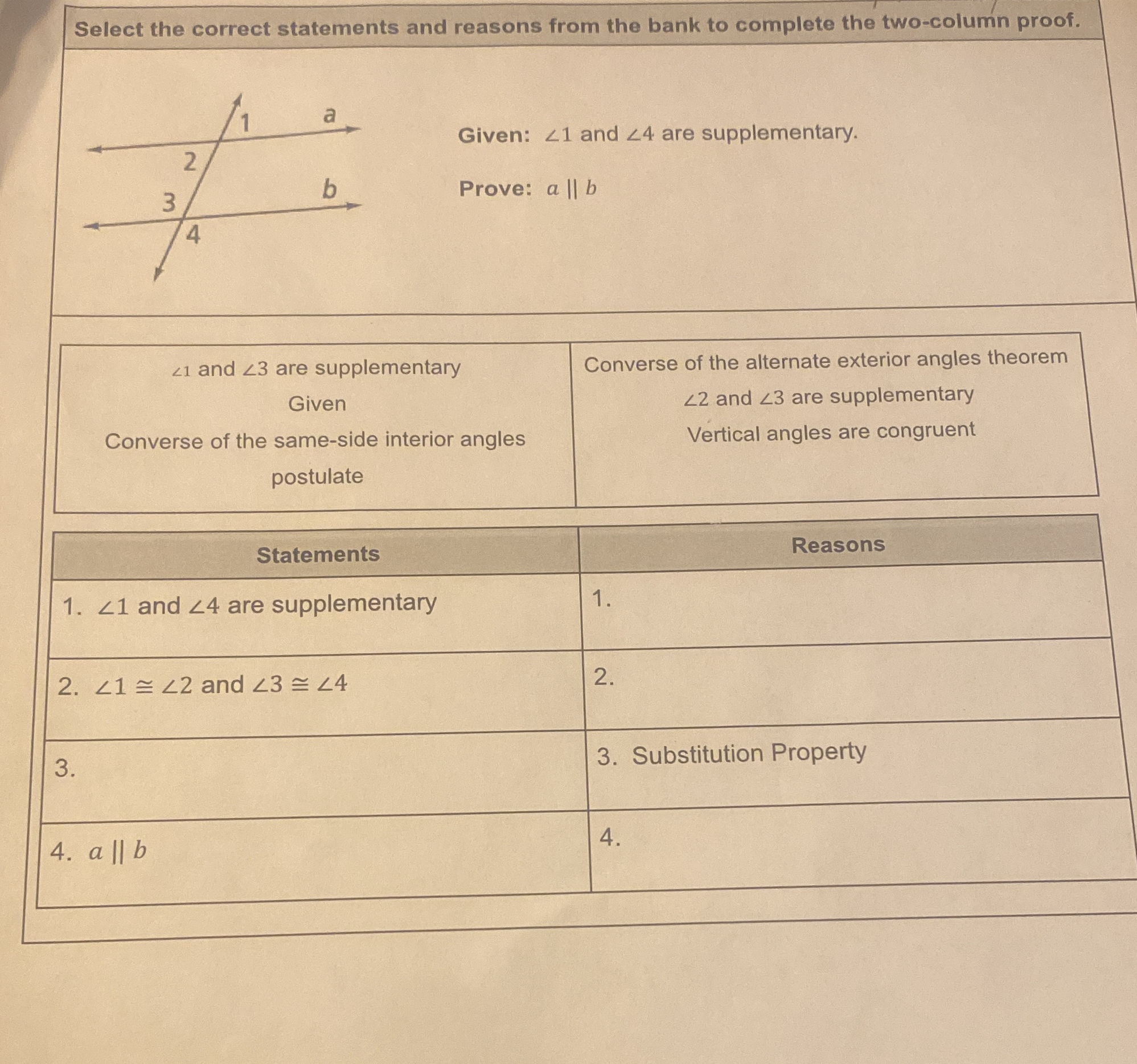
Select the correct statements and reasons from the bank to complete the two-column proof for proving that lines a and b are parallel given that angles 1 and 4 are supplementary.
Understand the Problem
The question involves completing a two-column proof utilizing logical statements and corresponding reasons related to angles and parallel lines. The user needs to identify which statements and reasons from a list are required to establish that two lines are parallel based on given information about angles.
Answer
1. $\angle 1$ and $\angle 4$ are supplementary. (Given) 2. $\angle 1 \cong \angle 2$ and $\angle 3 \cong \angle 4$. (Vertical angles are congruent) 3. Substitution Property. 4. $a \parallel b$. (Converse of the same-side interior angles postulate)
Answer for screen readers
The correct statements and reasons to complete the proof are:
- $\angle 1$ and $\angle 4$ are supplementary. (Given)
- $\angle 1 \cong \angle 2$ and $\angle 3 \cong \angle 4$. (Vertical angles are congruent)
- Substitution Property.
- $a \parallel b$. (Converse of the same-side interior angles postulate)
Steps to Solve
- Identify Given Information
We know from the problem that angles $\angle 1$ and $\angle 4$ are supplementary.
- Establish Angle Relationships
Since $\angle 1$ and $\angle 4$ are supplementary, we can conclude that their measures add up to $180^\circ$: $$ m\angle 1 + m\angle 4 = 180^\circ $$
- Use Vertical Angles Theorem
Assuming lines $a$ and $b$ intersect at a point, $\angle 1$ and $\angle 3$ are vertical angles. According to the vertical angles theorem, vertical angles are congruent: $$ m\angle 1 = m\angle 3 $$
- Show Congruent Angles
Now, we can set up the equation: $$ m\angle 3 + m\angle 4 = 180^\circ $$ This implies that $\angle 3$ and $\angle 4$ are also supplementary.
- Conclude with Corresponding Angles Postulate
According to the converse of the same-side interior angles postulate, if two angles are supplementary and both are formed by a transversal with two lines, the lines are parallel: $$ \text{Since } m\angle 1 + m\angle 4 = 180^\circ, \text{ we conclude that } a \parallel b. $$
The correct statements and reasons to complete the proof are:
- $\angle 1$ and $\angle 4$ are supplementary. (Given)
- $\angle 1 \cong \angle 2$ and $\angle 3 \cong \angle 4$. (Vertical angles are congruent)
- Substitution Property.
- $a \parallel b$. (Converse of the same-side interior angles postulate)
More Information
This proof utilizes properties of supplementary angles and vertical angles to show that two lines cut by a transversal are parallel, based on angle relationships.
Tips
- Confusing the definitions of supplementary and congruent angles.
- Not applying the vertical angles theorem correctly.
- Failing to recognize that supplementary angles can lead to parallel lines using the converse of the same-side interior angles postulate.
AI-generated content may contain errors. Please verify critical information