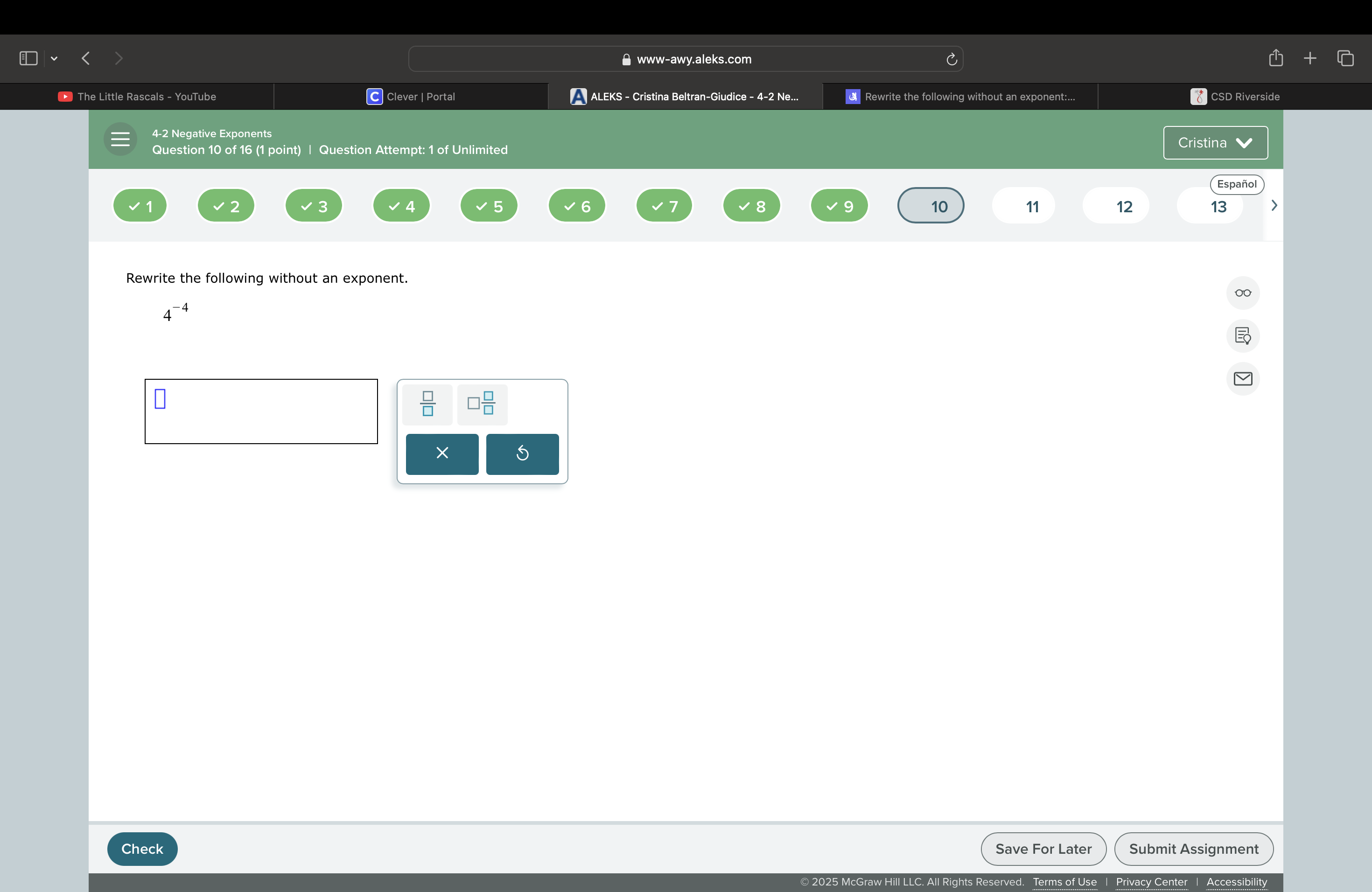
Rewrite the following without an exponent: 4^(-4)
Understand the Problem
The question is asking to rewrite the expression 4 raised to the power of -4 without using exponents. This involves applying the rule for negative exponents, which states that a^(-n) is equivalent to 1/(a^n). Therefore, we need to express 4^(-4) as 1/(4^4).
Answer
The expression $4^{-4}$ can be rewritten as $\frac{1}{256}$.
Answer for screen readers
The expression $4^{-4}$ rewritten without using an exponent is $\frac{1}{256}$.
Steps to Solve
-
Apply the Negative Exponent Rule
Using the rule for negative exponents, we rewrite the expression $4^{-4}$ as:
$$4^{-4} = \frac{1}{4^{4}}$$ -
Calculate $4^4$
Now, let's calculate $4^4$ to find its numerical value.
$$4^4 = 4 \times 4 \times 4 \times 4 = 256$$ -
Substitute the Value
We substitute the calculated value of $4^4$ into our rewritten expression.
So, we have:
$$\frac{1}{4^{-4}} = \frac{1}{256}$$
The expression $4^{-4}$ rewritten without using an exponent is $\frac{1}{256}$.
More Information
The expression $4^{-4}$ means that we are dealing with the reciprocal of $4$ raised to the power of $4$, which equals $256$. When we rewrite it, we emphasize that negative exponents indicate a reciprocal or division.
Tips
- Confusing Negative Exponents: A common mistake is to misinterpret $4^{-4}$ as a negative value instead of a reciprocal.
- Incorrect Calculation of Powers: Make sure not to miscalculate the power, which should be $256$ for $4^4$.
AI-generated content may contain errors. Please verify critical information