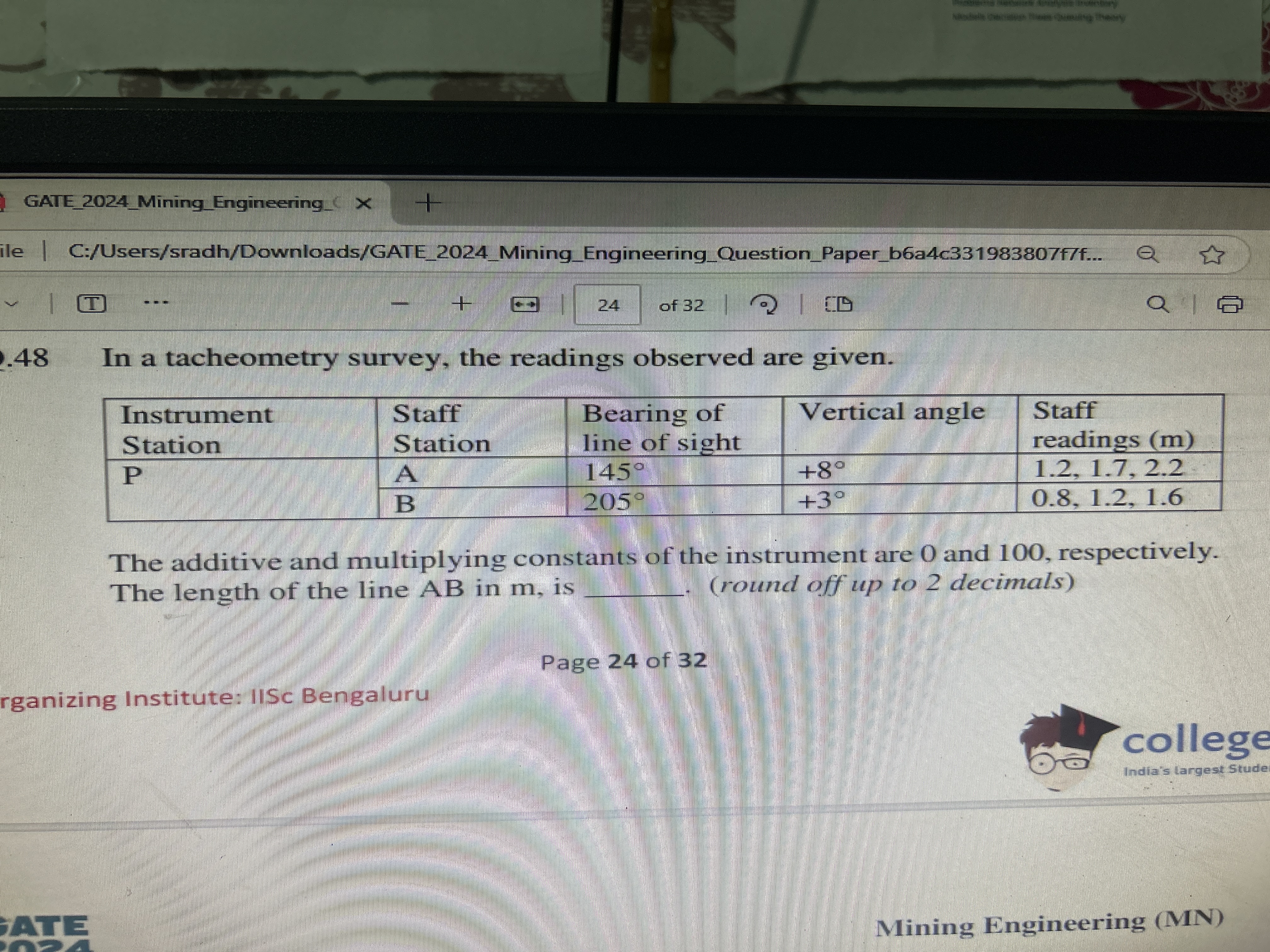
In a tacheometry survey, the readings observed are given. The additive and multiplying constants of the instrument are 0 and 100, respectively. The length of the line AB in m is __... In a tacheometry survey, the readings observed are given. The additive and multiplying constants of the instrument are 0 and 100, respectively. The length of the line AB in m is _______ (round off up to 2 decimals). Read more

Steps to Solve

1. Calculate Horizontal Distances for Both Stations

For station A, the formula to calculate the horizontal distance ($D_A$) is:

$$ D_A = (S_A - C) \times K $$

Where:

• $S_A$ is the staff reading at A (1.2 m)
• $C$ is the additive constant (0)
• $K$ is the multiplying constant (100)

Thus,

$$ D_A = (1.2 - 0) \times 100 = 120 \text{ m} $$

For station B, using the staff readings (0.8 m), the formula becomes:

$$ D_B = (S_B - C) \times K $$

Where:

• $S_B$ is the staff reading at B (0.8 m)

Thus,

$$ D_B = (0.8 - 0) \times 100 = 80 \text{ m} $$

2. Adjust for Vertical Angles

Now we need to calculate the lengths that account for the vertical angles.

For station A with a vertical angle of $+8^\circ$, the distance can be adjusted as:

$$ L_A = D_A \cos(\theta_A) $$

Where $\theta_A = 8^\circ$.

Thus,

$$ L_A = 120 \cos(8^\circ) $$

For station B with a vertical angle of $+3^\circ$, the length is:

$$ L_B = D_B \cos(\theta_B) $$

Where $\theta_B = 3^\circ$.

Thus,

$$ L_B = 80 \cos(3^\circ) $$

3. Calculate the Total Length of AB

Now using the formula for the length of the line AB ($L_{AB}$):

$$ L_{AB} = L_A + L_B $$

Substituting the values:

$$ L_{AB} = L_A + L_B = 120 \cos(8^\circ) + 80 \cos(3^\circ) $$

4. Perform the Necessary Calculations

Now calculate $L_A$ and $L_B$:

$$ L_A = 120 \times 0.9903 \approx 118.84 \text{ m} $$

$$ L_B = 80 \times 0.9980 \approx 79.84 \text{ m} $$

Then,

$$ L_{AB} = 118.84 + 79.84 = 198.68 \text{ m} $$

5. Round to Two Decimal Places

The final length of line AB, rounded to two decimal places, is:

$$ L_{AB} \approx 198.68 \text{ m} $$