If l and m are parallel lines and the angle on line l is 105° while the angle on line m is (3x - 18)°, what is the value of x?
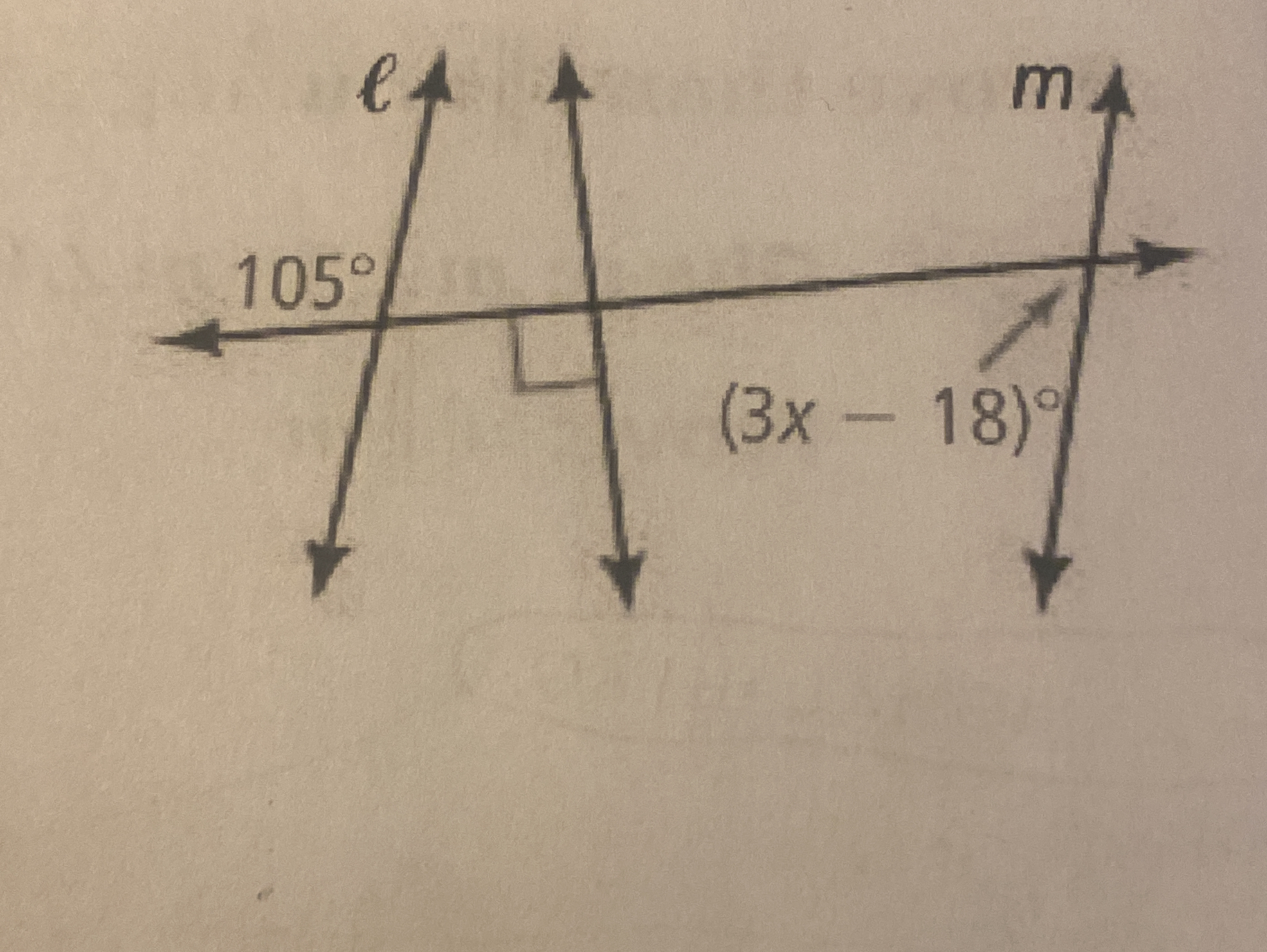
Understand the Problem
The question involves determining the value of x using the properties of angles formed by two parallel lines and a transversal. Specifically, we can use the fact that these alternate interior angles sum to 180 degrees.
Answer
The value of $x$ is $31$.
Answer for screen readers
The value of $x$ is $31$.
Steps to Solve
- Identify Angle Relationships
Since the lines $l$ and $m$ are parallel, and the transversal creates angles, we can say that the two angles (the given $105^\circ$ and the expression $(3x - 18)^\circ$) are supplementary. This means that they add up to $180^\circ$.
- Set Up the Equation
Set up the equation based on the property of supplementary angles: $$ 105 + (3x - 18) = 180 $$
- Simplify the Equation
Combine the constant terms in the equation: $$ 105 - 18 + 3x = 180 $$
This simplifies to: $$ 87 + 3x = 180 $$
- Isolate the Variable
Now, isolate $3x$ by subtracting $87$ from both sides: $$ 3x = 180 - 87 $$
This simplifies to: $$ 3x = 93 $$
- Solve for x
Finally, divide both sides by $3$ to solve for $x$: $$ x = \frac{93}{3} = 31 $$
The value of $x$ is $31$.
More Information
This problem utilizes the properties of angles formed by two parallel lines and a transversal, specifically focusing on supplementary angles, which sum to $180^\circ$.
Tips
- Misidentifying the angles as equal rather than supplementary. Remember that angles that are on the same side of the transversal must be added together to equal $180^\circ$.
- Forgetting to simplify the equation correctly. Ensure to combine like terms properly to avoid errors in calculations.
AI-generated content may contain errors. Please verify critical information