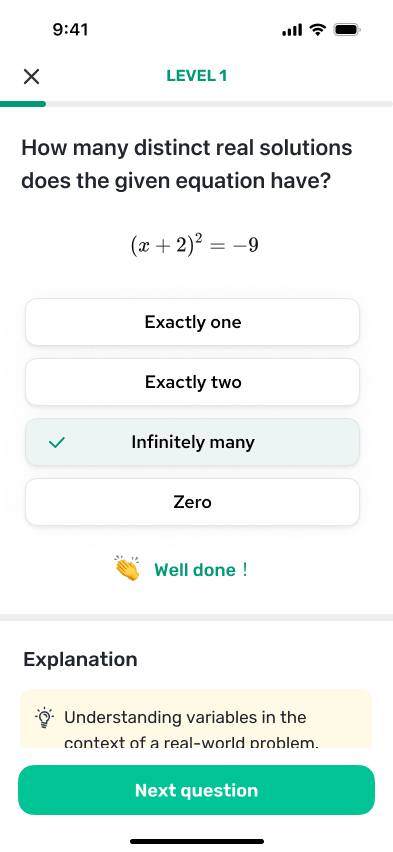
How many distinct real solutions does the given equation have? (x + 2)^2 = -9
Understand the Problem
The question is asking to determine the number of distinct real solutions to the quadratic equation (x + 2)^2 = -9. To solve this, we need to analyze the equation and consider the characteristics of quadratic functions and their solutions.
Answer
The equation $(x + 2)^2 = -9$ has zero distinct real solutions.
Answer for screen readers
The number of distinct real solutions to the equation $(x + 2)^2 = -9$ is zero.
Steps to Solve
- Identify the structure of the equation
The equation given is $ (x + 2)^2 = -9 $. This is a quadratic equation.
- Analyze the right side of the equation
Notice that the right side of the equation is $ -9 $. The square of any real number is always non-negative, meaning $ (x + 2)^2 \geq 0 $ for all real $ x $.
- Compare values
Since $ (x + 2)^2 \geq 0 $, it cannot equal a negative number. Thus, there are no real numbers $ x $ such that $ (x + 2)^2 = -9 $.
- Conclusion about the solutions
Since there are no real numbers that can satisfy the equation, we conclude that there are zero distinct real solutions.
The number of distinct real solutions to the equation $(x + 2)^2 = -9$ is zero.
More Information
Quadratic equations can typically have zero, one, or two distinct real solutions, depending on the value of the discriminant. In this case, since we are equating a square to a negative number, the solutions are not possible in the realm of real numbers.
Tips
null
AI-generated content may contain errors. Please verify critical information