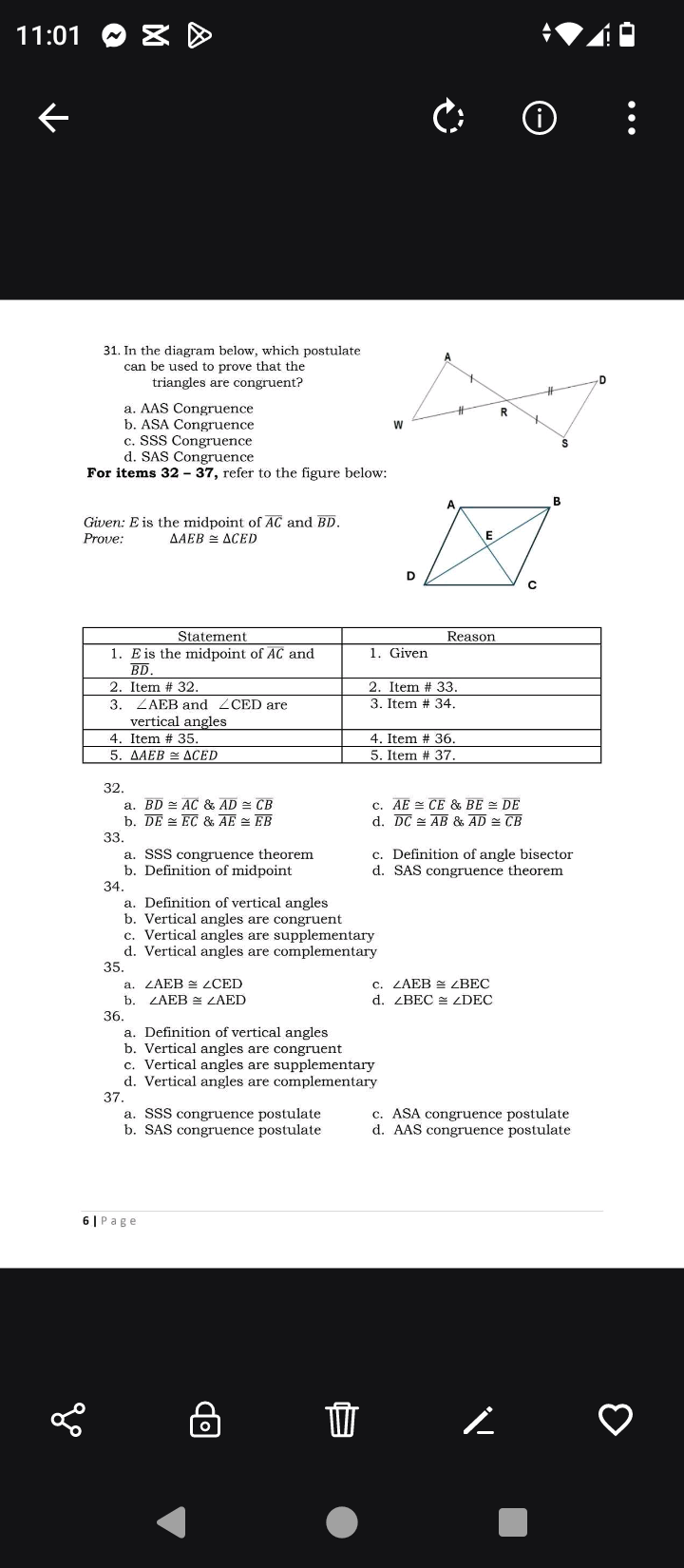
Geometry questions on triangle congruence postulates and properties of geometric figures. Question 31 asks: In the diagram below, which postulate can be used to prove that the tria... Geometry questions on triangle congruence postulates and properties of geometric figures. Question 31 asks: In the diagram below, which postulate can be used to prove that the triangles are congruent? Options: AAS Congruence, ASA Congruence, SSS Congruence, SAS Congruence. Questions 32-37 refer to the figure below. Given: E is the midpoint of AC and BD. Prove: ΔΑΕΒ ≅ ΔCED. Fill in the blanks in the table.
Understand the Problem
The image contains a series of geometry questions, specifically focusing on triangle congruence postulates and properties of geometric figures. Questions 32-37 each correspond to filling in the blank spots in a table, the statements are on the left and the corresponding reasons are on the right.
Answer
31. d. SAS Congruence 32. b. DE ≅ EC & AE ≅ EB 33. b. Definition of midpoint 34. a. Definition of vertical angles 35. a. ∠AEB ≅ ∠CED 36. b. Vertical angles are congruent 37. b. SAS congruence postulate
Answer for screen readers
-
The postulate that can be used to prove that the triangles are congruent is d. SAS Congruence.
-
b. DE ≅ EC & AE ≅ EB
-
b. Definition of midpoint
-
a. Definition of vertical angles
-
a. ∠AEB ≅ ∠CED
-
b. Vertical angles are congruent
-
b. SAS congruence postulate
Steps to Solve
-
Identify the Given Information The given information states that ( E ) is the midpoint of both ( AC ) and ( BD ). This implies that ( AE = EC ) and ( BE = ED ).
-
Determine the First Reason For item #32, we need to express the lengths that can be deduced from the midpoint property. Hence, the correct reason would be ( DE \cong EC ) and ( AE \cong BE ).
-
Determine the Next Reason For item #33, since E is the midpoint, the appropriate reason we refer to is the "Definition of Midpoint" which verifies that segments from a midpoint to the ends are equal.
-
Establish Vertical Angles For item #34, since ( \angle AEB ) and ( \angle CED ) are opposite angles formed when two lines intersect, we state they are vertical angles.
-
State Congruence of Angles For item #35, as established, we can say ( \angle AEB \cong \angle CED ).
-
Conclude on Angle Properties For item #36, we can use the fact that vertical angles are congruent, which justifies our previous statement about the angles.
-
Final Congruence Statement Finally, for item #37, with the corresponding sides and angle equalities established, we can conclude using the SAS Congruence Theorem since we have two sides and the angle between them congruent.
-
The postulate that can be used to prove that the triangles are congruent is d. SAS Congruence.
-
b. DE ≅ EC & AE ≅ EB
-
b. Definition of midpoint
-
a. Definition of vertical angles
-
a. ∠AEB ≅ ∠CED
-
b. Vertical angles are congruent
-
b. SAS congruence postulate
More Information
The study of triangle congruence is fundamental in geometry and is used extensively in proofs and problem-solving. The congruence postulates (SAS, SSS, ASA, AAS) are essential tools that allow us to establish that two triangles are identical in shape and size.
Tips
- Confusing Midpoints and Bisectors: When identifying segments, ensure you relate equal lengths to the definitions of midpoints rather than misapplying them to angle bisectors.
- Not Recognizing Vertical Angles: Students often overlook the concept of vertical angles being equal and may mistakenly consider them supplementary.
AI-generated content may contain errors. Please verify critical information