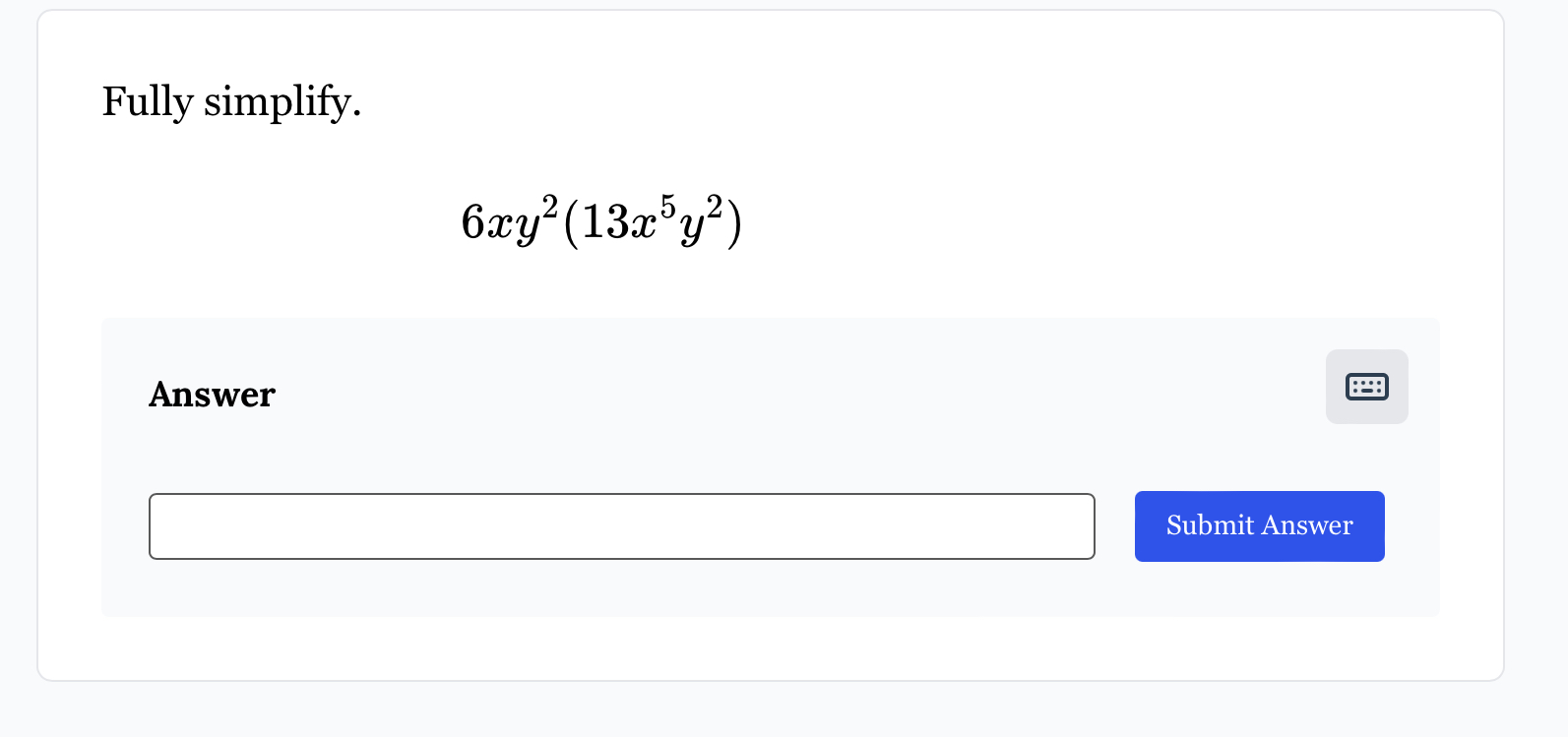
Fully simplify 6xy²(13x⁵y²).
Understand the Problem
The question is asking to fully simplify the expression 6xy²(13x⁵y²). This involves multiplying the coefficients and combining the variables according to the rules of exponents.
Answer
$78x^6y^4$
Answer for screen readers
The fully simplified expression is $78x^6y^4$.
Steps to Solve
- Multiply the coefficients
First, multiply the numerical coefficients:
$$ 6 \times 13 = 78 $$
- Multiply the x terms
Next, multiply the $x$ terms. You have $x$ (which can be expressed as $x^1$) and $x^5$. According to the rules of exponents:
$$ x^1 \times x^5 = x^{1+5} = x^6 $$
- Multiply the y terms
Now, multiply the $y$ terms. You have $y^2$ and $y^2$. Again, use the rules of exponents:
$$ y^2 \times y^2 = y^{2+2} = y^4 $$
- Combine all parts
Now, combine all parts together:
$$ 78 x^6 y^4 $$
The fully simplified expression is $78x^6y^4$.
More Information
This expression results from multiplying the coefficients and applying the laws of exponents for the variable parts. Simplifying expressions like this is a fundamental skill in algebra, necessary for solving equations and making calculations easier.
Tips
- Forgetting to apply the exponent rule correctly when multiplying variables. Always remember to add the exponents when multiplying like bases.
- Miscalculating the coefficients during multiplication. Always double-check numerical calculations to avoid errors.
AI-generated content may contain errors. Please verify critical information