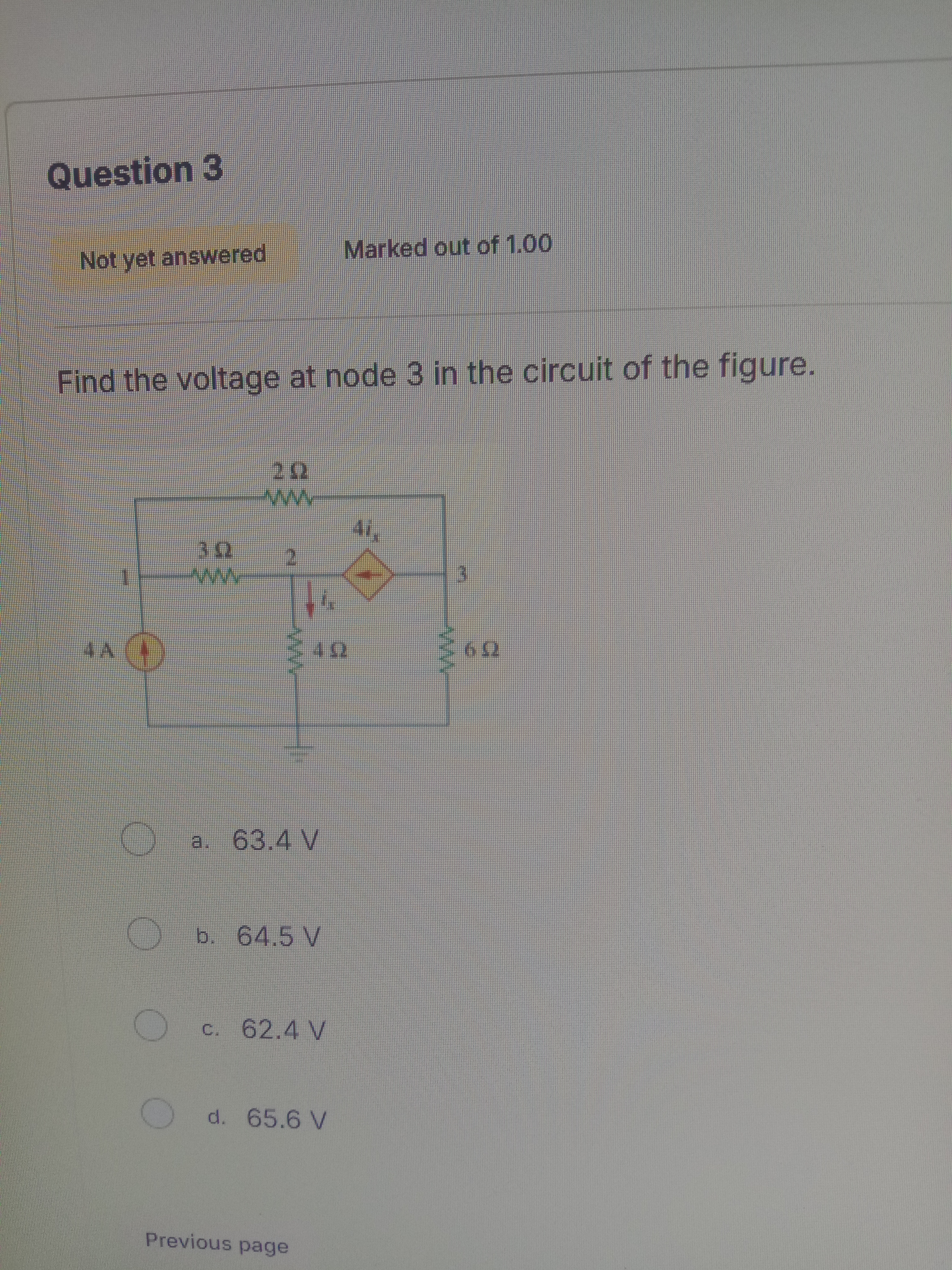
Find the voltage at node 3 in the circuit of the figure.

Steps to Solve

1. Identify circuit components and connections

Examine the circuit diagram to identify resistances and the current source. The circuit has resistors (2Ω, 3Ω, 4Ω, 4Ω, and 6Ω) and a current source of 4A.

2. Apply Kirchhoff's Current Law (KCL)

At node 3, apply KCL which states that the sum of currents entering a node equals the sum of currents leaving the node.

$$ I_{in} = I_{out} $$

3. Express currents in terms of voltages and resistances

Let ( V_3 ) be the voltage at node 3. The currents can be expressed as:

• For the 4Ω resistor: ( I_1 = \frac{V_3 - V_2}{4} )
• For the 4Ω resistor connected to the 6Ω resistor: ( I_2 = \frac{V_3}{6} )
• Between node 3 and the lower node through the 4Ω resistor: ( I_3 = \frac{V_3}{4} )

4. Set up the KCL equation at node 3

From KCL at node 3, inputting the values, we get:

$$ 4 = \frac{V_3 - V_2}{4} + \frac{V_3}{6} + \frac{V_3}{4} $$

5. Simplify and solve the equation

Combine terms and solve for ( V_3 ). To do this, multiply through by 12 to eliminate denominators:

$$ 48 = 3(V_3 - V_2) + 2V_3 + 3V_3 $$

6. Calculate Voltage Drop across the resistor

Assuming ( V_2 ) is known or defined, substitute ( V_2 ) into the simplified KCL equation and solve for ( V_3 ).

7. Find the final value of ( V_3 )

Using the calculated value for ( V_3 ), compare with the given options to find the correct voltage at node 3.