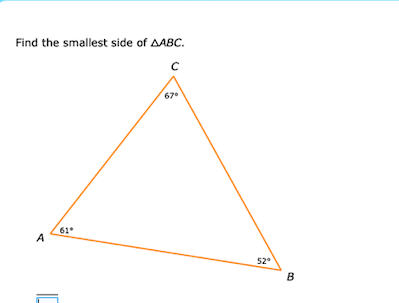
Find the smallest side of triangle ABC.
Understand the Problem
The question is asking to determine which side of triangle ABC is the smallest based on the given angles. We'll use the fact that in a triangle, the side opposite the smallest angle is the shortest.
Answer
The smallest side of triangle \( ABC \) is side \( AC \).
Answer for screen readers
The smallest side of triangle ( ABC ) is side ( AC ).
Steps to Solve
- Identify the Angles The angles of triangle $ABC$ are given as follows:
- $\angle A = 61^\circ$
- $\angle B = 52^\circ$
- $\angle C = 67^\circ$
- Determine the Smallest Angle To find the smallest side, first identify the smallest angle among the three:
- Compare $61^\circ$, $52^\circ$, and $67^\circ$.
The smallest angle is $\angle B = 52^\circ$.
- Find the Smallest Side According to the rule that states the side opposite the smallest angle is the shortest, we need to identify which side corresponds to $\angle B$.
- The side opposite $\angle B$ is side $AC$.
- Conclusion Thus, side $AC$ is the smallest side of triangle $ABC$.
The smallest side of triangle ( ABC ) is side ( AC ).
More Information
In any triangle, the relationship between the angles and sides dictates that the side opposite the smallest angle is the shortest. This principle is fundamental in triangle geometry and helps in various proofs and problem-solving scenarios.
Tips
-
Misidentifying Angles: Sometimes, students might confuse which angle corresponds to which side. Always double-check which angle is opposite which side.
-
Calculation Errors: Incorrectly adding or comparing angles can lead to wrong conclusions about which is the smallest angle. Make sure to carefully evaluate each angle.
AI-generated content may contain errors. Please verify critical information