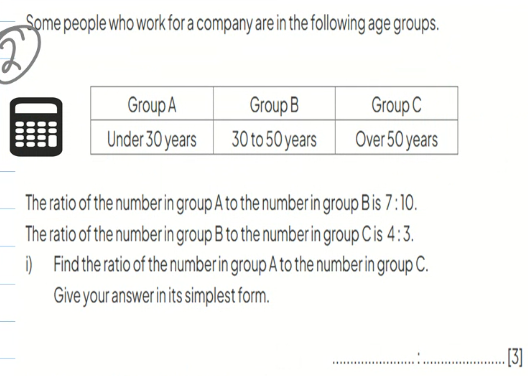
Find the ratio of the number in group A to the number in group C. Give your answer in its simplest form.

Steps to Solve

1. Define the Variables for Groups A and B

Let the number of people in Group A be $7x$ and in Group B be $10x$, based on the given ratio of $7:10$.

2. Express Group C in Terms of Group B

Using the ratio between Groups B and C, which is $4:3$, we can let the number in Group B be $4y$ and the number in Group C be $3y$.

3. Set Up the Equations

From the previous step, we have:

• Group B: $10x = 4y$

  Rearranging this for $y$ gives:

  $$ y = \frac{10x}{4} = \frac{5x}{2} $$

4. Find the Number in Group C Using y

Now, substitute $y$ into the expression for Group C:

$$ \text{Number in Group C} = 3y = 3 \left(\frac{5x}{2}\right) = \frac{15x}{2} $$

5. Calculate the Ratio of Group A to Group C

Now we have:

• Group A: $7x$
• Group C: $\frac{15x}{2}$

The ratio can be calculated as:

$$ \text{Ratio } A:C = \frac{7x}{\frac{15x}{2}} = \frac{7x \cdot 2}{15x} = \frac{14}{15} $$

6. Simplifying the Ratio

The ratio of the number in Group A to Group C is simplified to:

$$ A:C = 14:15 $$