Find the equation of the equation with the root (α² - β²) = 3 and α - β = 2.
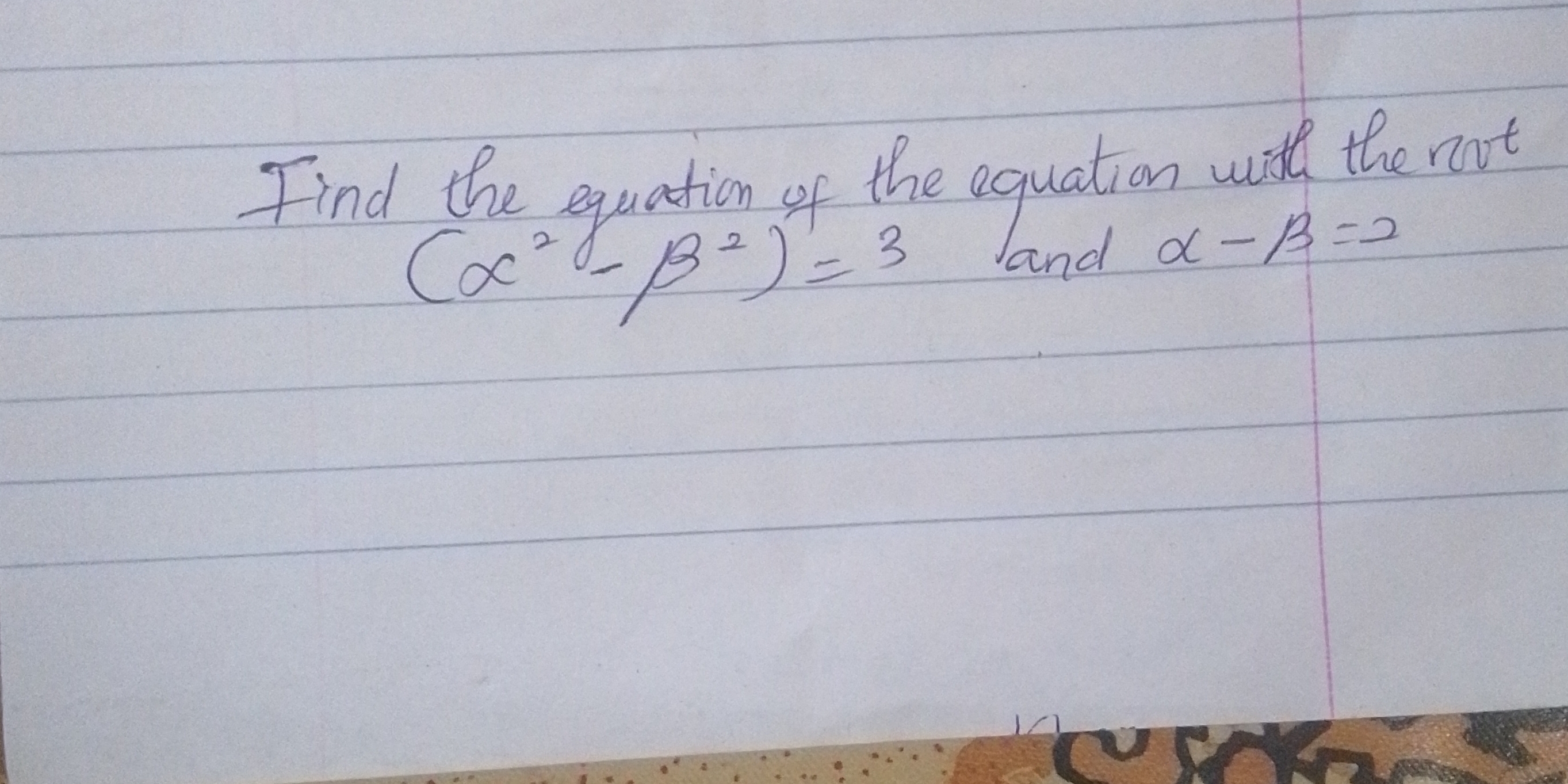
Understand the Problem
The question asks for the formation of an equation given specific conditions involving the roots (α² - β² = 3) and (α - β = 2). The goal is to determine an equation based on these roots.
Answer
The equation is $$x^2 - \frac{3}{2}x + \frac{7}{16} = 0$$.
Answer for screen readers
The equation is $$x^2 - \frac{3}{2}x + \frac{7}{16} = 0$$.
Steps to Solve
-
Define the Variables Let $\alpha$ and $\beta$ be the roots of the equation. We have two conditions: $$\alpha^2 - \beta^2 = 3$$ and $$\alpha - \beta = 2$$.
-
Express $\alpha$ in terms of $\beta$ From the second condition, we can rearrange the equation: $$\alpha = \beta + 2$$.
-
Substitute in the first condition Substituting $\alpha$ in the first equation: $$(\beta + 2)^2 - \beta^2 = 3$$.
-
Expand the equation Expanding the left side: $$\beta^2 + 4\beta + 4 - \beta^2 = 3$$. This simplifies to: $$4\beta + 4 = 3$$.
-
Solve for $\beta$ Rearranging gives us: $$4\beta = 3 - 4$$ which simplifies to: $$4\beta = -1$$, thus, $$\beta = -\frac{1}{4}$$.
-
Find $\alpha$ Now substituting $\beta$ back to find $\alpha$: $$\alpha = -\frac{1}{4} + 2 = \frac{7}{4}$$.
-
Form the Equation from Roots Using the roots $\alpha$ and $\beta$, the quadratic polynomial whose roots are given by the formula: $$x^2 - (\alpha + \beta)x + \alpha \beta = 0$$.
-
Calculate the Sum and Product of Roots
- Sum of the roots: $$\alpha + \beta = \frac{7}{4} - \frac{1}{4} = \frac{6}{4} = \frac{3}{2}$$.
- Product of the roots: $$\alpha \beta = \left(\frac{7}{4}\right) \left(-\frac{1}{4}\right) = -\frac{7}{16}$$.
- Write the Equation Now, we can substitute into the standard form: $$x^2 - \left(\frac{3}{2}\right)x - \left(-\frac{7}{16}\right) = 0$$, which simplifies to: $$x^2 - \frac{3}{2}x + \frac{7}{16} = 0$$.
The equation is $$x^2 - \frac{3}{2}x + \frac{7}{16} = 0$$.
More Information
This equation represents a quadratic function with roots $\alpha = \frac{7}{4}$ and $\beta = -\frac{1}{4}$, satisfying the given conditions on their differences and squares.
Tips
- Neglecting to simplify the calculations clearly when substituting values.
- Forgetting to apply the signs correctly when finding roots.
AI-generated content may contain errors. Please verify critical information