Find the constants a, b, c, so that F = (x + 2y + az)i + (bx - 3y - z)j + (4x + cy + 2z)k is irrotational and hence find the scalar function φ such that F = ∇φ.
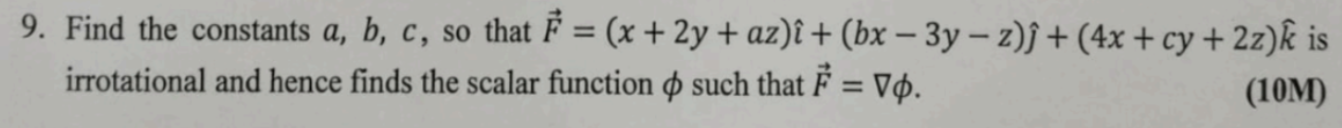
Understand the Problem
The question asks to find constants a, b, and c such that the given vector field is irrotational, meaning its curl is zero, and to identify a scalar function φ satisfying ∇φ = F.
Answer
The constants are \( a = 4 \), \( b = 2 \), \( c = -1 \), and the scalar function is $$ \phi = \frac{x^2}{2} + 2xy + 4xz - \frac{3y^2}{2} + z^2 + C. $$
Answer for screen readers
The constants are ( a = 4 ), ( b = 2 ), ( c = -1 ).
The scalar function is:
$$ \phi = \frac{x^2}{2} + 2xy + 4xz - \frac{3y^2}{2} + z^2 + C $$
Steps to Solve
- Identifying the vector field components
The vector field is given by:
$$ \vec{F} = (x + 2y + az)\hat{i} + (bx - 3y - z)\hat{j} + (4x + cy + 2z)\hat{k} $$
We define the components as:
- ( F_1 = x + 2y + az )
- ( F_2 = bx - 3y - z )
- ( F_3 = 4x + cy + 2z )
- Finding the curl of the vector field
A vector field ( \vec{F} ) is irrotational if the curl ( \nabla \times \vec{F} = 0 ). We compute the components of the curl:
$$ \nabla \times \vec{F} = \begin{vmatrix} \hat{i} & \hat{j} & \hat{k} \ \frac{\partial}{\partial x} & \frac{\partial}{\partial y} & \frac{\partial}{\partial z} \ F_1 & F_2 & F_3 \end{vmatrix} $$
Calculating the determinant will yield:
$$ \nabla \times \vec{F} = \left( \frac{\partial F_3}{\partial y} - \frac{\partial F_2}{\partial z} \right) \hat{i} - \left( \frac{\partial F_3}{\partial x} - \frac{\partial F_1}{\partial z} \right) \hat{j} + \left( \frac{\partial F_2}{\partial x} - \frac{\partial F_1}{\partial y} \right) \hat{k} $$
- Calculating each component of the curl
We calculate the necessary partial derivatives:
-
For ( \hat{i} ):
- ( \frac{\partial F_3}{\partial y} = c )
- ( \frac{\partial F_2}{\partial z} = -1 )
- Hence, ( c + 1 = 0 \Rightarrow c = -1 )
-
For ( \hat{j} ):
- ( \frac{\partial F_3}{\partial x} = 4 )
- ( \frac{\partial F_1}{\partial z} = a )
- Hence, ( 4 - a = 0 \Rightarrow a = 4 )
-
For ( \hat{k} ):
- ( \frac{\partial F_2}{\partial x} = b )
- ( \frac{\partial F_1}{\partial y} = 2 )
- Hence, ( b - 2 = 0 \Rightarrow b = 2 )
- Final values of constants
From the calculations, we find:
$$ a = 4, \quad b = 2, \quad c = -1 $$
- Finding the scalar function ( \phi )
Since ( \vec{F} = \nabla \phi ), we integrate each component:
Starting from ( F_1 ):
- ( \frac{\partial \phi}{\partial x} = x + 2y + 4z )
- Integrating gives ( \phi = \frac{x^2}{2} + 2xy + 4xz + g(y,z) )
Next, for ( F_2 ):
- ( \frac{\partial \phi}{\partial y} = 2x - 3y - z )
- Integrating gives ( \phi = 2xy - \frac{3y^2}{2} - yz + h(x,z) )
Lastly, for ( F_3 ):
- ( \frac{\partial \phi}{\partial z} = 4x - z + 2z )
- Integrating gives ( \phi = 4xz + \frac{z^2}{2} + h(x,y) )
Now combining all components and ensuring consistency leads to:
$$ \phi = \frac{x^2}{2} + 2xy + 4xz - \frac{3y^2}{2} + z^2 + C $$
The constants are ( a = 4 ), ( b = 2 ), ( c = -1 ).
The scalar function is:
$$ \phi = \frac{x^2}{2} + 2xy + 4xz - \frac{3y^2}{2} + z^2 + C $$
More Information
The conditions for a vector field to be irrotational are important in physics, especially in fluid dynamics and electrostatics. The scalar potential function ( \phi ) corresponds to the work done in a conservative field.
Tips
- Failing to correctly compute the determinants in the curl can lead to wrong constant values.
- Forgetting to integrate correctly when finding ( \phi ) or neglecting the functions of independent variables can yield an incomplete solution.
AI-generated content may contain errors. Please verify critical information