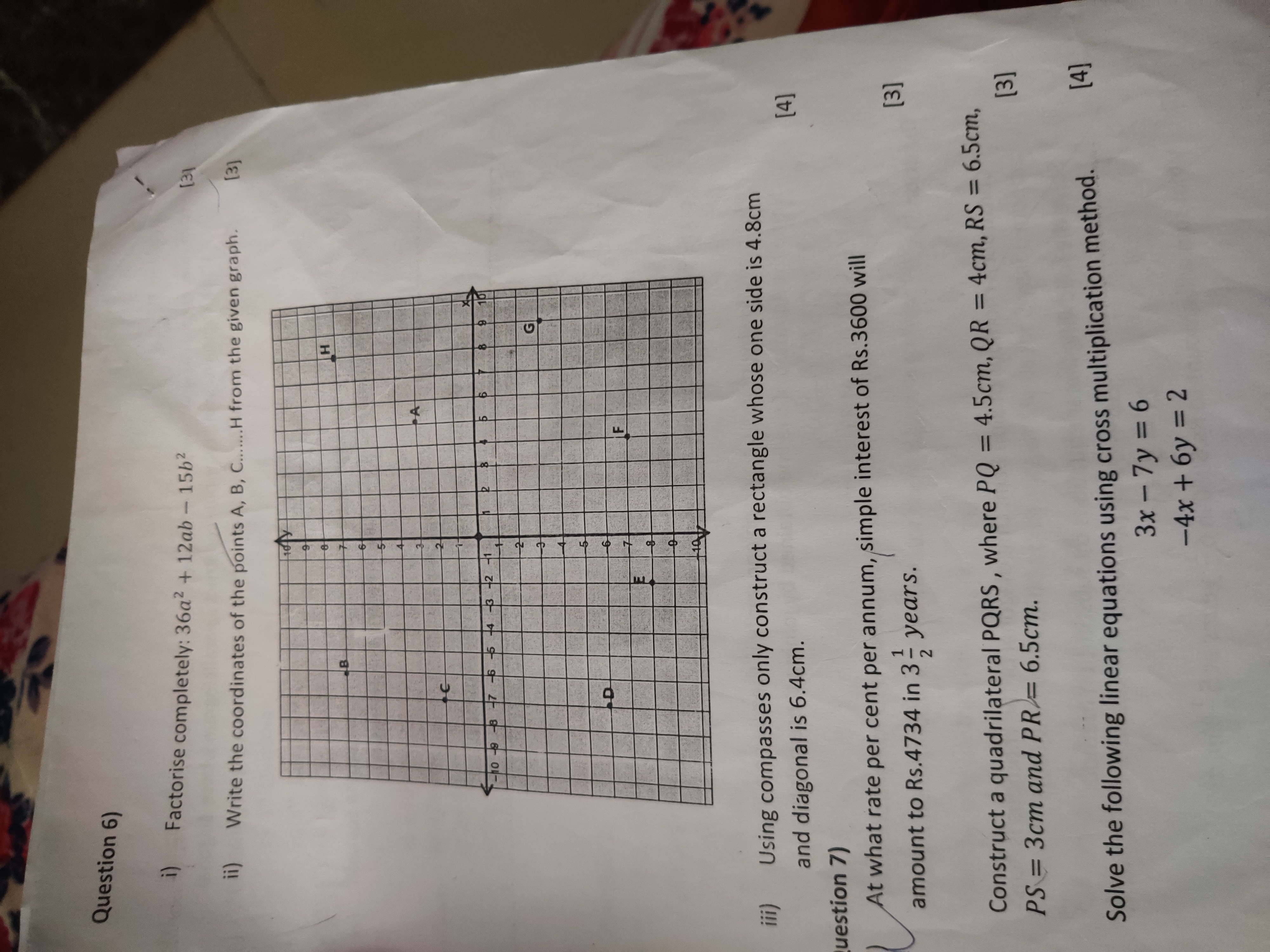
Factorise completely: 36a² + 12ab - 15b²
Understand the Problem
The image contains several math questions related to factorization, coordinate geometry, construction, finding simple interest, quadrilateral construction, and solving linear equations. We must classify each question individually.
Answer
$3(6a + 5b)(2a - b)$
Answer for screen readers
$3(6a + 5b)(2a - b)$
Steps to Solve
- Factor out the Greatest Common Divisor (GCD)
The GCD of 36, 12, and 15 is 3. Factoring out 3 from the expression, we get: $3(12a^2 + 4ab - 5b^2)$
- Factor the quadratic expression inside the parentheses
We need to find two binomials of the form $(px + qy)(rx + sy)$ such that when multiplied, they give $12a^2 + 4ab - 5b^2$. We are looking for two numbers that multiply to $12 \times -5 = -60$ and add up to $4$. These numbers are $10$ and $-6$. So, we rewrite the middle term: $12a^2 + 10ab - 6ab - 5b^2$
- Factor by grouping
Group the terms in pairs: $(12a^2 + 10ab) + (-6ab - 5b^2)$ Factor out the greatest common factor from each pair: $2a(6a + 5b) - b(6a + 5b)$
- Factor out the common binomial factor
Now we can factor out the common binomial factor $(6a + 5b)$: $(6a + 5b)(2a - b)$
- Write the final factored expression
Don't forget to include the 3 we factored out initially: $3(6a + 5b)(2a - b)$
$3(6a + 5b)(2a - b)$
More Information
Factoring is the reverse process of expanding brackets. It involves breaking down an expression into a product of simpler expressions, typically binomials or polynomials of lower degree.
Tips
- Forgetting to factor out the GCD: A common mistake is to forget to factor out the greatest common divisor (GCD) at the beginning. This can lead to a more complex factoring process and a partially factored answer.
- Incorrectly identifying the factors: Choosing the wrong numbers when factoring the quadratic expression can lead to incorrect binomial factors.
- Sign errors: Making errors with the signs of the terms while factoring can change the entire solution.
- Not fully factoring the expression: One might stop after factoring out the GCD or after a partial factorization, missing the complete factorization.
AI-generated content may contain errors. Please verify critical information