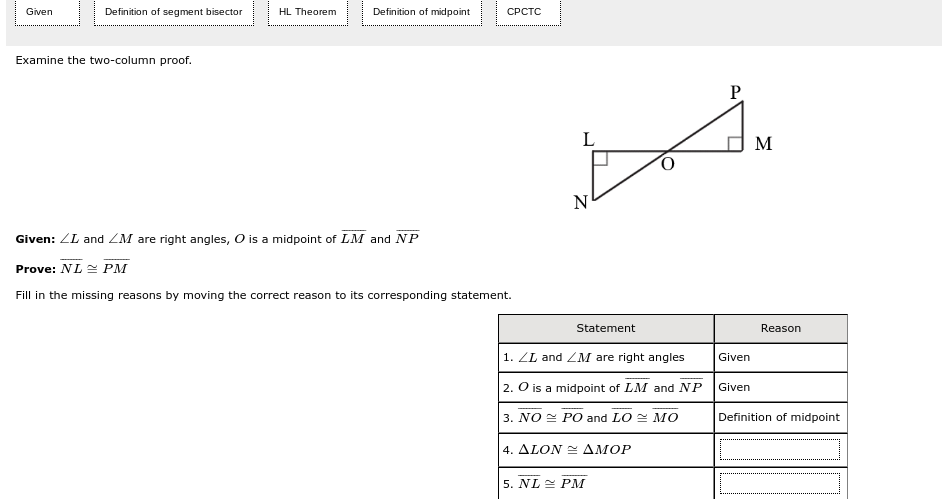
Examine the two-column proof. Given: ∠L and ∠M are right angles, O is a midpoint of LM and NP. Prove: NL ≅ PM. Fill in the missing reasons by moving the correct reason to its corre... Examine the two-column proof. Given: ∠L and ∠M are right angles, O is a midpoint of LM and NP. Prove: NL ≅ PM. Fill in the missing reasons by moving the correct reason to its corresponding statement. Read more

Steps to Solve

1. Identify the Given Information The given information states that angles $\angle L$ and $\angle M$ are right angles, and that point $O$ is the midpoint of segments $\overline{LM}$ and $\overline{NP}$.

2. Match Statement 3 with its Reason Statement 3 refers to line segments $NO \cong PO$ and $LO \cong MO$. The reason for this is the definition of the midpoint, since $O$ is a midpoint dividing both segments into two equal parts.

   • Hence, we can use Definition of midpoint for Statement 3.

3. Examine Statement 4 The congruence $\triangle LON \cong \triangle MOP$ suggests that $\angle LON \cong \angle MOP$ because both angles are right angles (from the given information). The triangle congruence can be established using the following properties: Right angles and congruent sides (from Statement 2).

   • The reason for Statement 4 is thus HL Theorem (Hypotenuse-Leg theorem) since we have right angles and legs are equal.

4. Check Statement 5 Statement 5 asserts that $NL \cong PM$. This can be concluded from the congruence of the triangles established earlier, specifically that the corresponding sides of congruent triangles are equal due to the properties of triangle congruence.

   • The reason for Statement 5 is CPCTC (Corresponding Parts of Congruent Triangles are Congruent).