Evaluate the following limit: lim (x->3) (ln(x) - ln(3))/(x^(1/3) - 3^(1/3))
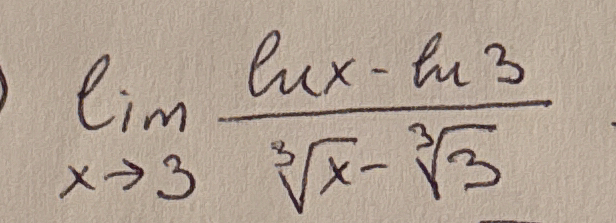
Understand the Problem
The question is asking us to evaluate a limit. This involves finding the value that the expression approaches as x approaches 3. We will need to simplify the expression and apply L'Hopital's rule. We must evaluate the limit: lim (x->3) (ln(x) - ln(3))/(x^(1/3) - 3^(1/3)).
Answer
$3^{\frac{2}{3}}$
Answer for screen readers
$3^{\frac{2}{3}}$
Steps to Solve
-
Check for Indeterminate Form First, check if we can directly substitute $x = 3$ into the expression. $$ \frac{\ln(3) - \ln(3)}{\sqrt[3]{3} - \sqrt[3]{3}} = \frac{0}{0} $$ Since we get an indeterminate form $\frac{0}{0}$, we can apply L'Hopital's Rule.
-
Apply L'Hopital's Rule L'Hopital's Rule states that if $\lim_{x\to c} \frac{f(x)}{g(x)}$ is of the form $\frac{0}{0}$ or $\frac{\infty}{\infty}$, then $$ \lim_{x\to c} \frac{f(x)}{g(x)} = \lim_{x\to c} \frac{f'(x)}{g'(x)} $$ provided the limit on the right exists.
-
Find the Derivatives Let $f(x) = \ln(x) - \ln(3)$ and $g(x) = x^{\frac{1}{3}} - 3^{\frac{1}{3}}$. Find the derivatives $f'(x)$ and $g'(x)$. $$ f'(x) = \frac{1}{x} $$ $$ g'(x) = \frac{1}{3}x^{-\frac{2}{3}} = \frac{1}{3x^{\frac{2}{3}}} $$
-
Apply L'Hopital's Rule with Derivatives Now, we have: $$ \lim_{x\to 3} \frac{\ln(x) - \ln(3)}{x^{\frac{1}{3}} - 3^{\frac{1}{3}}} = \lim_{x\to 3} \frac{\frac{1}{x}}{\frac{1}{3x^{\frac{2}{3}}}} $$
-
Simplify the Expression Simplify the expression inside the limit: $$ \lim_{x\to 3} \frac{\frac{1}{x}}{\frac{1}{3x^{\frac{2}{3}}}} = \lim_{x\to 3} \frac{3x^{\frac{2}{3}}}{x} = \lim_{x\to 3} \frac{3}{x^{\frac{1}{3}}} $$
-
Evaluate the Limit Now, substitute $x = 3$ into the simplified expression: $$ \lim_{x\to 3} \frac{3}{x^{\frac{1}{3}}} = \frac{3}{3^{\frac{1}{3}}} = \frac{3}{\sqrt[3]{3}} $$
-
Rationalize the Denominator To rationalize the denominator, multiply both the numerator and the denominator by $3^{\frac{2}{3}}$: $$ \frac{3}{3^{\frac{1}{3}}} \cdot \frac{3^{\frac{2}{3}}}{3^{\frac{2}{3}}} = \frac{3 \cdot 3^{\frac{2}{3}}}{3} = 3^{\frac{2}{3}} $$
$3^{\frac{2}{3}}$
More Information
The limit evaluates to $3^{\frac{2}{3}}$, which can also be written as $\sqrt[3]{9}$.
Tips
A common mistake is not recognizing the indeterminate form and attempting to directly substitute the value into the expression. Another common mistake is incorrectly calculating the derivatives in L'Hopital's rule.
AI-generated content may contain errors. Please verify critical information