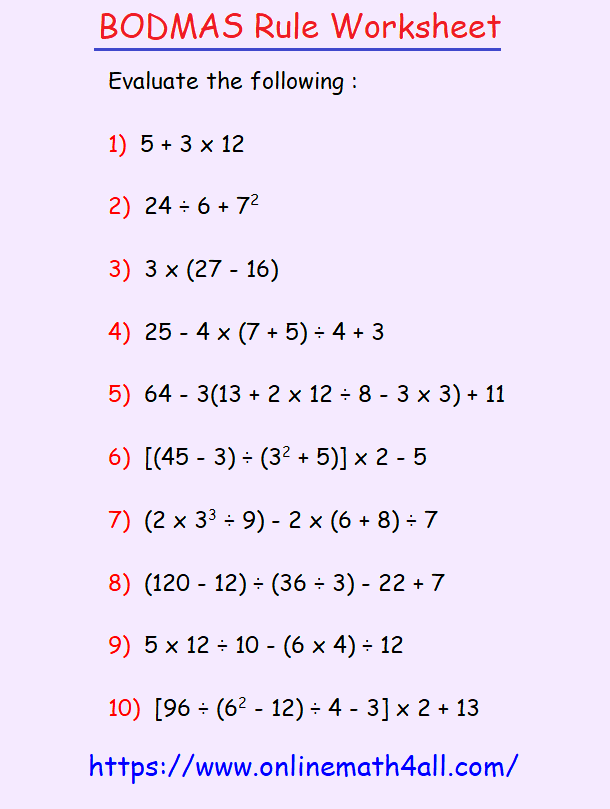
Evaluate the following expressions: 1) 5 + 3 x 12 2) 24 ÷ 6 + 7² 3) 3 x (27 - 16) 4) 25 - 4 x (7 + 5) ÷ 4 + 3 5) 64 - 3(13 + 2 x 12 ÷ 8 - 3 x 3) + 11 6) [(45 - 3) ÷ (3² + 5)] x 2 -... Evaluate the following expressions: 1) 5 + 3 x 12 2) 24 ÷ 6 + 7² 3) 3 x (27 - 16) 4) 25 - 4 x (7 + 5) ÷ 4 + 3 5) 64 - 3(13 + 2 x 12 ÷ 8 - 3 x 3) + 11 6) [(45 - 3) ÷ (3² + 5)] x 2 - 5 7) (2 x 3³ ÷ 9) - 2 x (6 + 8) ÷ 7 8) (120 - 12) ÷ (36 ÷ 3) - 22 + 7 9) 5 x 12 ÷ 10 - (6 x 4) ÷ 12 10) [96 ÷ (6² - 12) ÷ 4 - 3] x 2 + 13
Understand the Problem
The image presents a BODMAS rule worksheet with ten expressions to evaluate. The questions require applying the order of operations (Brackets, Orders, Division, Multiplication, Addition, Subtraction) to simplify and find the numerical answer for each expression.
Answer
1) 41 2) 53 3) 33 4) 16 5) 54 6) 1 7) 2 8) -6 9) 4 10) 9
Answer for screen readers
- 41
- 53
- 33
- 16
- 54
- 1
- 2
- -6
- 4
- 9
Steps to Solve
-
Evaluate expression 1: $5 + 3 \times 12$ Following BODMAS, perform multiplication before addition. $3 \times 12 = 36$ Then, $5 + 36 = 41$
-
Evaluate expression 2: $24 \div 6 + 7^2$ Following BODMAS, evaluate the exponent first. $7^2 = 49$ Then perform division: $24 \div 6 = 4$ Finally, add: $4 + 49 = 53$
-
Evaluate expression 3: $3 \times (27 - 16)$ Following BODMAS, evaluate the expression inside the parentheses first. $27 - 16 = 11$ Then multiply: $3 \times 11 = 33$
-
Evaluate expression 4: $25 - 4 \times (7 + 5) \div 4 + 3$ First, evaluate the parentheses: $7 + 5 = 12$ Then, perform multiplication and division from left to right: $4 \times 12 = 48$ $48 \div 4 = 12$ Finally, perform addition and subtraction from left to right: $25 - 12 = 13$ $13 + 3 = 16$
-
Evaluate expression 5: $64 - 3(13 + 2 \times 12 \div 8 - 3 \times 3) + 11$ First, evaluate the expression inside the parentheses. $2 \times 12 = 24$ $24 \div 8 = 3$ $3 \times 3 = 9$ $13 + 3 - 9 = 16 - 9 = 7$ Then, $3 \times 7 = 21$ Now, perform addition and subtraction from left to right: $64 - 21 = 43$ $43 + 11 = 54$
-
Evaluate expression 6: $[(45 - 3) \div (3^2 + 5)] \times 2 - 5$ First, evaluate the expressions inside the parentheses: $45 - 3 = 42$ $3^2 = 9$ $9 + 5 = 14$ Then, $42 \div 14 = 3$ $3 \times 2 = 6$ $6 - 5 = 1$
-
Evaluate expression 7: $(2 \times 3^3 \div 9) - 2 \times (6 + 8) \div 7$ First, evaluate the expressions inside the parentheses: $3^3 = 27$ $2 \times 27 = 54$ $54 \div 9 = 6$ $6 + 8 = 14$ $2 \times 14 = 28$ $28 \div 7 = 4$ Then, $6 - 4 = 2$
-
Evaluate expression 8: $(120 - 12) \div (36 \div 3) - 22 + 7$ First, evaluate the expressions inside the parentheses: $120 - 12 = 108$ $36 \div 3 = 12$ Then, $108 \div 12 = 9$ Finally, $9 - 22 + 7 = -13 + 7 = -6$
-
Evaluate expression 9: $5 \times 12 \div 10 - (6 \times 4) \div 12$ First, evaluate the expression inside the parentheses: $6 \times 4 = 24$ Then, perform multiplication and division from left to right: $5 \times 12 = 60$ $60 \div 10 = 6$ $24 \div 12 = 2$ Finally, $6 - 2 = 4$
-
Evaluate expression 10: $[96 \div (6^2 - 12) \div 4 - 3] \times 2 + 13$ First, evaluate the expression inside the parentheses: $6^2 = 36$ $36 - 12 = 24$ $96 \div 24 = 4$ $4 \div 4 = 1$ $1 - 3 = -2$ Then, $-2 \times 2 = -4$ Finally, $-4 + 13 = 9$
- 41
- 53
- 33
- 16
- 54
- 1
- 2
- -6
- 4
- 9
More Information
The problems have been evaluated by following the BODMAS order of operations.
Tips
A common mistake is not following the correct order of operations (BODMAS/PEMDAS). For example, adding before multiplying. Another common mistake is performing addition before subtraction or multiplication before division, when these operations should be performed from left to right. Similarly, the expressions within parenthesis must be simplified before carrying out any other operations. Paying close attention to each step, and writing the result after each operation can help to avoid errors.
AI-generated content may contain errors. Please verify critical information