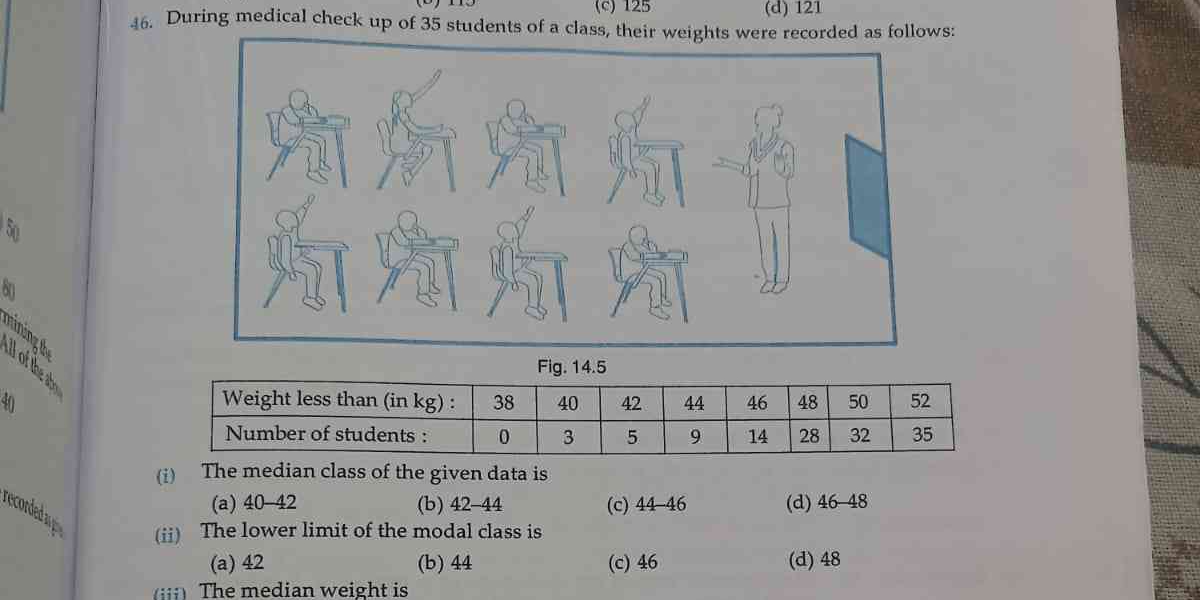
During medical check up of 35 students of a class, their weights were recorded as follows: Weight less than (in kg): 38, 40, 42, 44, 46, 48, 50, 52. Number of students: 0, 3, 5, 9,... During medical check up of 35 students of a class, their weights were recorded as follows: Weight less than (in kg): 38, 40, 42, 44, 46, 48, 50, 52. Number of students: 0, 3, 5, 9, 14, 28, 32, 35. What is the median class of the given data? (i) 40-42 (ii) 42-44 (iii) 44-46 (iv) 46-48. What is the lower limit of the modal class? (i) 42 (ii) 44 (iii) 46 (iv) 48. What is the median weight?
Understand the Problem
The question involves statistical analysis of student weights, specifically asking for the median class, the lower limit of the modal class, and the median weight based on provided frequency data. To address it, one will need to analyze the cumulative frequency distribution and apply the definitions of median and mode in statistics.
Answer
(i) (c) 44–46, (ii) (b) 50, (iii) approximately $44.11 \text{ kg}$.
Answer for screen readers
(i) The median class is (c) 44–46.
(ii) The lower limit of the modal class is (b) 50.
(iii) The median weight is approximately $ 44.11 \text{ kg} $.
Steps to Solve
- Identify Total Number of Students
The total number of students is given as 35.
- Determine the Median Class
To find the median class, we need to calculate the cumulative frequency and find the median position, which is given by:
$$ \text{Median position} = \frac{N}{2} = \frac{35}{2} = 17.5 $$
Now, we find the cumulative frequency:
- For 38: 0
- For 40: 3
- For 42: 3 + 5 = 8
- For 44: 8 + 9 = 17
- For 46: 17 + 14 = 31
- For 48: 31 + 28 = 59
- For 50: 59 + 32 = 91
- For 52: 91 + 35 = 126
The cumulative frequency just after 17.5 is at the weight 46 (31). Therefore, the median class is 44-46.
- Identify the Modal Class
The modal class is the class with the highest frequency. From the frequency data:
- 40-42: 3
- 42-44: 5
- 44-46: 9
- 46-48: 14
- 48-50: 28
- 50-52: 32
The highest frequency is 32 found in the class 50-52. Thus, the lower limit of the modal class is 50.
- Calculate the Median Weight
To find the median weight, we use the following formula:
$$ \text{Median} = L + \left(\frac{\frac{N}{2} - CF}{f}\right) \times h $$
Where:
- ( L ) = lower limit of the median class = 44
- ( N ) = total number of students = 35
- ( CF ) = cumulative frequency of the class preceding the median class = 17
- ( f ) = frequency of the median class = 9
- ( h ) = class width = 2 (from 44 to 46)
Substituting the values gives:
$$ \text{Median} = 44 + \left(\frac{17.5 - 17}{9}\right) \times 2 $$
$$ = 44 + \left(\frac{0.5}{9}\right) \times 2 $$
$$ = 44 + \frac{1}{9} $$
This yields $
\text{Median} \approx 44.11 \
$ (approximately).
(i) The median class is (c) 44–46.
(ii) The lower limit of the modal class is (b) 50.
(iii) The median weight is approximately $ 44.11 \text{ kg} $.
More Information
The median class indicates the range where the middle values lie, while the modal class shows where most data points are. The median weight provides insight into the central tendency of the dataset.
Tips
- Miscalculating cumulative frequencies can lead to errors in identifying the median class.
- Confusing the modal class with the median class; they represent different concepts.
- Forgetting to properly apply the median formula can lead to incorrect results.
AI-generated content may contain errors. Please verify critical information