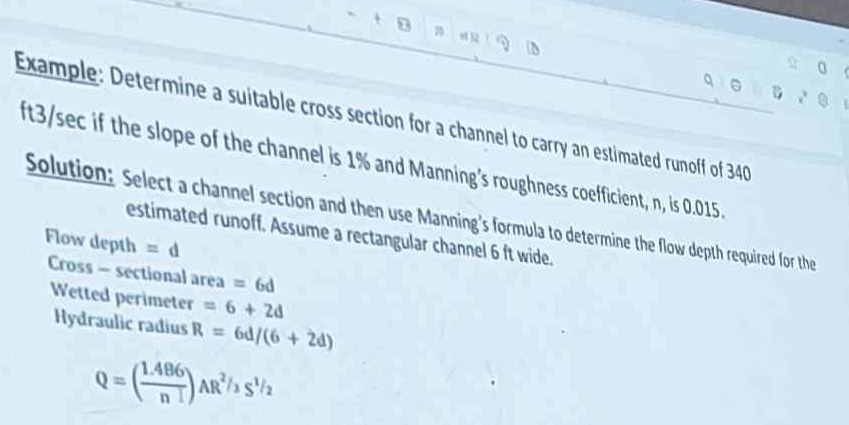
Determine a suitable cross section for a channel to carry an estimated runoff of 340 ft3/sec if the slope of the channel is 1% and Manning's roughness coefficient, n, is 0.015. Sel... Determine a suitable cross section for a channel to carry an estimated runoff of 340 ft3/sec if the slope of the channel is 1% and Manning's roughness coefficient, n, is 0.015. Select a channel section and then use Manning's formula to determine the flow depth required for the estimated runoff. Assume a rectangular channel 6 ft wide. Read more

Steps to Solve

1. State Manning's Formula

Manning's formula is given as: $Q = (\frac{1.486}{n}) AR^{2/3}S^{1/2}$

2. Substitute known variables

We are given $Q = 340 \text{ ft}^3/\text{sec}$, $n = 0.015$, $S = 1% = 0.01$.

3. Substitute geometric relationships

We also have $A = 6d$ and $R = \frac{6d}{6+2d}$. Substitute these into Manning's formula:

$340 = (\frac{1.486}{0.015}) (6d) (\frac{6d}{6+2d})^{2/3} (0.01)^{1/2}$

4. Simplify the equation

Simplify the constants: $340 = (99.067) (6d) (\frac{6d}{6+2d})^{2/3} (0.1)$ $340 = 9.9067 (6d) (\frac{6d}{6+2d})^{2/3}$ $340 = 59.44 d (\frac{6d}{6+2d})^{2/3}$

5. Isolate the term with 'd'

Divide both sides by $59.44$:

$\frac{340}{59.44} = d (\frac{6d}{6+2d})^{2/3}$ $5.72 = d (\frac{6d}{6+2d})^{2/3}$

6. Solve for d

This equation is difficult to solve analytically, so we can use numerical methods or trial and error to find the value of $d$. By inspection, we can try different values of $d$.

Let's try $d=3$: $3 (\frac{6(3)}{6+2(3)})^{2/3} = 3 (\frac{18}{12})^{2/3} = 3 (1.5)^{2/3} = 3 * 1.31 = 3.93 \neq 5.72 $

Now, let's try $d=4$: $4 (\frac{6(4)}{6+2(4)})^{2/3} = 4 (\frac{24}{14})^{2/3} = 4 (1.714)^{2/3} = 4 * 1.43 = 5.72 $

Therefore, by trial and error, $d \approx 4 \text{ ft}$.