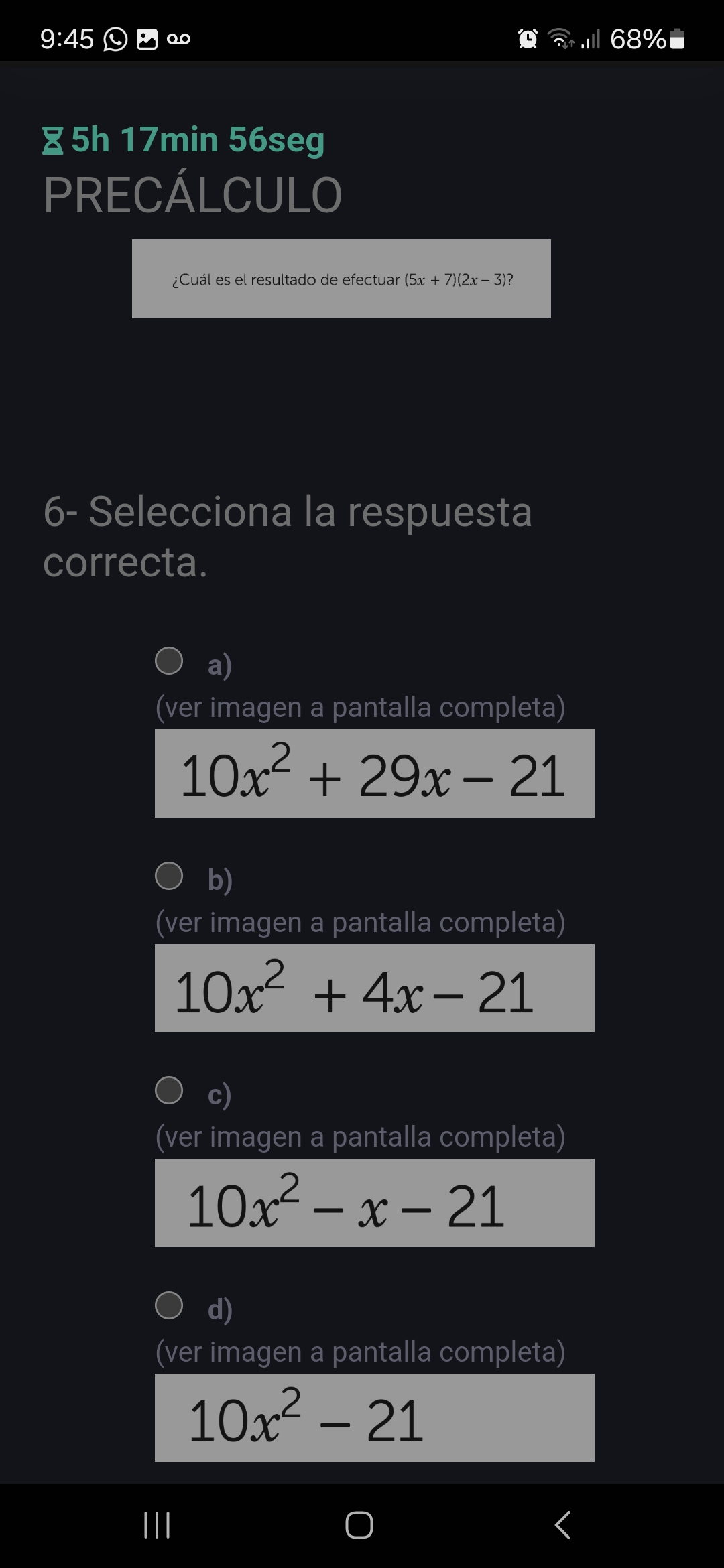
¿Cuál es el resultado de efectuar (5x + 7)(2x - 3)?
Understand the Problem
La pregunta pide encontrar el resultado de la multiplicación de dos binomios: (5x + 7) y (2x - 3). Esto implica aplicar la propiedad distributiva (a veces llamada FOIL) para multiplicar cada término del primer binomio por cada término del segundo binomio, y luego simplificar el resultado combinando términos semejantes.
Answer
$10x^2 - x - 21$
Answer for screen readers
$10x^2 - x - 21$
Steps to Solve
- Apply the distributive property (FOIL) Multiply each term in the first binomial by each term in the second binomial:
$ (5x + 7)(2x - 3) = 5x(2x) + 5x(-3) + 7(2x) + 7(-3) $
- Perform the multiplications $ 5x(2x) = 10x^2 $ $ 5x(-3) = -15x $ $ 7(2x) = 14x $ $ 7(-3) = -21 $
So we have: $ 10x^2 - 15x + 14x - 21 $
- Combine like terms Combine the $x$ terms: $-15x + 14x = -x$
So the expression simplifies to: $ 10x^2 - x - 21 $
$10x^2 - x - 21$
More Information
The result of multiplying the two binomials $(5x + 7)(2x - 3)$ is $10x^2 - x - 21$.
Tips
A common mistake is to incorrectly apply the distributive property, such as forgetting to multiply all terms or making errors with the signs. Another common mistake is to incorrectly combine like terms. Carefully check each multiplication and addition/subtraction step to avoid these errors.
AI-generated content may contain errors. Please verify critical information