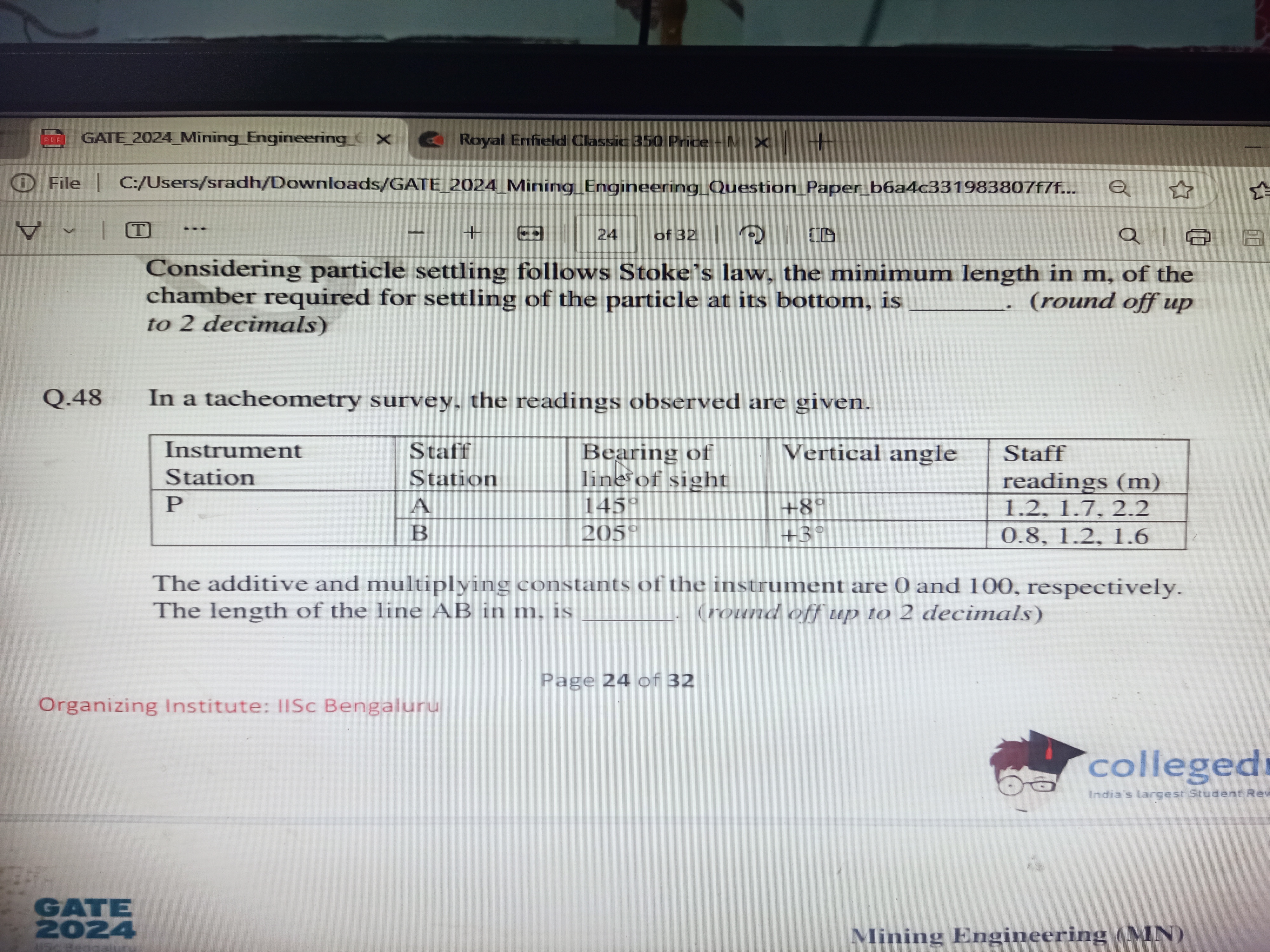
Considering particle settling follows Stokes' law, the minimum length in m of the chamber required for settling of the particle at its bottom is ______. In a tacheometry survey, th... Considering particle settling follows Stokes' law, the minimum length in m of the chamber required for settling of the particle at its bottom is ______. In a tacheometry survey, the readings observed are given. The length of the line AB in m is ______. Read more

Steps to Solve

1. Calculate Minimum Length for Particle Settling Using Stokes' Law

Stokes' law states that the settling velocity ( v ) of a particle in a fluid is given by:

$$ v = \frac{2r^2(\rho_p - \rho_f)g}{9\mu} $$

Assuming maximum settling, the chamber length can be calculated using the formula:

$$ L = \frac{v \cdot t}{g} $$

where:

• ( L ) is the minimum length of the chamber,
• ( t ) is the time taken for settling,
• ( g ) is the acceleration due to gravity (approximately 9.81 m/s(^2)).

2. Calculate Length of Line AB in Tacheometry Survey

Using the tacheometric formula, the length ( L ) between two points can be calculated using:

$$ L = \sqrt{(D^2 + H^2)} $$

Where:

• ( D ) is the horizontal distance derived from the staff readings and bearing.
• ( H ) is the vertical distance calculated using the vertical angle and the staff reading.

To find ( D ), we will use the average of the staff readings.

3. Calculate Horizontal and Vertical Components

Compute the average staff readings:

$$ \text{Average reading for A} = \frac{1.2 + 1.7 + 2.2}{3} $$ $$ \text{Average reading for B} = \frac{0.8 + 1.2 + 1.6}{3} $$

Next compute distances:

• Horizontal distance ( D ) based on angles of bearing: $$ D = \text{Average Reading} \cdot \cos(\text{Bearing Angle}) $$
• Vertical distance ( H ): $$ H = \text{Average Reading} \cdot \sin(\text{Vertical Angle}) $$

4. Calculate Overall Length

Finally, apply the square root formula:

$$ L = \sqrt{(D^2 + H^2)} $$

Substituting the calculated values will yield the answer.