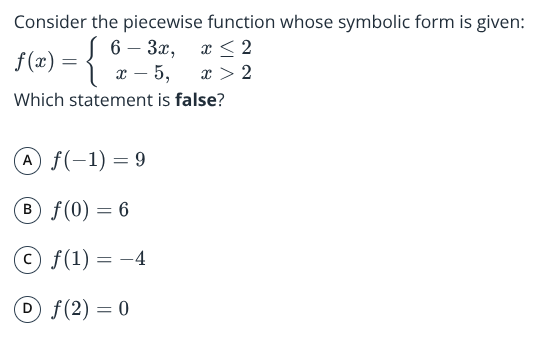
Consider the piecewise function whose symbolic form is given: f(x) = { 6 - 3x, x ≤ 2; x - 5, x > 2 } Which statement is false? A) f(-1) = 9 B) f(0) = 6 C) f(1) = -4 D) f(2) = 0

Understand the Problem

The question is asking us to evaluate a piecewise function at specific points and determine which statement about these evaluations is false. To solve this, we will substitute each value into the function and check the results.

Answer

$ f(1) = 3 $

Steps to Solve

Evaluate ( f(-1) )

Since (-1 \leq 2), we use the first piece of the function:

$$ f(-1) = 6 - 3(-1) = 6 + 3 = 9 $$

Evaluate ( f(0) )

Since (0 \leq 2), again we use the first piece of the function:

$$ f(0) = 6 - 3(0) = 6 - 0 = 6 $$

Evaluate ( f(1) )

Since (1 \leq 2), we use the first piece of the function:

$$ f(1) = 6 - 3(1) = 6 - 3 = 3 $$

Evaluate ( f(2) )

Since (2 \leq 2), we use the first piece of the function:

$$ f(2) = 6 - 3(2) = 6 - 6 = 0 $$

Determine False Statement

Now we review the results:

( f(-1) = 9 ) (True)
( f(0) = 6 ) (True)
( f(1) = 3 ) (This is different from (-4), so this statement is False)
( f(2) = 0 ) (True)

The false statement is ( f(1) = -4 ).

More Information

In piecewise functions, it's important to determine which piece of the function to use based on the given input value. Here, different intervals led to the correct evaluations confirming the false statement.

Tips

Misidentifying which piece of the function to use for a given input. Always check what the condition is for each piece to ensure you're using the correct formula.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!