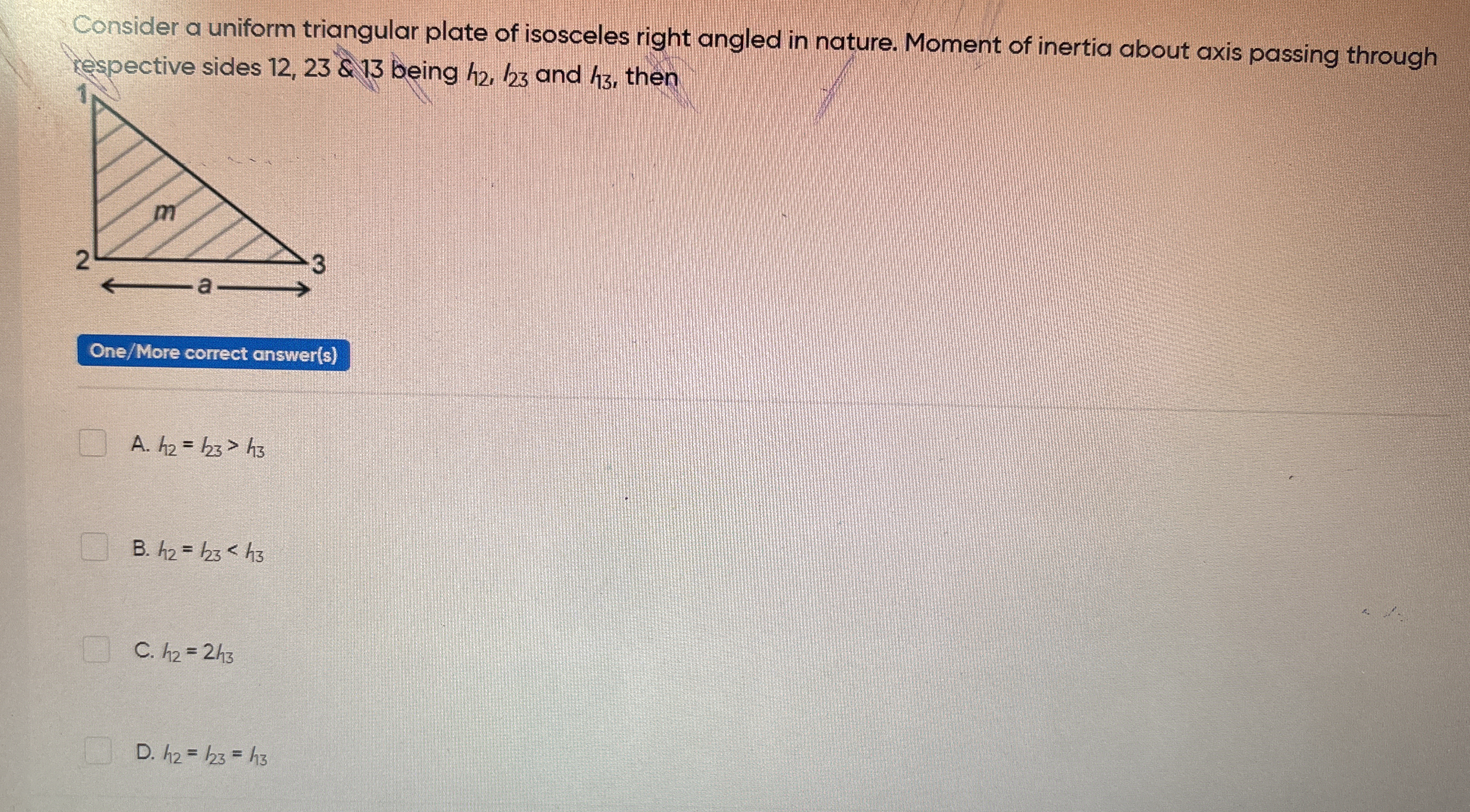
Consider a uniform triangular plate of isosceles right angled in nature. Moment of inertia about axis passing through respective sides 12, 23 & 13 being h12, h23 and h13, then.
Understand the Problem
The question is asking about the moment of inertia of a uniform isosceles right-angled triangular plate. It refers to the moments of inertia about different axes and compares them. We need to analyze the relationship between these moments of inertia, labeled as h12, h23, and h13.
Answer
B: \( h_{12} = h_{23} < h_{13} \)
Answer for screen readers
The correct answer is B: ( h_{12} = h_{23} < h_{13} ).
Steps to Solve
- Understand the axes of rotation
Identify the three axes associated with the moment of inertia:
- Axis 12 through side 12
- Axis 23 through side 23
- Axis 13 through side 13
- Use the formula for moment of inertia of the triangular plate
The moment of inertia ( I ) for a rigid body is defined with respect to an axis as: $$ I = \int r^2 , dm $$ For a uniform triangular plate, the moments of inertia about the respective axes will be calculated based on their positions and geometry.
- Moment of inertia calculations for each axis
The moment of inertia about each axis can be found using the known formulas for right-angled triangular plates.
- For axis 12, $$ h_{12} = \frac{1}{36} m b^3 = \frac{1}{36} m a^3 $$ where ( b ) is the base.
- For axis 23, $$ h_{23} = \frac{1}{36} m a^3 $$
- For axis 13, $$ h_{13} = \frac{1}{6} m a^2 $$
- Compare the moments of inertia
Now, we compare the computed values:
- From calculations:
- ( h_{12} ) relates to the length of the base.
- ( h_{23} ) has a similar relationship.
- ( h_{13} ) being maximum due to distance from the axis.
We find ( h_{12} = h_{23} < h_{13} ).
- Select the correct option(s)
Based on the relationships derived, we choose the option that reflects the comparisons established in the previous step.
The correct answer is B: ( h_{12} = h_{23} < h_{13} ).
More Information
The moment of inertia helps to understand how a shape resists rotational motion about a specific axis. In this case, the relationship stems from the geometry of isosceles right triangles, which have specific patterns in their distribution of mass relative to different axes.
Tips
- Ignoring the geometry or symmetry of the triangle can lead to incorrect conclusions about the moment of inertia.
- Forgetting to properly account for the distances in the inertia formulas may lead to inconsistencies.
AI-generated content may contain errors. Please verify critical information