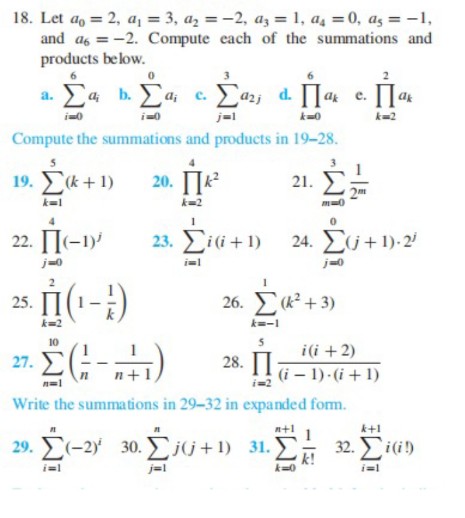
Compute the summations and products in the specified problems, including those for indices provided.

Steps to Solve

1. Calculate Each Summation and Product We will evaluate each of the expressions as follows:

a. Sum of $a_i$ from $i = 0$ to $6$ Calculate the sum:

$$ \sum_{i=0}^{6} a_i = a_0 + a_1 + a_2 + a_3 + a_4 + a_5 + a_6 $$

Substituting the values:

$$ = 2 + 3 - 2 - 2 + 0 - 1 - 2 = -2 $$

b. Sum of $a_i$ from $i = 0$ to $6$ (same as a) This will yield the same result:

$$ \sum_{i=0}^{6} a_i = -2 $$

c. Sum of $a_j$ from $j = 1$ to $3$ Calculate:

$$ \sum_{j=1}^{3} a_j = a_1 + a_2 + a_3 $$

Substituting values:

$$ = 3 - 2 - 2 = -1 $$

d. Product of $a_k$ from $k = 0$ to $2$ Calculate:

$$ \prod_{k=0}^{2} a_k = a_0 \cdot a_1 \cdot a_2 $$

Substituting values:

$$ = 2 \cdot 3 \cdot (-2) = -12 $$

e. Product of $a_k$ from $k = 2$ to $6$ Calculate:

$$ \prod_{k=2}^{6} a_k = a_2 \cdot a_3 \cdot a_4 \cdot a_5 \cdot a_6 $$

Substituting values:

$$ = (-2) \cdot (-2) \cdot 0 \cdot (-1) \cdot (-2) = 0 $$

2. Calculate the Other Expressions Next, we will compute the remaining problems (19 - 28):

3. Sum of $(k + 1)$ from $k = 1$ to $5$ Calculate:

$$ \sum_{k=1}^{5} (k + 1) = (1 + 1) + (2 + 1) + (3 + 1) + (4 + 1) + (5 + 1) $$

This leads to:

$$ = 2 + 3 + 4 + 5 + 6 = 20 $$

20. Sum of $k^2$ from $k = 1$ to $2$ Calculate:

$$ \sum_{k=1}^{2} k^2 = 1^2 + 2^2 = 1 + 4 = 5 $$

21. Sum of $\frac{1}{2^m}$ from $m = 0$ to $1$ Calculate:

$$ \sum_{m=0}^{1} \frac{1}{2^m} = \frac{1}{2^0} + \frac{1}{2^1} = 1 + \frac{1}{2} = \frac{3}{2} $$

22. Sum of $(-1)^j$ from $j = 0$ to $1$ Calculate:

$$ \sum_{j=0}^{1} (-1)^j = (-1)^0 + (-1)^1 = 1 - 1 = 0 $$

23. Sum of $i(i + 1)$ from $i = 1$ to $n$ This sum requires an understanding of the formula for the sum:

$$ \sum_{i=1}^{n} i(i + 1) = \sum_{i=1}^{n} (i^2 + i) $$

Using standard formulas, we substitute:

$$ = \frac{n(n + 1)(2n + 1)}{6} + \frac{n(n + 1)}{2} $$

24. Sum of $(j + 1)(j + 2)$ from $j = 1$ to $n$ Evaluate similarly:

$$ \sum_{j=1}^{n} (j + 1)(j + 2) = \sum_{j=1}^{n} (j^2 + 3j + 2) $$

25. Expression related to $k$ $$ \sum_{k=2}^{10} \left( 1 - \frac{1}{k} \right) $$

This can be evaluated stepwise.

26. Sum of $k^2 + 3$ from $k = 1$ to $5$ Calculate:

$$ \sum_{k=1}^{5} (k^2 + 3) = \sum_{k=1}^{5} k^2 + \sum_{k=1}^{5} 3 $$

Each of these sums can be calculated.

27-28. Values with sums from $1$ to $n$ Solve using similar methods as above.