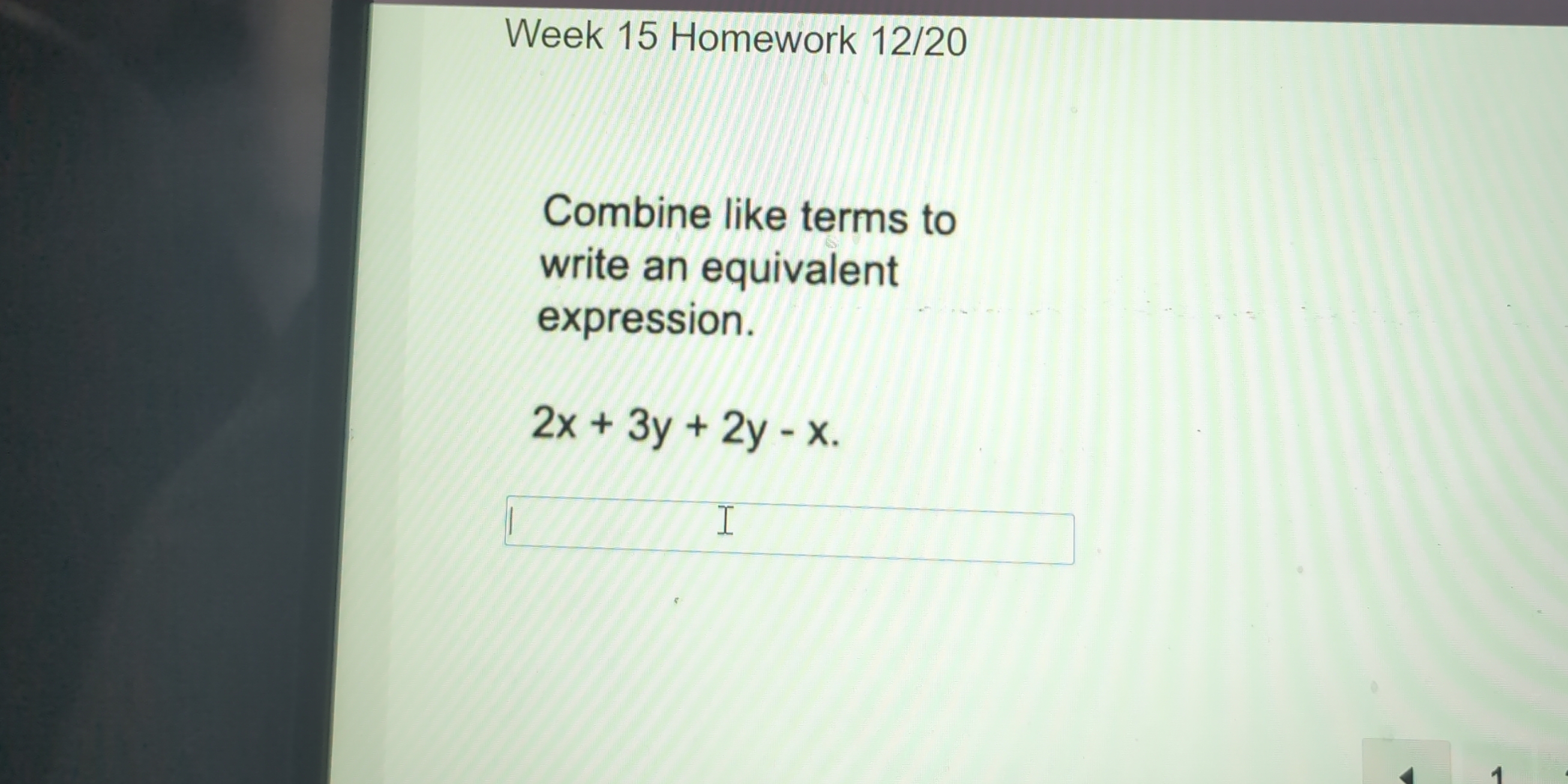
Combine like terms to write an equivalent expression: 2x + 3y + 2y - x.
Understand the Problem
The question is asking to combine like terms from the expression 2x + 3y + 2y - x to write an equivalent expression. The task involves simplifying the expression by adding or subtracting similar variables.
Answer
The equivalent expression is \( x + 5y \).
Answer for screen readers
The equivalent expression is ( x + 5y ).
Steps to Solve
- Identify the terms to combine
Look at the expression (2x + 3y + 2y - x). We need to identify like terms, which are terms that contain the same variable.
- Combine the 'x' terms
In the expression, we have (2x) and (-x). To combine them: [ 2x - x = (2-1)x = 1x \text{ or simply } x ]
- Combine the 'y' terms
Now, look at the 'y' terms: (3y) and (2y). We can combine them as follows: [ 3y + 2y = (3+2)y = 5y ]
- Write the final simplified expression
Putting together the combined terms, we get: [ x + 5y ]
The equivalent expression is ( x + 5y ).
More Information
Combining like terms is a fundamental concept in algebra that simplifies expressions, making it easier to solve equations or evaluate them later. This process allows clearer mathematical communication and clearer solutions.
Tips
- Forgetting to combine negative terms properly, such as treating (-x) as positive.
- Missing an opportunity to combine multiple like terms, which can lead to incorrect simplified forms.
AI-generated content may contain errors. Please verify critical information