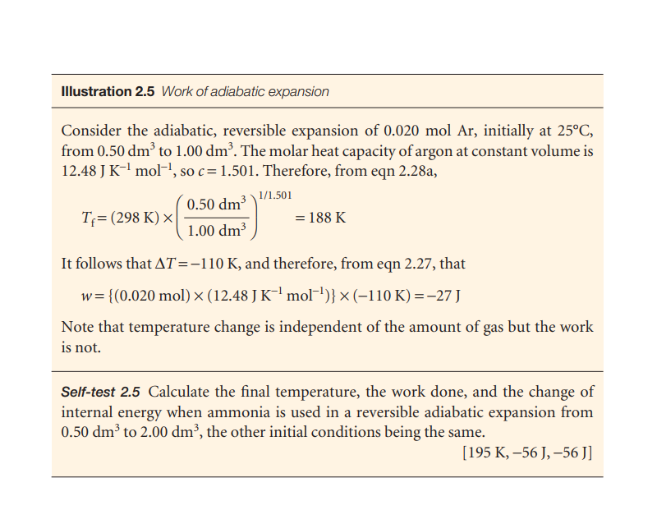
Calculate the final temperature, the work done, and the change of internal energy when ammonia is used in a reversible adiabatic expansion from 0.50 dm³ to 2.00 dm³, the other init... Calculate the final temperature, the work done, and the change of internal energy when ammonia is used in a reversible adiabatic expansion from 0.50 dm³ to 2.00 dm³, the other initial conditions being the same. Read more

Steps to Solve

1. Identify Given Data

The problem states that we have:

• Initial volume, $V_i = 0.50 , \text{dm}^3$
• Final volume, $V_f = 2.00 , \text{dm}^3$
• Initial temperature, $T_i = 25^{\circ}C = 298 , K$
• Molar heat capacity at constant volume for ammonia (we need to find this, typically around 35.06 J K$^{-1}$ mol$^{-1}$)
• Amount of substance, $n = 0.020 , \text{mol}$

2. Calculate Final Temperature ($T_f$)

Using the adiabatic condition for an ideal gas, we can express the final temperature as:

$$ T_f = T_i \left(\frac{V_i}{V_f}\right)^{c_V/(c_V - R)} $$

Assuming $R = 8.314 , \text{J K}^{-1} \text{mol}^{-1}$ and taking the specific heat of ammonia $c_V = 35.06 , \text{J K}^{-1} \text{mol}^{-1}$ gives:

$$ T_f = 298 \left(\frac{0.50}{2.00}\right)^{35.06/(35.06 - 8.314)} $$

3. Compute the Work Done ($w$)

The work done in a reversible adiabatic expansion can be calculated using the following equation:

$$ w = n \cdot c_V \cdot \Delta T $$

where $\Delta T = T_f - T_i$. Thus, we have:

$$ w = (0.020 , \text{mol}) \cdot (35.06 , \text{J K}^{-1} \text{mol}^{-1}) \cdot (T_f - 298) $$

4. Calculate Change in Internal Energy ($\Delta U$)

For an ideal gas undergoing an adiabatic process, the change in internal energy is given by:

$$ \Delta U = w $$

Thus, $\Delta U$ will equal the value obtained for $w$.

5. Final Calculations

Perform necessary calculations with the values calculated previously to find $T_f$, work done $w$, and change in internal energy $\Delta U$. Plugging in final values will yield the results.