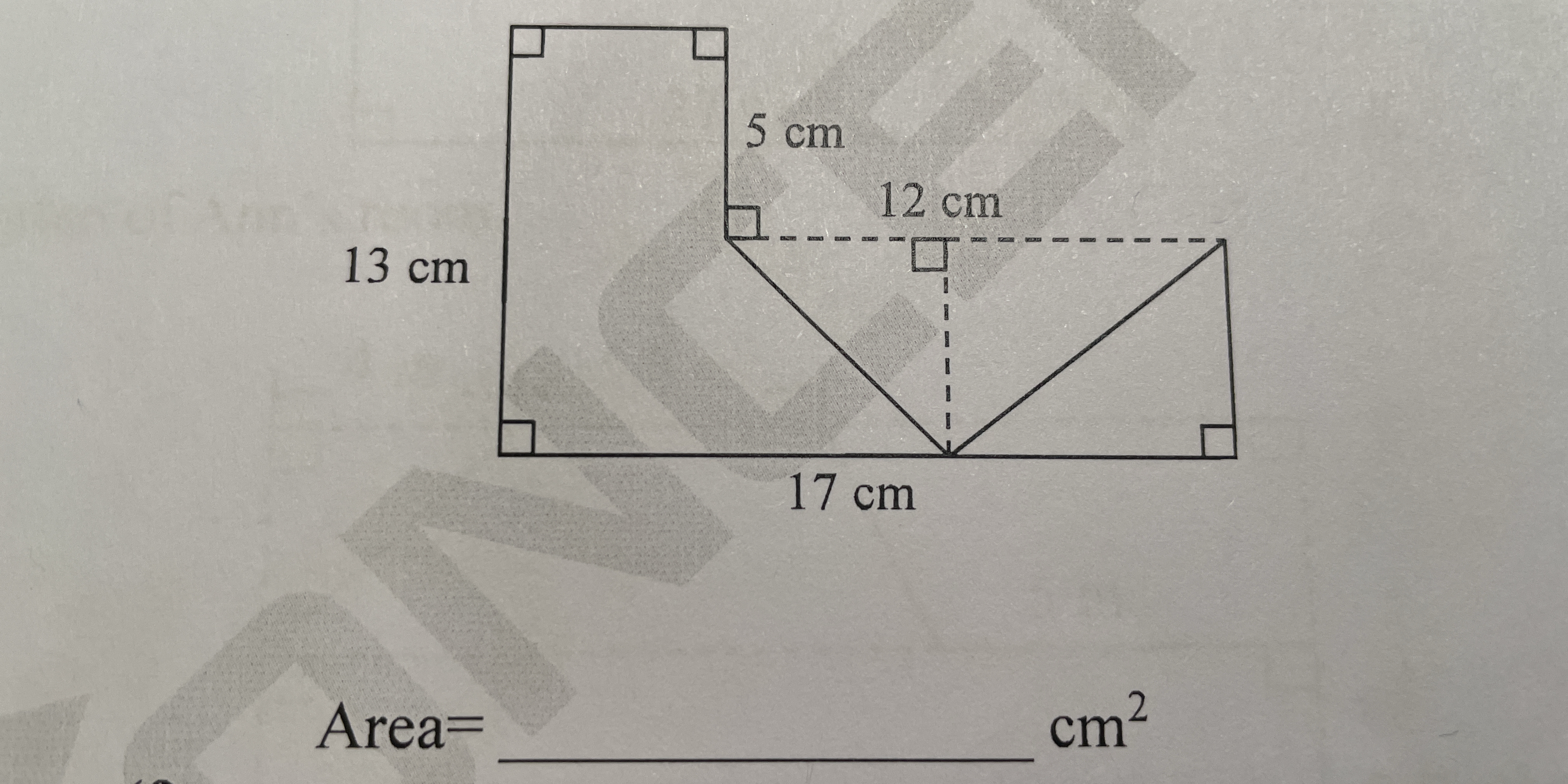
Calculate the area of the shape shown in the diagram.

Steps to Solve

1. Identify the shapes and their dimensions

We have a composite shape consisting of rectangles and a right triangle. The dimensions provided are:

• Rectangle on the left: height = 13 cm and width = 5 cm
• Rectangle on the top: height = 5 cm and width = 12 cm
• Rectangle on the right bottom: height = 12 cm and width = 17 cm
• Right triangle: base = 12 cm and height = 5 cm from the rectangles.

2. Calculate the area of the rectangles

For the left rectangle: $$ \text{Area}_{\text{left rectangle}} = \text{height} \times \text{width} = 13 , \text{cm} \times 5 , \text{cm} = 65 , \text{cm}^2 $$

For the top rectangle: $$ \text{Area}_{\text{top rectangle}} = \text{height} \times \text{width} = 5 , \text{cm} \times 12 , \text{cm} = 60 , \text{cm}^2 $$

For the right rectangle: $$ \text{Area}_{\text{right rectangle}} = \text{height} \times \text{width} = 12 , \text{cm} \times 17 , \text{cm} = 204 , \text{cm}^2 $$

3. Calculate the area of the triangle

The area of a right triangle can be calculated using the formula: $$ \text{Area}_{\text{triangle}} = \frac{1}{2} \times \text{base} \times \text{height} = \frac{1}{2} \times 12 , \text{cm} \times 5 , \text{cm} = 30 , \text{cm}^2 $$

4. Sum all areas to find the total area

Now, add all the areas together: $$ \text{Total Area} = \text{Area}{\text{left rectangle}} + \text{Area}{\text{top rectangle}} + \text{Area}{\text{right rectangle}} + \text{Area}{\text{triangle}} $$

$$ \text{Total Area} = 65 , \text{cm}^2 + 60 , \text{cm}^2 + 204 , \text{cm}^2 + 30 , \text{cm}^2 $$

$$ \text{Total Area} = 359 , \text{cm}^2 $$