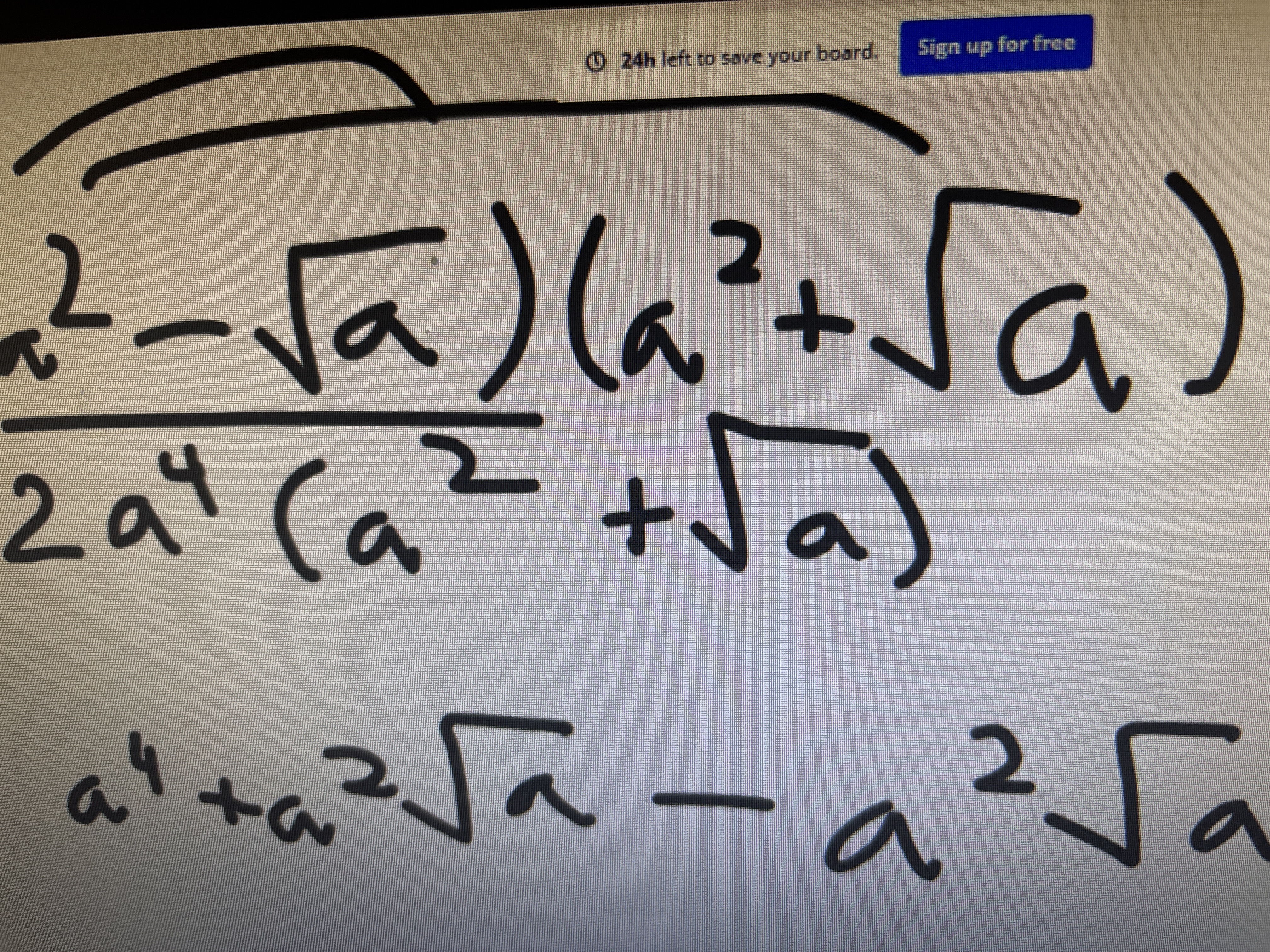
(a^2 - √a)(a^2 + √a) / (2a^4(a^2 + √a))
Understand the Problem
The question involves simplifying or solving a mathematical expression that includes variables and radical terms. The expression seems to require step-by-step manipulation to either simplify or evaluate.
Answer
The simplified expression is $$ \frac{(a - 1)(a^2 + a + 1)}{2a^3(a^2 + \sqrt{a})} $$
Answer for screen readers
The simplified expression is
$$ \frac{(a - 1)(a^2 + a + 1)}{2a^3(a^2 + \sqrt{a})} $$
Steps to Solve
- Factor the numerator
We have the expression $(a^2 - \sqrt{a})(a^2 + \sqrt{a})$. This is a difference of squares and can be simplified as follows:
$$(a^2 - \sqrt{a})(a^2 + \sqrt{a}) = a^4 - (\sqrt{a})^2 = a^4 - a$$
- Write the full expression
Now we can substitute this into our original expression:
$$ \frac{a^4 - a}{2a^4(a^2 + \sqrt{a})} $$
- Factor the numerator completely
We can factor out an $a$ from $a^4 - a$:
$$ a(a^3 - 1) $$
Thus the expression becomes:
$$ \frac{a(a^3 - 1)}{2a^4(a^2 + \sqrt{a})} $$
- Cancel common terms
Now, we can simplify by canceling the common $a$ in the numerator and denominator:
$$ = \frac{a^3 - 1}{2a^3(a^2 + \sqrt{a})} $$
- Factoring $a^3 - 1$
The term $(a^3 - 1)$ can be factored as $(a - 1)(a^2 + a + 1)$:
$$ = \frac{(a - 1)(a^2 + a + 1)}{2a^3(a^2 + \sqrt{a})} $$
- Final Expression
The simplified expression is now:
$$ \frac{(a - 1)(a^2 + a + 1)}{2a^3(a^2 + \sqrt{a})} $$
The simplified expression is
$$ \frac{(a - 1)(a^2 + a + 1)}{2a^3(a^2 + \sqrt{a})} $$
More Information
This represents the division of two polynomial expressions. The simplification involves recognizing patterns such as the difference of squares and factoring out common terms.
Tips
- Failing to factor the numerator completely before simplifying.
- Forgetting to cancel common terms in numerator and denominator.
AI-generated content may contain errors. Please verify critical information