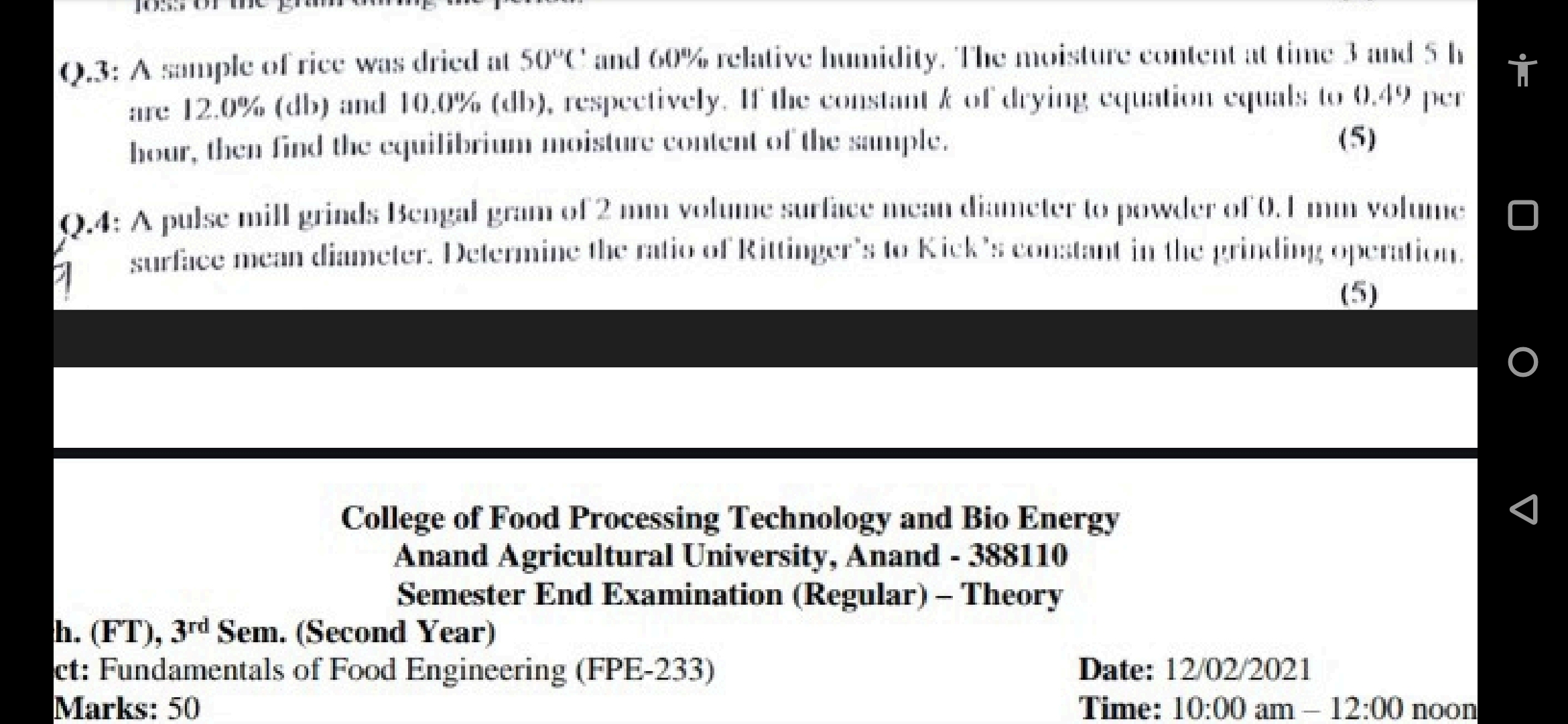
A sample of rice was dried at 50°C and 60% relative humidity. The moisture content at time 3 and 5 h are 12.0% (db) and 10.0% (db), respectively. If the constant k of drying equati... A sample of rice was dried at 50°C and 60% relative humidity. The moisture content at time 3 and 5 h are 12.0% (db) and 10.0% (db), respectively. If the constant k of drying equation equals 0.49 per hour, then find the equilibrium moisture content of the sample. A pulse mill grinds Bengal gram of 2 mm volume surface mean diameter to powder of 0.1 mm volume surface mean diameter. Determine the ratio of Rittinger's to Kick's constant in the grinding operation. Read more

Steps to Solve

1. Identify Variables and Constants

   The given values are:

   • Moisture content at 3 hours, $M_3 = 12.0%$ (db)
   • Moisture content at 5 hours, $M_5 = 10.0%$ (db)
   • Drying constant, $k = 0.49 , \text{hr}^{-1}$

   We need to find the equilibrium moisture content, $M_e$.

2. Use the Drying Equation

   The drying equation can be expressed as:

   $$ M_t = M_e + (M_0 - M_e) e^{-kt} $$

   We will use the moisture contents at times $t = 3$ hours and $t = 5$ hours.

3. Set Up Two Equations

   For $M_3$:

   $$ 12.0 = M_e + (M_0 - M_e)e^{-0.49 \cdot 3} $$

   For $M_5$:

   $$ 10.0 = M_e + (M_0 - M_e)e^{-0.49 \cdot 5} $$

   Here, $M_0$ is the initial moisture content.

4. Rearranging the Equations

   Rearranging gives us:

   For the first equation:

   $$ (M_0 - M_e)e^{-1.47} = 12.0 - M_e $$

   For the second equation:

   $$ (M_0 - M_e)e^{-2.45} = 10.0 - M_e $$

5. Eliminate Initial Moisture Content

   From both equations, we can isolate the expression for $M_0 - M_e$:

   Let:

   • $C_3 = 12.0 - M_e$
   • $C_5 = 10.0 - M_e$

   This allows us to express:

   $$ \frac{C_3}{C_5} = \frac{e^{-1.47}}{e^{-2.45}} $$

6. Solve for $M_e$

   Setting these equal gives:

   $$ C_3 e^{1.98} = C_5 $$

   Substitute back to find $M_e$. You will have:

   $$ 12.0 - M_e = e^{1.98} (10.0 - M_e) $$

7. Final Calculation for $M_e$

   Solving this will give you the equilibrium moisture content $M_e$.

8. Calculate the Grinding Ratio

   The grinding ratio from Rittinger’s constant $R_r$ and Kick's constant $R_k$ relates to particle size reduction. Use the formula:

   $$ \frac{R_r}{R_k} = \frac{d_1}{d_2} $$

   Here, $d_1 = 2 \text{ mm}$ and $d_2 = 0.1 \text{ mm}$

   Substitute to find the ratio.