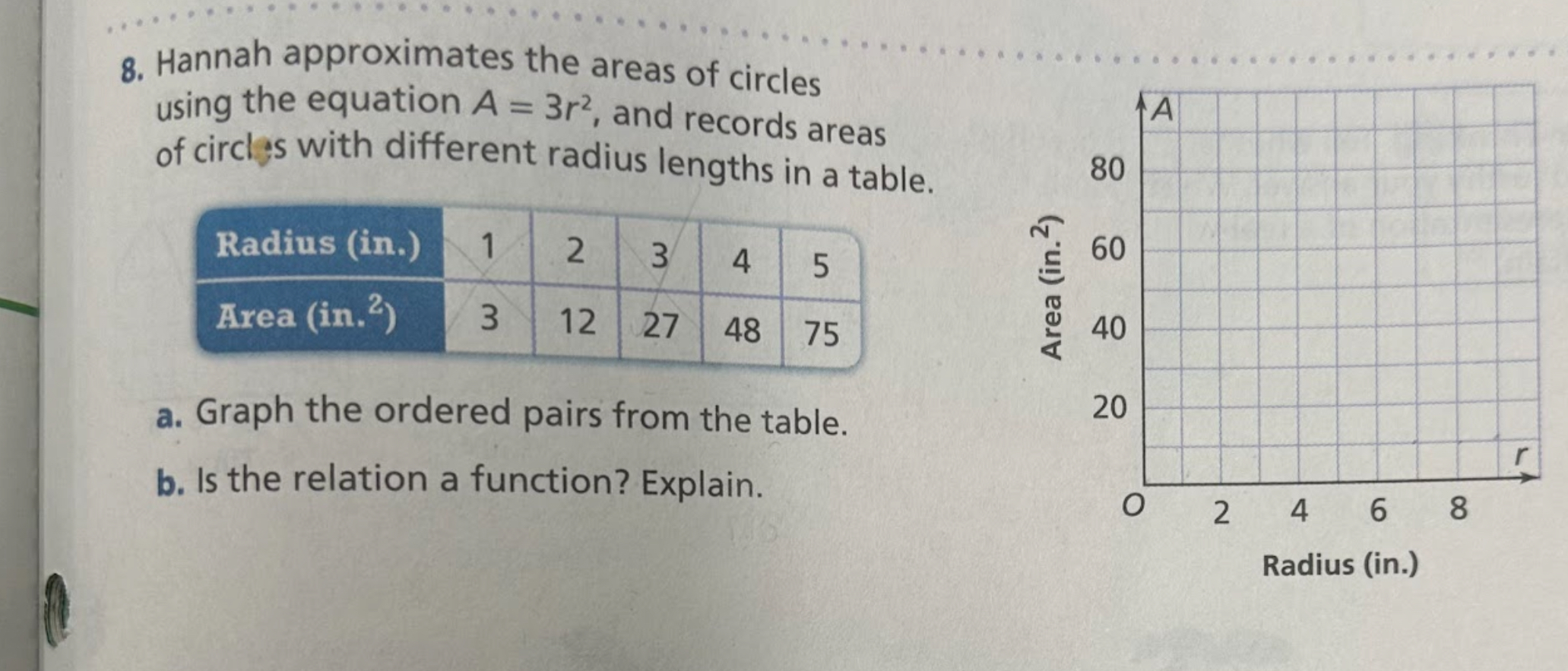
a. Graph the ordered pairs from the table. b. Is the relation a function? Explain.
Understand the Problem
The question requires us to graph the ordered pairs from a table showing the relationship between the radius of circles and their areas. It also asks to determine if the relationship defined by the table is a function and to explain why.
Answer
Yes, the relation is a function since each radius corresponds to exactly one area.
Answer for screen readers
Yes, the relation is a function since each radius corresponds to exactly one area.
Steps to Solve
- Prepare the Ordered Pairs From the table, we can extract the ordered pairs that represent the relationship between the radius and the area of the circles. The pairs are:
- (1, 3)
- (2, 12)
- (3, 27)
- (4, 48)
- (5, 75)
-
Graph the Ordered Pairs On a grid, plot these points. The x-axis will represent the radius (in inches) and the y-axis will represent the area (in square inches).
-
Plot each point
- For (1, 3): Start at (1, 3).
- For (2, 12): Move to (2, 12).
- For (3, 27): Plot at (3, 27).
- For (4, 48): Place a point at (4, 48).
- For (5, 75): Finally, plot (5, 75).
-
Determine if the Relation is a Function A relation is a function if each input (x-value, in this case, the radius) corresponds to exactly one output (y-value, the area).
-
Check for Multiple Outputs Here, each radius value has one unique area value; therefore, each radius corresponds to only one area. This confirms that the relation is indeed a function.
Yes, the relation is a function since each radius corresponds to exactly one area.
More Information
The relationship between radius and area in circles is defined by the formula $A = 3r^2$, where $A$ is the area and $r$ is the radius. This is a variation based on a constant, showing the proportionality in growth as the radius increases.
Tips
null
AI-generated content may contain errors. Please verify critical information