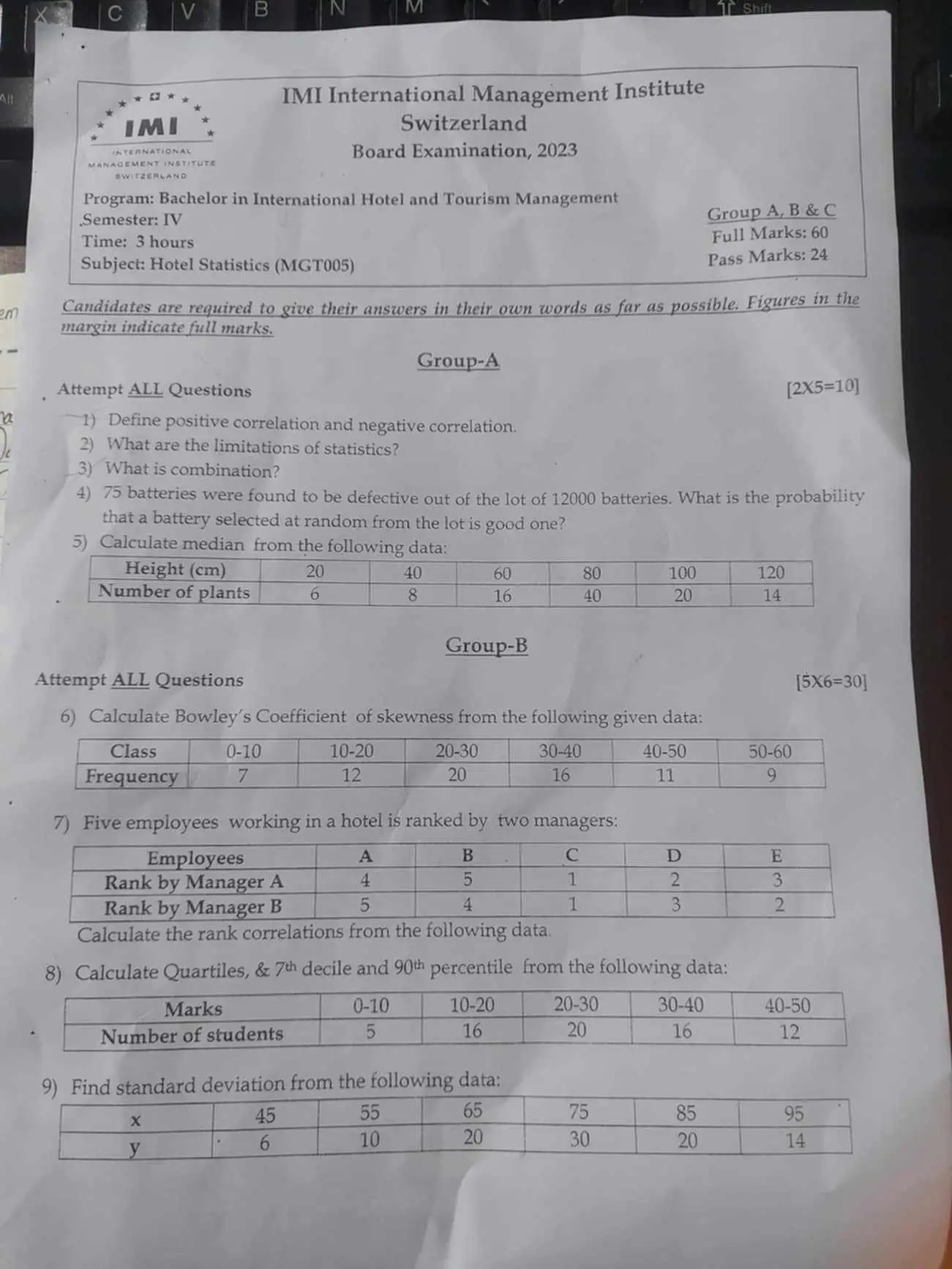
1) Define positive correlation and negative correlation. 2) What are the limitations of statistics? 3) What is combination? 4) 75 batteries were found to be defective out of the lo... 1) Define positive correlation and negative correlation. 2) What are the limitations of statistics? 3) What is combination? 4) 75 batteries were found to be defective out of the lot of 12000 batteries. What is the probability that a battery selected at random from the lot is good one? 5) Calculate median from the following data: Height (cm): 20, 40, 60, 80, 100, 120; Number of plants: 8, 16, 4, 2, 0, 14. 6) Calculate Bowley's Coefficient of skewness from the following given data: Class 0-10, 10-20, 20-30, 30-40, 40-50, 50-60; Frequency: 7, 12, 20, 16, 11, 9. 7) Five employees working in a hotel are ranked by two managers. Calculate the rank correlations from the given data. 8) Calculate Quartiles, & 7th decile and 90th percentile from the following data: Marks 0-10, 10-20, 20-30, 30-40, 40-50; Number of students: 5, 16, 20, 16, 12. 9) Find standard deviation from the following data: x: 45, 55, 65, 75, 85, 95; y: 6, 10, 20, 30, 20, 14.
Understand the Problem
The questions are focused on statistics and include defining key concepts, calculating probabilities and other statistical measures, and analyzing data sets related to hotel management.
Answer
The probability that a battery selected at random from the lot is good is $0.99375$.
Answer for screen readers
The probability that a battery selected at random from the lot is good is $0.99375$.
Steps to Solve
-
Determine the Total Number of Batteries The total number of batteries is given as 12,000.
-
Identify the Number of Defective Batteries It states that out of these, 75 batteries are defective.
-
Calculate the Number of Non-Defective Batteries To find the good batteries, subtract the defective ones from the total: $$ \text{Good Batteries} = 12000 - 75 = 11925 $$
-
Calculate the Probability of Selecting a Good Battery The probability is calculated as the ratio of good batteries to the total number of batteries: $$ P(\text{Good Battery}) = \frac{\text{Good Batteries}}{\text{Total Batteries}} = \frac{11925}{12000} $$
-
Simplify the Probability Calculating the probability gives: $$ P(\text{Good Battery}) = 0.99375 $$
The probability that a battery selected at random from the lot is good is $0.99375$.
More Information
This means that there is a 99.375% chance that a randomly chosen battery will be non-defective. This high probability reflects the overall quality of the lot, as only a small fraction is defective.
Tips
- Confusing Good and Defective Counts: Make sure to correctly identify the good batteries by subtracting defects from the total.
- Miscalculating Probability: Always remember that probability is a ratio of favorable outcomes to total outcomes.
AI-generated content may contain errors. Please verify critical information