Statistics and Probability: Scatter Plots

• Study notes for Statistics and Probability

Describing Relationships Using Scatter Plot

• Univariate data involves a single variable.
• Bivariate data involves two variables.
• Correlation analysis describes the relationship between two variables, using a statistical method to determine if a relationship exists.
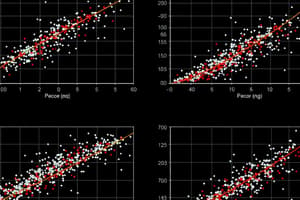
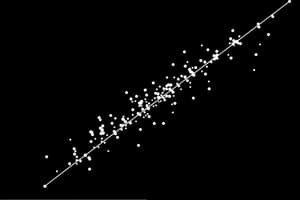
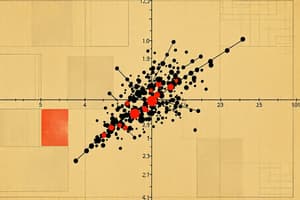
• A scatter plot graphically represents the relationship between two variables, using ordered pairs to determine if a relationship exists.
• A trend line (or line of best fit) goes through a scatter plot's data points and expresses the relationship between the points which are closest to said trend line.

Correlation Description

• Correlation can be described by strength (perfect, high, moderately high/low, low, or zero/no) and direction (positive, negative, or zero/no).

Types of Correlation According to Direction

• Positive correlation exists if high values in one variable are associated with high values in another variable, and low values are associated with low values.
• With positive correlation, as one variable increases, the other increases, and the trend line slants up to the right.
• Negative correlation exists if high values in one variable are associated with low values in another variable, and low values are associated with high values.
• With negative correlation, as one variable increases, the other decreases, and the trend line slants down to the right.
• Zero/no correlation exists if high values in one variable are associated with high or low values in the other variable, showing no relationship and no trend line.

Types of Correlation According to Strength

• Perfect correlation exists when all points on the scatter plot lie on a straight line which is a controlled variable.
• If the points on a scatter plot don't follow a straight line, the relationship may be very high, high, moderately high, low, negligible, or zero.
• Further categorizations include perfect positive, high positive, moderately high positive/negative, high negative, and perfect negative correlations.

Exploring the Pearson Product-Moment Correlation

• Correlation coefficient (r) describes the strength and direction of the relationship between two variables, ranging from -1 to +1.
• r = 1 means perfect positive relationship, r = -1 means perfect negative relationship, r = 0 means no relationship.

Values of r and their interpretations

• -1: Perfect Negative Correlation
• 1: Perfect Positive Correlation
• 0: No Correlation
• 0.80 to 0.99: Very High Positive Correlation
• 0.60 to 0.79: High Positive Correlation
• 0.40 to 0.59: Moderately Positive Correlation
• 0.20 to 0.39: Low Positive Correlation
• 0.01 to 0.19: Negligible Positive Correlation
• -0.01 to -0.19: Negligible Negative Correlation
• -0.20 to -0.39: Low Negative Correlation
• -0.40 to -0.59: Moderately Negative Correlation
• -0.69 to -0.79: High Negative Correlation
• -0.80 to -0.99: Very High Negative Correlation

Exploring Regression Analysis

• Regression analysis predicts the value of one variable based on another.
• Simple linear regression deals with one dependent and one independent variable.
• Multiple linear regression deals with more than one independent variable.

Components of a Regression Analysis

• Dependent variable (y) is the "response variable."
• Independent variable (x) is the "predictor variable" or "explanatory variable."
• A regression line is a straight line that best fits a set of data points.
• A regression equation is the equation of the regression line.
• The Y-intercept of the Regression Equation is the predicted value of the dependent variable when the independent variable is zero.
• Predicting one variable requires getting the regression equation, represented graphically as a line, assuming a linear relationship.
• The formula for this is shown as Y’ = b0X + b1
• Where
  • Y’: predicted value
  • X: value of the independent variable
  • b0: slope of the regression line
  • b1: y-intercept of the regression line
• Find the slope of the line with: b0= 𝑛∑𝑋𝑌 − ∑𝑋∑ / 𝑛∑𝑋² − (∑𝑋)²
• Find the y-intercept of the line with: b1= ∑𝑌 − 𝑏 0 ∑𝑋 / 𝑛

Normal Probability Distribution

• Probability distribution assigns probabilities to the values of a random variable and is equal to the area under the curve.
• Discrete random variable has a countable set of possible outcomes.
• Continuous random variable takes on values from within an interval, or disjoint union of intervals.
• Probability density function is a frequency function or a probability distribution.
• The curve of a continuous random variable is the function of X denoted by f(X).
• The mathematical equation of the normal distribution is 𝑓(𝑥) = 1 /σ 2π * 𝑒 −2(𝑋−µ/σ)^2

Normal Distribution Aspects

• μ = mean
• σ = standard deviation
• Raw Score that Corresponds to each Z-score Value is X = μ ± 𝑧σ

Normal Distribution

• The normal distribution is a "bell curve" or "Gaussian Distribution" (developed by Carl Friedrich Gauss).
• It calculates probabilities concerning a population.
• Abraham de Moivre developed the mathematical equation for the normal curve.
• Properties of the normal distribution include being bell-shaped, symmetric, having mean = median = mode, having tail ends asymptotic to the base line, a shape dependent upon the mean and standard deviation, and a total area of 1 or 100% (50% less/greater than the mean).

Standardizing a Normal Probability Distribution

• Standardizing involves converting the X-scale to the z-scale
• This follows the formula: 𝑧 = 𝑥−µ / σ
• Where X = value of the variable