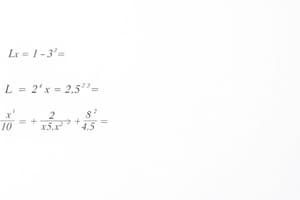Rational Functions: Asymptotes, Operations, Solving Equations, Graphing, Domain & Range

Study Notes

Rational Functions

Rational functions represent a category of mathematical expressions where the numerator and denominator contain polynomial terms. They play a significant role in various areas such as algebra, calculus, physics, engineering, economics, and computer science. In this article, we'll explore different aspects of rational functions, including their asymptotes, operations, solving equations, graphing techniques, and domain and range considerations.

Asymptotes

An asymptote is a line that the graph of a function approaches arbitrarily close but never meets. For rational functions, there are two types of asymptotes: horizontal and vertical. A horizontal asymptote occurs when the degree of the numerator equals the degree of the denominator, while a vertical asymptote occurs when the degree of the numerator exceeds the degree of the denominator by one unit.

For example, let's look at the expression f(x) = (x^2 + 1) / (x - 2). This function has a horizontal asymptote y = 1 since the degrees of both the numerator and the denominator are equal (2), and it has a vertical asymptote x = 2 because the denominator (x - 2) becomes zero.

Operations with Rational Functions

Several arithmetic operations can be applied to rational functions, including addition, multiplication, division, and composition. Adding two rational functions involves adding their respective coefficients and changing the signs if necessary. When multiplying rational functions, we follow the same pattern for like powers of x, considering the highest power present. Division can also be performed between two rational functions. However, before performing these operations, it's crucial to ensure that the domains of the functions overlap so that the operation can be defined over all common values of x.

Solving Rational Equations

Solving rational equations involves manipulating the given equation and simplifying it to obtain the solution. There are several techniques to solve these equations, including cross-multiplication, substitution, and elimination methods. The key is to first rewrite the equation in the form of a = b / c, where c ≠ 0. This allows us to perform the necessary operations to isolate x and obtain a value for it.

Graphing Techniques

Graphing rational functions can be done using transformations of the basic rational function y = 1 / x. This involves identifying the number of zeros, vertical asymptotes, horizontal asymptotes, and the transformations required to obtain the given function's graph. Additionally, graphing calculators and software can be used to visualize the function and identify its key features.

Domain and Range

The domain of a rational function is the set of all real numbers for which the denominator is not zero. This means that we exclude the values of x that make the denominator zero, as it would result in a division by zero error. The range, on the other hand, is the set of all possible outputs of the function as x varies over its domain. It's important to note that the range of a rational function is infinite, as it can take any real number as a value.

In conclusion, rational functions are a vital concept in mathematics with various applications. Understanding their properties, such as asymptotes, operations, solving equations, graphing techniques, and domain and range, can help us better appreciate their importance and versatility in solving real-world problems.

Description

Explore the fundamental concepts of rational functions, including asymptotes, arithmetic operations, solving equations, graphing techniques, and considerations of domain and range. Learn about horizontal and vertical asymptotes, various operations with rational functions, solving techniques, graphing methods, and the domain and range restrictions for these functions.