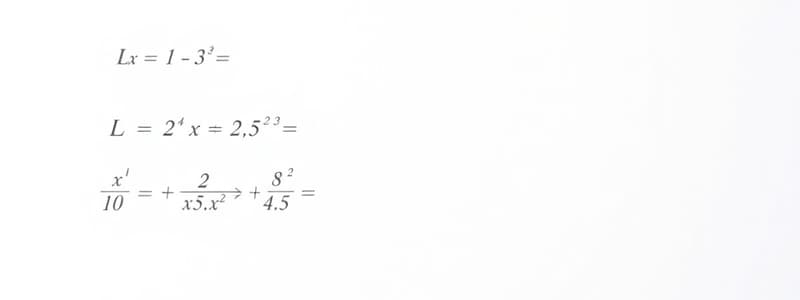Podcast
Questions and Answers
What is the left limit of the function 𝑓(𝑥) as 𝑥 approaches 1 for the expression (𝑥 − 1)(𝑥 + 5)?
What is the left limit of the function 𝑓(𝑥) as 𝑥 approaches 1 for the expression (𝑥 − 1)(𝑥 + 5)?
- 3
- 2 (correct)
- −∞
- 0
What type of discontinuity is present at 𝑥 = 1 for the function 𝑓(𝑥) = (𝑥 − 1)(𝑥 + 2)?
What type of discontinuity is present at 𝑥 = 1 for the function 𝑓(𝑥) = (𝑥 − 1)(𝑥 + 2)?
- Hole (correct)
- Removable discontinuity
- Jump discontinuity
- Vertical asymptote
What is the left end behavior of the function $f(x)$ as $x$ approaches 1?
What is the left end behavior of the function $f(x)$ as $x$ approaches 1?
- lim $f(x)$ = 1 as $x$ → 1 (correct)
- lim $f(x)$ = 2 as $x$ → 1
- lim $f(x)$ = 0 as $x$ → 1
- lim $f(x)$ = -1 as $x$ → 1
What is the right limit of the function 𝑓(𝑥) = (𝑥 − 2)(𝑥 − 4) as 𝑥 approaches 1?
What is the right limit of the function 𝑓(𝑥) = (𝑥 − 2)(𝑥 − 4) as 𝑥 approaches 1?
What is the right end behavior of the function $g(x)$ as $x$ approaches 2?
What is the right end behavior of the function $g(x)$ as $x$ approaches 2?
For the rational function 𝑓(𝑥) = −2(𝑥 + 3)(𝑥 + 1), what determines the behavior of the limit as 𝑥 approaches 1?
For the rational function 𝑓(𝑥) = −2(𝑥 + 3)(𝑥 + 1), what determines the behavior of the limit as 𝑥 approaches 1?
What is the left end behavior of the function $k(x)$ as $x$ approaches 1?
What is the left end behavior of the function $k(x)$ as $x$ approaches 1?
As 𝑥 approaches 1 for 𝑓(𝑥) = (𝑥 − 1)(𝑥 + 2), what happens to the function's value?
As 𝑥 approaches 1 for 𝑓(𝑥) = (𝑥 − 1)(𝑥 + 2), what happens to the function's value?
What is the right limit of 𝑓(𝑥) = −2(𝑥 + 3)(𝑥 + 1) as 𝑥 approaches 1?
What is the right limit of 𝑓(𝑥) = −2(𝑥 + 3)(𝑥 + 1) as 𝑥 approaches 1?
What is the right end behavior of the function $h(x)$ as $x$ approaches 3?
What is the right end behavior of the function $h(x)$ as $x$ approaches 3?
What can be concluded about the function 𝑓(𝑥) = (𝑥 − 1)(𝑥 + 2) at the point 𝑥 = 1?
What can be concluded about the function 𝑓(𝑥) = (𝑥 − 1)(𝑥 + 2) at the point 𝑥 = 1?
What is the left end behavior of the function $r(x)$ as $x$ approaches 2?
What is the left end behavior of the function $r(x)$ as $x$ approaches 2?
What is the nature of the right limit of the function 𝑓(𝑥) as 𝑥 approaches 1 for (𝑥 − 2)(𝑥 − 4)?
What is the nature of the right limit of the function 𝑓(𝑥) as 𝑥 approaches 1 for (𝑥 − 2)(𝑥 − 4)?
What is the limit of 𝑦 as 𝑥 approaches 2?
What is the limit of 𝑦 as 𝑥 approaches 2?
Which value indicates a hole in the function 𝑘(𝑥)?
Which value indicates a hole in the function 𝑘(𝑥)?
What is the vertical asymptote of the function 𝑟(𝑥)?
What is the vertical asymptote of the function 𝑟(𝑥)?
For which inequality does the solution set result in (−2,3]?
For which inequality does the solution set result in (−2,3]?
What is the limit of function $f(x)$ as the input values decrease without bound?
What is the limit of function $f(x)$ as the input values decrease without bound?
What is the limit of 𝑟(𝑥) as 𝑥 approaches -1?
What is the limit of 𝑟(𝑥) as 𝑥 approaches -1?
Which of the following expressions indicates a vertical asymptote for 𝑦?
Which of the following expressions indicates a vertical asymptote for 𝑦?
What is the limit of function $g(x)$ as the input values increase without bound?
What is the limit of function $g(x)$ as the input values increase without bound?
What is the limit of function $h(x)$ as the input approaches a specific value from the left?
What is the limit of function $h(x)$ as the input approaches a specific value from the left?
When does the function 𝑘(𝑥) approach a limit of zero?
When does the function 𝑘(𝑥) approach a limit of zero?
For 𝑦 = (𝑥 + 3)/(𝑥 − 2), what value of 𝑥 creates a vertical asymptote?
For 𝑦 = (𝑥 + 3)/(𝑥 − 2), what value of 𝑥 creates a vertical asymptote?
What can be concluded about the limit of function $k(x)$ as the input approaches a specific value from the left?
What can be concluded about the limit of function $k(x)$ as the input approaches a specific value from the left?
What is the limit of function $h(x)$ as the input approaches infinity?
What is the limit of function $h(x)$ as the input approaches infinity?
What is the limit of function $k(x)$ as the input approaches infinity?
What is the limit of function $k(x)$ as the input approaches infinity?
Which limit correctly describes the behavior of function $h(x)$ as the input approaches a specific value from the right?
Which limit correctly describes the behavior of function $h(x)$ as the input approaches a specific value from the right?
What is the limit of function $k(x)$ when the input approaches the same specific value from both left and right?
What is the limit of function $k(x)$ when the input approaches the same specific value from both left and right?
What is the domain of the rational function represented by $\frac{x + 2}{(x + 1)}$?
What is the domain of the rational function represented by $\frac{x + 2}{(x + 1)}$?
Which interval represents where the expression $x^2 - x - 12 \geq 0$ is true?
Which interval represents where the expression $x^2 - x - 12 \geq 0$ is true?
Which of the following rational function expressions are in lowest terms?
Which of the following rational function expressions are in lowest terms?
What is the range of the rational function $\frac{x + 2}{x + 1}$?
What is the range of the rational function $\frac{x + 2}{x + 1}$?
For the expression $-2x(x - 3)$, what are the critical points?
For the expression $-2x(x - 3)$, what are the critical points?
Flashcards are hidden until you start studying
Study Notes
Limits of Rational Functions
- As (x) approaches 1, the left limit of (f(x) = \frac{(x - 1)(x + 5)}{(x - 2)(x - 4)}) evaluates to 2, while the right limit also evaluates to 2.
- For (f(x) = \frac{(x - 1)(x + 2)}{(1 - 2)(1 - 4)}), the left limit approaches -∞ and the right limit approaches -∞.
- The left limit of (f(x) = \frac{-2(x + 3)(x + 1)}{(x - 1)^2}) approaches -∞, and the right limit also approaches -∞.
Identifying Holes and Asymptotes
- For (y = \frac{(x + 3)(x - 2)}{(x + 3)^2}), there is a hole at (x = -3) and a vertical asymptote at (x = -1).
- For (k(x) = \frac{(x + 7)(x + 2)}{(x + 1)(x + 2)}), identify no hole due to the absence of common factors in numerator and denominator.
- The vertical asymptote in (k(x)) is at (x = -1).
- (r(x) = \frac{x^2 - x}{x^2(x - 1)}) has a hole at (x = 1) and a vertical asymptote at (x = -1).
Solving Inequalities
- The inequality (x - 3 \leq 0) has solution ( (-\infty, 3] ).
- The inequality ((x - 1)(x + 2) \geq 0) has solution ([-2, 1)).
Limit Behavior of Graphs
- As input values decrease without bound for (f(x)), the limit approaches -∞.
- For (g(x)), as input values increase without bound, the limit approaches 1.
Analyzing Graphs
- Limit of (h(x)) approaches -∞ as (x) approaches values near the graph from the left and also tends to a finite value as input increases.
- The function (k(x)) has a limit approaching -2 as (x) approaches a certain value from the left.
Summary of Function Behavior
- (f(x)) and (g(x)) both stabilize at a limit of 1.
- Horizontal asymptotes and behavior at infinity show functions approaching fixed value limits across specified intervals.
- Continuous consideration of holes and vertical asymptotes is crucial for understanding graph behavior of rational functions.
Notable Functions and Their Limits
- (f(x) = \frac{2x^2 - 2x + 1}{3x^2 + 5x + 7}) indicated a left limit of 1.
- The graphs of (h(x)) and (k(x)) demonstrate distinct limits based on their structural forms and asymptotic behavior.
Analyzing Output Values
- There are descriptions connecting input values to output limits, emphasizing continuity and variability in rational function graphs.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.