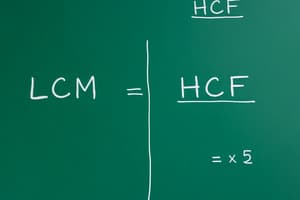Fractions, Roots, and Exponents: Exploring Mathematical Concepts

Fractions, Roots, and Exponents: A Deep Dive

In mathematics, fractions, roots, and exponents are connected concepts that play a crucial role in solving various mathematical problems. In this article, we will explore the fundamental concepts of fractions, roots, and exponents, along with some practical examples to help you understand these topics better.

Fractions

Definition and Components of Fractions

A fraction is a mathematical expression that represents a part of a whole. It consists of two main components: the numerator and the denominator. The numerator represents the number of parts taken from the whole, while the denominator represents the total number of equal parts in the whole. For example, in the fraction $\frac{3}{4}$, 3 is the numerator, and 4 is the denominator.

Types of Fractions

There are different types of fractions, including:

1. Proper Fractions: These are fractions where the numerator is smaller than the denominator, such as $\frac{1}{2}$ or $\frac{3}{4}$.

2. Improper Fractions: These are fractions where the numerator is greater than or equal to the denominator, such as $\frac{5}{4}$ or $1\frac{1}{2}$. An improper fraction can be converted into a mixed fraction by dividing the numerator by the denominator and expressing the result as a whole number and a proper fraction, e.g., $1\frac{1}{2} = 1 + \frac{1}{2}$.

3. Equivalent Fractions: These are fractions that have the same value but different numerators and denominators. For example, $\frac{3}{4}$ and $\frac{6}{8}$ are equivalent fractions, as they both represent the same part of the whole.

Fraction Operations

There are four basic fraction operations: addition, subtraction, multiplication, and division. These operations can be performed using the following rules:

• Addition: To add two fractions, the denominators must be the same. If they are not, find the least common multiple (LCM) of the denominators and convert each fraction to an equivalent fraction with the LCM as the denominator. Then, add the numerators and keep the resulting sum as the numerator of the new fraction.

• Subtraction: Subtract the numerator of the second fraction from the numerator of the first fraction. If the result is negative, change the sign of the denominator as well.

• Multiplication: Multiply the numerators and denominators separately. If the result of the multiplication of the numerators is greater than the LCM of the original denominators, reduce the resulting fraction to its simplest form.

• Division: Divide the numerator of the dividend fraction by the numerator of the divisor fraction, and divide the denominator of the dividend fraction by the denominator of the divisor fraction. If the result of the division of the numerators is less than the LCM of the original denominators, reduce the resulting fraction to its simplest form.

Roots

Definition and Types of Roots

A root of a number is a value that, when substituted for the variable in an equation, makes the equation true. There are two main types of roots:

1. Real Roots: These are the roots that can be expressed as real numbers, without the need of an imaginary part. For example, the root of $x^2 = 25$ is 5, which is a real number.

2. Complex Roots: These are the roots that cannot be expressed as real numbers and require the use of the imaginary unit $i$, which is the square root of -1. For example, the roots of $x^2 + 1 = 0$ are $i$ and $-i$.

Square Roots and Cube Roots

Square roots and cube roots are special types of roots that are used to find the value of a number when it is raised to a power of 1/2 or 1/3, respectively. For example, the square root of 25 is 5, and the cube root of 8 is 2.

Finding Roots of Numbers

To find the roots of a number, you can use the following methods:

• Factorization: Factor the number into its prime factors and then find the roots of each factor. For example, to find the square root of 81, factor 81 as $3^4$ and then find the square root of 3.

• Long Division: If the number is not a perfect power, you can use long division to find the root. For example, to find the square root of 625, divide 625 by 25.

Exponents

Definition and Rules of Exponents

An exponent is a mathematical operation that raises a base to a power, which is defined as the number of times the base is multiplied by itself. For example, $2^3$ means 2 multiplied by itself 3 times, which equals 8. The base is the number being raised to a power, and the exponent is the number of times the base is multiplied by itself.

There are several rules for exponents, including:

1. Product of Powers: $a^m \cdot a^n = a^{m+n}$

2. Quotient of Powers: $\frac{a^m}{a^n} = a^{m-n}$

3. Power of a Power: $(a^m)^n = a^{mn}$

4. Zero Exponent: $a^0 = 1$ for $a \neq 0$

5. Negative Exponent: $a^{-n} = \frac{1}{a^n}$

Exponential Functions

An exponential function is a function that models the relationship between a quantity and the time it takes for the quantity to grow or decay at a constant percentage rate. The general form of an exponential function is $f(x) = ab^x$, where $a$ is the initial value, $b$ is the growth or decay factor, and $x$ is the time.

Exponential Growth and Decay

Exponential growth occurs when the growth factor $b$ is greater than 1, meaning the quantity is growing at a constant percentage rate. Exponential decay occurs when the growth factor $b$ is between 0 and 1, meaning the quantity is decreasing at a constant percentage rate.

Solving Exponential Equations

To solve exponential equations, you can use the following methods:

• **Log

Explore the fundamental concepts of fractions, roots, and exponents in mathematics, along with practical examples and methods for solving various mathematical problems involving these concepts.