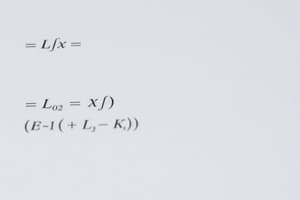Exploring Calculus: Limits, Derivatives, and Integrals

Study Notes

Exploring Math's Evolutionary Realm: Calculus

Calculus isn't just another math subject; it's a fascinating toolkit for understanding dynamics of change within our world. This field builds upon foundational mathematics by delving into concepts like limits, derivatives, integrals—conceiving how things change over time and space. In this exploratory journey through math's evolutionary realm, let's examine the intricacies of calculus.

Limits: Prelude to Continuous Change

Limits introduce us to the conceptual heartbeat of calculus—a means of describing continuous changes in quantities. A limit is the value a function approaches as its input gets closer and closer to a particular number. For instance, if (f(x)) becomes increasingly close to (L) as (x \to c,) we say that the limit of (f(x)) is (L).

Let me illustrate with a classic example. Consider the function (y = x^2.) As (x) nears zero from either side, (y) becomes smaller and smaller without reaching zero itself. We say the limit as (x) goes to zero equals zero: (\lim_{x \to 0} x^2 = 0).

Derivatives: Measuring Instantaneous Rates of Change

Derivatives represent the instantaneous rate of change of functions. Mathematically speaking, they define the slope of tangent lines to graphs of functions at specific points. To calculate a derivative, you'll often employ differentiation rules, such as power rule (((ax)^n=anx^{n-1})), product rule, quotient rule, chain rule, etc.. These techniques help solve complex problems involving rates of change.

For instance, suppose we want to find out how fast a car's velocity changes when traveling along a road described by the distance-time curve, (d(t)=9t+3), where (t) represents time measured in hours. To do so, we need to compute the first derivative of the distance function, which gives the speed: (v'(t) = d'(t) = 9\text{ mph}.) Thus, the car travels at a constant rate of 9 miles per hour.

Integrals: Summing Up Chunks of Information Over Intervals

Geometrically, integration corresponds to finding areas under curves. However, this powerful technique also helps us determine total amounts, accumulations, and averages. To compute integrals, mathematicians utilize various strategies—such as substitution, parts, and definite integral methods.

To exemplify, imagine estimating the total revenue generated during (1\text{ min}-2\text{ min}) by a business whose income follows a quadratic trend given by the equation (R(t) = t^2 + 1). By evaluating the definite integral [\int_1^2 (t^2+1) dt,] we can ascertain the company earned $3 square units of their chosen currency within one minute.

In closing, these fundamental tools enrich our mathematical knowledge and propel scientific discovery across diverse fields. Learning calculus expands your ability to explore relationships between variables and understand dynamic systems in ways previously unimaginable.

Description

Embark on a journey to unravel the intricacies of calculus by examining concepts like limits, derivatives, and integrals. Understand how calculus serves as a powerful toolkit to comprehend continuous changes, instantaneous rates of change, and summing up information over intervals.