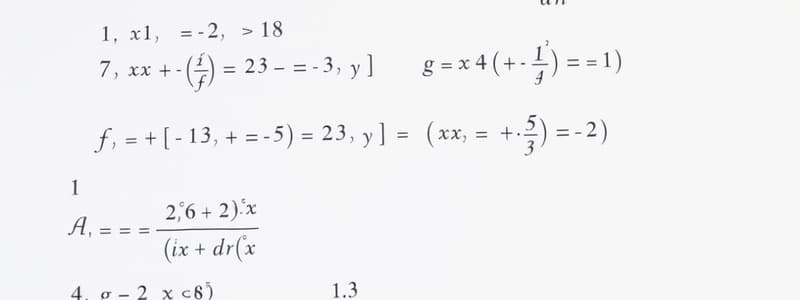Algebra 2 Functions and Relations Flashcards

Questions and Answers

What is the mapping diagram for the relation ((-2,-6), (0,3), (1,-5), (5,0))?

-2 -> -6, 0 -> 3, 1 -> -5, 5 -> 0

What is the domain and range of the relation (-1, 1/2), (-1/2, -1), (3/2, 0), (2, 3/2)?

domain: (-1, -1/2, 3/2, 2); range: (-1, 0, 1/2, 3/2)

Is the relation {(14, 15), (5, 7), (3, 10), (11, 1), (5, 8)} a function?

False

Use the vertical line test to determine which graph represents a function.

The graph with the singular horizontal line. Signup and view all the answers

For f(x) = 5x + 1, what is f(-4)?

-19 Signup and view all the answers

What function rule models the cost of concert tickets that are $25 each with a $1.75 handling fee? Evaluate the function for 6 tickets.

25t + 1.75; $151.75 Signup and view all the answers

Study Notes

Mapping Diagrams and Functions

A mapping diagram visually represents relationships by showing how each input corresponds to an output.
Example relation:
- (-2 maps to -6)
- (0 maps to 3)
- (1 maps to -5)
- (5 maps to 0)

Graphing Relations

Graph points:
- (-1, 1/2),
- (-1/2, -1),
- (3/2, 0),
- (2, 3/2)
Domain consists of all x-values:
- (-1, -1/2, 3/2, 2)
Range consists of all y-values:
- (-1, 0, 1/2, 3/2)

Identifying Functions

A relation is not a function if an input is associated with multiple outputs.
Example relation:
- {(14, 15), (5, 7), (3, 10), (11, 1), (5, 8)} is not a function because the input 5 corresponds to two outputs (7 and 8).

Vertical Line Test

A graph represents a function if any vertical line drawn intersects it at most once.
Identifying which graph passes this test is crucial for determining functions.

Function Evaluation

Evaluate linear functions by substituting values into the function:
- For f(x) = 5x + 1, substituting x = -4 yields f(-4) = -19.

Cost Functions

Functions can model real-world scenarios, like ticket pricing.
Example function for concert tickets includes a base price and a handling fee:
- Total cost = 25t + 1.75
- For 6 tickets, evaluate function: Total cost = $151.75.

Description

Test your understanding of functions and relations with these flashcards designed for Algebra 2. Each card presents a different problem related to mapping diagrams, graphs, and identifying functions. Prepare to enhance your algebra skills through engaging practice!