Write the equation of this line in slope-intercept form.
Understand the Problem
The question is asking to derive the equation of a line given in a graph and express it in slope-intercept form (y = mx + b). This involves determining the slope (m) and y-intercept (b) from the graph.
Answer
The equation of the line is $y = -\frac{1}{3}x - 13$.
Answer for screen readers
The equation of the line in slope-intercept form is:
$$ y = -\frac{1}{3}x - 13 $$
Steps to Solve
- Identify two points on the line
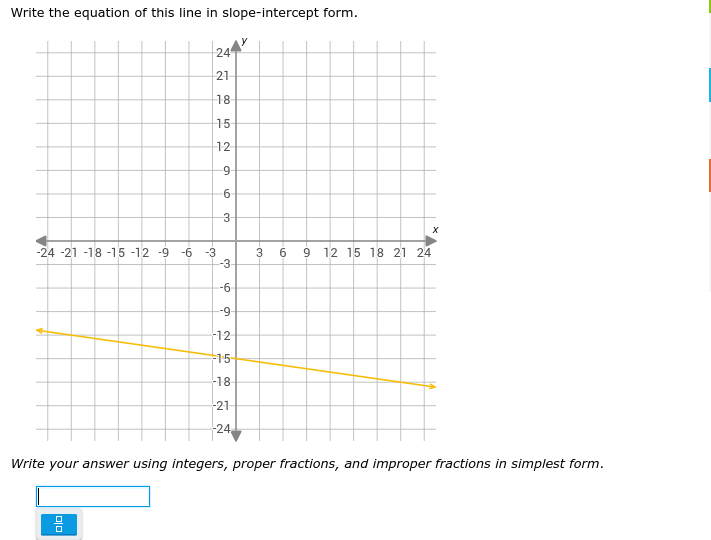
From the graph, we can identify two points that the line passes through. For example, we can select the points $(-3, -12)$ and $(6, -15)$.
- Calculate the slope (m)
The slope $m$ is calculated using the formula:
$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$
Substituting the points:
$$ m = \frac{-15 - (-12)}{6 - (-3)} = \frac{-15 + 12}{6 + 3} = \frac{-3}{9} = -\frac{1}{3} $$
- Determine the y-intercept (b)
To find the y-intercept, we can use one of the identified points along with the slope in the slope-intercept form $y = mx + b$. Using the point $(-3, -12)$:
$$ -12 = -\frac{1}{3}(-3) + b $$
This simplifies to:
$$ -12 = 1 + b $$
Solving for $b$ gives:
$$ b = -12 - 1 = -13 $$
- Write the equation in slope-intercept form
Now we can write the final equation of the line in slope-intercept form as:
$$ y = -\frac{1}{3}x - 13 $$
The equation of the line in slope-intercept form is:
$$ y = -\frac{1}{3}x - 13 $$
More Information
This equation represents a line that has a slope of $-\frac{1}{3}$, indicating that for every increase of 1 in $x$, $y$ decreases by $\frac{1}{3}$. The y-intercept of -13 indicates that the line crosses the y-axis at (0, -13).
Tips
- Forgetting to subtract values correctly when calculating the slope.
- Confusing the order of coordinates when using the slope formula, leading to calculation errors.
AI-generated content may contain errors. Please verify critical information