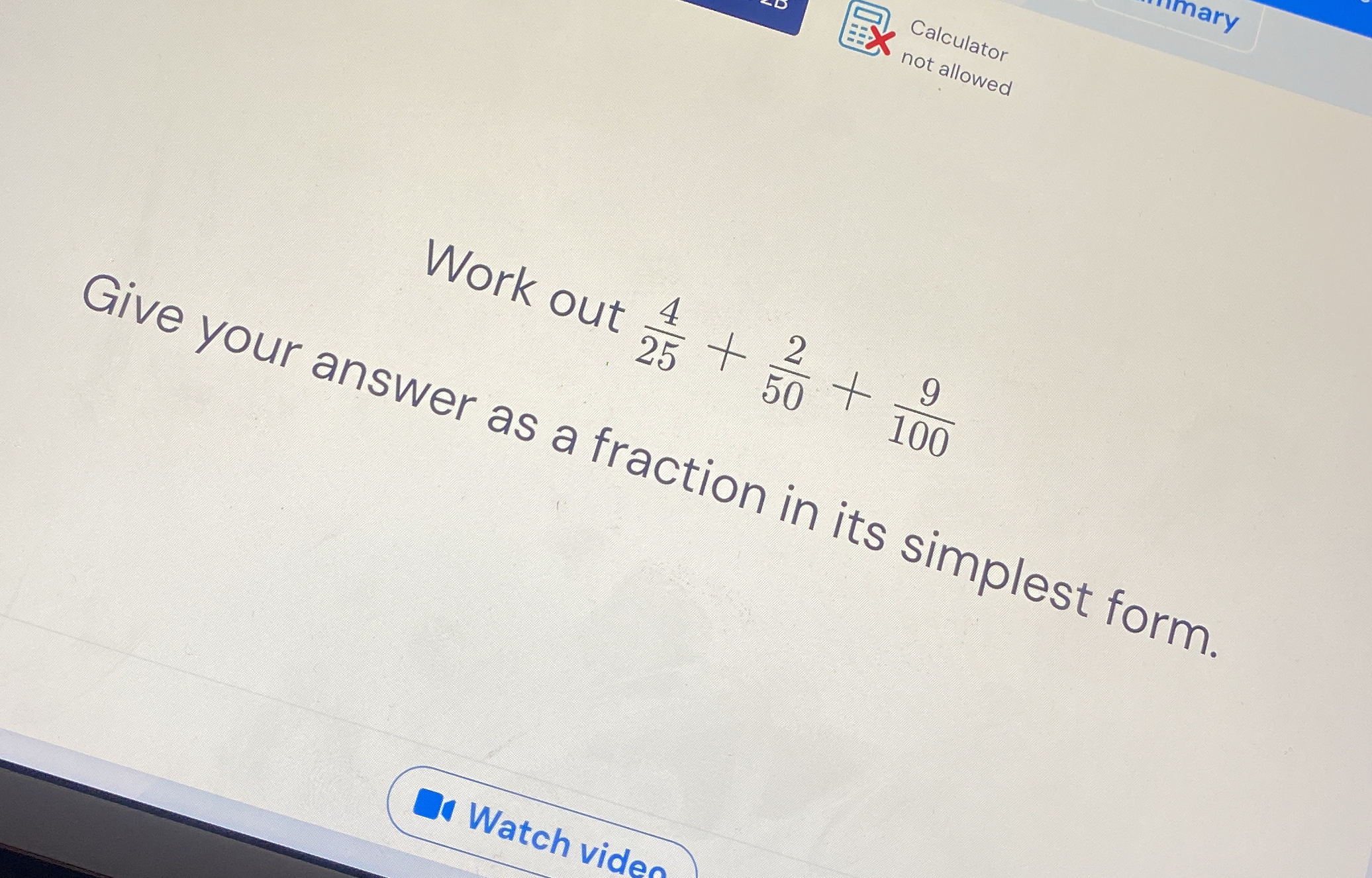
Work out 4/25 + 2/50 + 9/100. Give your answer as a fraction in its simplest form.
Understand the Problem
The question is asking to perform the addition of three fractions: 4/25, 2/50, and 9/100, and then simplify the result to its simplest form.
Answer
The sum of the fractions is $\frac{29}{100}$.
Answer for screen readers
The final answer is $\frac{29}{100}$.
Steps to Solve
- Find the least common denominator (LCD)
The denominators are 25, 50, and 100. The least common denominator is 100.
- Convert each fraction to the common denominator
-
Convert $\frac{4}{25}$: $$ \frac{4}{25} = \frac{4 \times 4}{25 \times 4} = \frac{16}{100} $$
-
Convert $\frac{2}{50}$: $$ \frac{2}{50} = \frac{2 \times 2}{50 \times 2} = \frac{4}{100} $$
-
The fraction $\frac{9}{100}$ is already at the common denominator.
- Add the converted fractions
Now we can add the fractions: $$ \frac{16}{100} + \frac{4}{100} + \frac{9}{100} = \frac{16 + 4 + 9}{100} = \frac{29}{100} $$
- Simplify the result
The fraction $\frac{29}{100}$ is already in its simplest form since 29 is a prime number.
The final answer is $\frac{29}{100}$.
More Information
The sum of the fractions results in $\frac{29}{100}$. This fraction cannot be simplified further, as the numerator and denominator share no common factors other than 1.
Tips
- Incorrect LCD: Often, students might select a common denominator that is not the least common multiple. Always double-check the denominators.
- Adding numerators incorrectly: Be careful to accurately add the numerators after converting to a common denominator.
AI-generated content may contain errors. Please verify critical information